Nhân dịp khai trương cửa hàng giảm giá cho 10% cho mặt hàng may mặc , 20% cho mặt hàng dụng cụ học tập.Bạn Phúc đi vào cửa hàng mua được 1 bộ đồ thể thao và 1 hộp bút.Biết giá niêm yết 1 bộ đồ thể thao là 280 000 đồng và giá 1 hộp bút là 80 000 đồng. Hỏi nếu bạn Phúc mang theo 400 000 đồng thì bạn còn dư bao nhiêu tiền?
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


`#3107.101107`
`a)`
`5 - (x - 6) = 4(2x - 3)`
\(\Leftrightarrow5-x+6=8x-12\)
\(\Leftrightarrow-x-8x=-12-5-6\)
\(\Leftrightarrow-9x=-23\)
\(\Leftrightarrow x=\dfrac{-23}{-9}=\dfrac{23}{9}\)
Vậy, \(x=\dfrac{23}{9}\)
`b)`
\(5\left(3x+2\right)-4\left(5-3x\right)=1\)
\(\Leftrightarrow15x+10-20+12x=1\)
\(\Leftrightarrow27x-10=1\)
\(\Leftrightarrow27x=11\)
\(\Leftrightarrow x=\dfrac{11}{27}\)
Vậy, \(x=\dfrac{11}{27}\)
`c)`
\(-4\left(x-3\right)=6x+\left(x-3\right)\)
\(\Leftrightarrow-4x+12=6x+x-3\)
\(\Leftrightarrow-4x-7x=-12-3\)
\(\Leftrightarrow11x=-15\)
\(\Leftrightarrow x=\dfrac{-15}{11}\)
Vậy, \(x=-\dfrac{15}{11}\)
`d)`
\(\dfrac{x}{3}-\dfrac{5x}{6}-\dfrac{5x}{12}=\dfrac{x}{4}-5\)
\(\Leftrightarrow\dfrac{4x}{12}-\dfrac{10x}{12}-\dfrac{5x}{12}=\dfrac{3x}{12}-\dfrac{60}{12}\)
\(\Leftrightarrow4x-10x-5x=3x-60\)
\(\Leftrightarrow-11x-3x=-60\)
\(\Leftrightarrow-14x=-60\)
\(\Leftrightarrow x=\dfrac{-60}{-14}=\dfrac{30}{7}\)
Vậy, \(x=\dfrac{30}{7}\)
`e)`
\(\dfrac{x-1}{2}-\dfrac{x+1}{15}-\dfrac{2x-13}{6}=0\)
\(\Leftrightarrow\dfrac{15\left(x-1\right)}{30}-\dfrac{2\left(x+1\right)}{30}-\dfrac{5\left(2x-13\right)}{30}=0\)
\(\Leftrightarrow15\left(x-1\right)-2\left(x+1\right)-5\left(2x-13\right)=0\)
\(\Leftrightarrow15x-15-2x-2-10x+65=0\)
\(\Leftrightarrow3x+48=0\)
\(\Leftrightarrow3x=-48\)
\(\Leftrightarrow x=-16\)
Vậy, \(x=-16.\)
`f)`
\(\dfrac{3\left(3-x\right)}{8}+\dfrac{2\left(5-x\right)}{3}=\dfrac{1-x}{2}-2\)
\(\Leftrightarrow\dfrac{9\left(3-x\right)}{24}+\dfrac{16\left(5-x\right)}{24}=\dfrac{12\left(1-x\right)}{24}-\dfrac{48}{24}\)
\(\Leftrightarrow27-9x+80-16x=12-12x-48\)
\(\Leftrightarrow-9x-16x+12x=12-48-27-80\)
\(\Leftrightarrow-13x=-143\)
\(\Leftrightarrow x=11\)
Vậy, `x = 11.`
`g)`
\(\dfrac{3\left(5x-2\right)}{4}-2=\dfrac{7x}{3}-5\left(x-7\right)\)
\(\Leftrightarrow\dfrac{9\left(5x-2\right)}{12}-\dfrac{24}{12}=\dfrac{28x}{12}-\dfrac{60\left(x-7\right)}{12}\)
\(\Leftrightarrow45x-18-24=28x-60x+420\)
\(\Leftrightarrow45x-28x+60x=42+420\)
\(\Leftrightarrow77x=462\)
\(\Leftrightarrow x=6\)
Vậy, `x = 6`
`h)`
\(\dfrac{x+5}{2}+\dfrac{3-2x}{4}=x-\dfrac{7+x}{6}\)
\(\Leftrightarrow\dfrac{6\left(x+5\right)}{12}+\dfrac{3\left(3-2x\right)}{12}=\dfrac{12x}{12}-\dfrac{2\left(7+x\right)}{12}\)
\(\Leftrightarrow6x+30+9-6x=12x-14-2x\)
\(\Leftrightarrow-10x=-53\)
\(\Leftrightarrow x=5,3\)
Vậy, `x = 5,3`
`i)`
\(\dfrac{x-3}{11}+\dfrac{x+1}{3}=\dfrac{x+7}{9}-1\)
\(\Leftrightarrow\dfrac{9\left(x-3\right)}{99}+\dfrac{33\left(x+1\right)}{99}=\dfrac{11\left(x+7\right)}{99}-\dfrac{99}{99}\)
\(\Leftrightarrow9x-27+33x+33=11x+77-99\)
\(\Leftrightarrow42x-11x=-22-6\)
\(\Leftrightarrow31x=-28\)
\(\Leftrightarrow x=-\dfrac{28}{31}\)
Vậy, \(x=-\dfrac{28}{31}.\)

a: 5+2x=20-3x
=>2x+3x=20-5
=>3x=15
=>\(x=\dfrac{15}{3}=5\)
b: \(7-\left(2x+4\right)=-\left(x+4\right)\)
=>\(7-2x-4+x+4=0\)
=>7-x=0
=>x=7
c: \(\left(5x+2\right)-3\left(2x+1\right)=-3x+7\)
=>\(5x+2-6x-3=-3x+7\)
=>-3x+7=-x-1
=>-2x=-8
=>x=4
d: \(2x-\left(3-5x\right)=4\left(x+3\right)\)
=>2x-3+5x=4x+12
=>7x-3=4x+12
=>3x=15
=>\(x=\dfrac{15}{3}=5\)
e: \(5\left(2x-3\right)-4\left(5x-7\right)=19-2\left(x+11\right)\)
=>\(10x-15-20x+28=19-2x-22\)
=>\(-10x+13=-2x-3\)
=>-8x=-16
=>x=2
f: \(x^2-\left(x+2\right)\left(x-2\right)=2x\)
=>\(x^2-\left(x^2-4\right)=2x\)
=>\(2x=x^2-x^2+4=4\)
=>\(x=\dfrac{4}{2}=2\)
g: \(\dfrac{1-3x}{6}+x-1=\dfrac{x+2}{2}\)
=>\(\dfrac{1-3x+6\left(x-1\right)}{6}=\dfrac{3x+6}{6}\)
=>1-3x+6x-6=3x+6
=>3x-5=3x+6
=>-5=6(loại)
h: \(\dfrac{3\left(2x+1\right)}{4}-5-\dfrac{3x+2}{10}=\dfrac{2\left(3x-1\right)}{5}\)
=>\(\dfrac{15\left(2x+1\right)-100-2\left(3x+2\right)}{20}=\dfrac{8\left(3x-1\right)}{20}\)
=>30x+15-100-6x-4=24x-8
=>24x-89=24x-8
=>-89=-8(vô lý)

a: 2x+6=0
=>2x=-6
=>x=-3
b: 4x+20=0
=>4x=-20
=>x=-5
c: 2(x+1)=5x-7
=>5x-7=2x+2
=>3x=9
=>x=3
d: \(2x-3=0\)
=>2x=3
=>\(x=\dfrac{3}{2}\)
e: 3x-1=x+3
=>3x-x=3+1
=>2x=4
=>\(x=\dfrac{4}{2}=2\)
f: 15-7x=9-3x
=>-7x+3x=9-15
=>-4x=-6
=>x=1,5
g: x-3=18
=>x=18+3=21
h: 2x+1=15-5x
=>2x+5x=15-1
=>7x=14
=>\(x=\dfrac{14}{7}=2\)
i: 3x-2=2x+5
=>3x-2x=2+5
=>x=7
k: -4x+8=0
=>-4x=-8
=>\(x=\dfrac{-8}{-4}=2\)
l: 2x+3=0
=>2x=-3
=>\(x=-\dfrac{3}{2}\)
m: 4x+5=3x
=>4x-3x=-5
=>x=-5

a: |x-4|=1
=>\(\left[{}\begin{matrix}x-4=1\\x-4=-1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=5\left(nhận\right)\\x=3\left(loại\right)\end{matrix}\right.\)
Khi x=5 thì \(B=\dfrac{5+1}{5-3}=\dfrac{6}{2}=3\)
b: \(A=\dfrac{x}{x-3}-\dfrac{x+1}{x+3}+\dfrac{3x-3}{9-x^2}\)
\(=\dfrac{x}{x-3}-\dfrac{x+1}{x+3}-\dfrac{3x-3}{\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{x\left(x+3\right)-\left(x+1\right)\left(x-3\right)-3x+3}{\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{x^2+3x-\left(x^2-2x-3\right)-3x+3}{\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{x^2+3-x^2+2x+3}{\left(x-3\right)\left(x+3\right)}=\dfrac{2x+6}{\left(x-3\right)\left(x+3\right)}=\dfrac{2}{x-3}\)
c: \(M=B:A=\dfrac{2}{x-3}:\dfrac{x}{x-3}=\dfrac{2}{x}\)
Để M=5 thì 2/x=5
=>\(x=\dfrac{2}{5}\left(nhận\right)\)
d: \(N=B-A=\dfrac{2}{x-3}-\dfrac{x}{x-3}=\dfrac{2-x}{x-3}\)
Để N là số nguyên thì \(2-x⋮x-3\)
=>\(x-2⋮x-3\)
=>\(x-3+1⋮x-3\)
=>\(1⋮x-3\)
=>\(x-3\in\left\{1;-1\right\}\)
=>\(x\in\left\{4;2\right\}\)

Bài 19:
Gọi số sản phẩm tổ 1 làm được trong tháng đầu là x(sản phẩm)
(Điều kiện: \(x\in Z^+\))
Số sản phẩm tổ 2 làm được trong tháng đầu là:
800-x(sản phẩm)
Số sản phẩm tổ 1 làm đươc trong tháng hai là:
\(\left(1+15\%\right)\cdot x=1,15x\left(sảnphẩm\right)\)
Số sản phẩm tổ 2 làm được trong tháng hai là:
\(\left(1+20\%\right)\left(800-x\right)=1,2\left(800-x\right)\left(sảnphẩm\right)\)
Tổng số sản phẩm hai tổ làm được trong tháng hai là 945 sản phẩm nên ta có:
1,15x+1,2(800-x)=945
=>1,15x+960-1,2x=945
=>-0,05x=-15
=>x=300(nhận)
Vậy: Trong tháng đầu, tổ 1 làm được 300 sản phẩm, tổ 2 làm được 800-300=500 sản phẩm

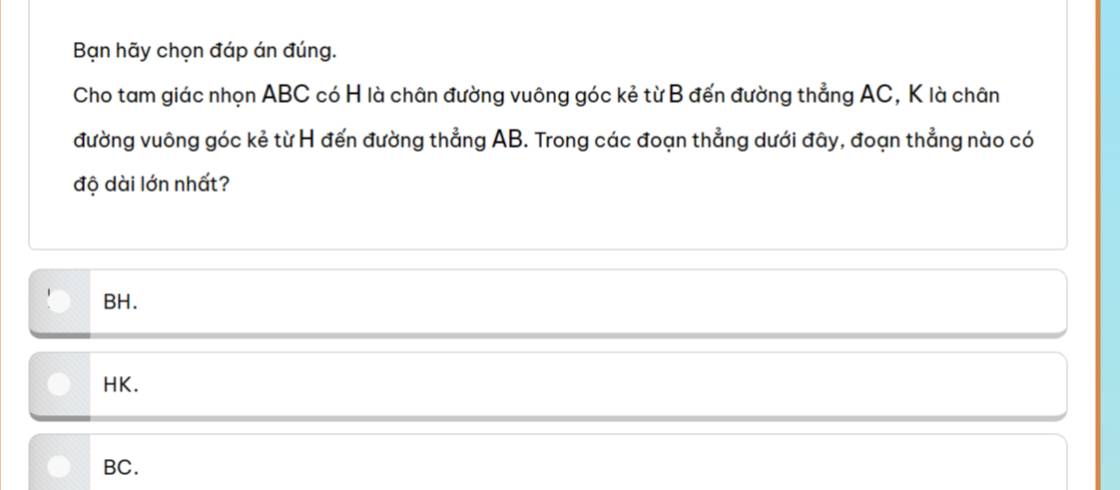
a: PA là đường cao ứng với đỉnh P của ΔMNP
=>PA\(\perp\)MN
b: Xét ΔMIN vuông tại I và ΔMAP vuông tại A có
\(\widehat{IMN}\) chung
Do đó: ΔMIN~ΔMAP
c: Xét ΔPIN vuông tại I và ΔPKM vuông tại K có
\(\widehat{IPN}\) chung
Do đó: ΔPIN~ΔPKM
=>\(\dfrac{PI}{PK}=\dfrac{PN}{PM}\)
=>\(\dfrac{PI}{PN}=\dfrac{PK}{PM}\)
Xét ΔPIK và ΔPNM có
\(\dfrac{PI}{PN}=\dfrac{PK}{PM}\)
\(\widehat{IPK}\) chung
Do đó: ΔPIK~ΔPNM
=>\(\widehat{PIK}=\widehat{PNM}\)
d: Xét ΔMIH vuông tại I và ΔMKP vuông tại K có
\(\widehat{IMH}\) chung
Do đó: ΔMIH~ΔMKP
=>\(\dfrac{MI}{MK}=\dfrac{MH}{MP}\)
=>\(MI\cdot MP=MH\cdot MK\)
e: \(\dfrac{PI}{PN}=\dfrac{PK}{PM}\)
=>\(PI\cdot PM=PK\cdot PN\)
\(MH\cdot MK+PK\cdot PN=PI\cdot PM+MI\cdot MP\)
\(=MP\left(PI+MI\right)=MP^2\)

\(\text{Δ}=\left[-2\left(m-1\right)\right]^2-4\cdot1\cdot\left(m^2-3\right)\)
\(=\left(2m-2\right)^2-4\left(m^2-3\right)\)
\(=4m^2-8m+4-4m^2+12=-8m+16\)
Để phương trình có hai nghiệm thì Δ>=0
=>-8m+16>=0
=>-8m>=-16
=>m<=2
Theo Vi-et, ta có:
\(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=2\left(m-1\right)\\x_1x_2=\dfrac{c}{a}=m^2-3\end{matrix}\right.\)
\(x_1^2+2\left(m-1\right)x_2< =3m^2+8\)
=>\(x_1^2+x_2\left(x_1+x_2\right)< =3m^2+8\)
=>\(\left(x_1^2+x_2^2\right)+x_1x_2< =3m^2+8\)
=>\(\left(x_1+x_2\right)^2-x_1x_2< =3m^2+8\)
=>\(\left(2m-2\right)^2-\left(m^2-3\right)-3m^2-8< =0\)
=>\(4m^2-8m+4-m^2+3-3m^2-8< =0\)
=>-8m-1<=0
=>8m+1>=0
=>\(m>=-\dfrac{1}{8}\)
=>\(-\dfrac{1}{8}< =m< =2\)
x2 - 2(m-1)x + m2 -3 = 0 (1)
(1) có 2 nghiệm khi Δ = [ -2(m-1)]2 - 4 . 1. (m2 -3) ≥ 0
<=> 4m2 - 8m + 4 - 4m2 +12 ≥ 0
<=> -8m + 16 ≥ 0
<=> m ≤ 2
Theo định lý Vi-ét:
S= x1 + x2 = -b/2.a = m -1
P= x1.x2 = c/a = m2 -3
Ta có : x1 là nghiệm của (1) nên
x12 - 2(m-1) x1 + m2 -3 = 0
<=> x12 = -2(m-1) x1 - m2 + 3
Từ đó:
x12 - 2(m-1) x2 ≤ 3m2 + 8
<=> -2(m-1) x1 - m2 + 3 - 2(m-1) x2 ≤ 3m2 + 8
<=> - 2(m-1)(x1 + x2) - 4m2 -5 ≤ 0
<=> -2(m2 - 2m +1) - 4m2 -5 ≤ 0
<=> -6m2 + 4m -7 ≤ 0 (đúng với mọi m ϵ R)
Vậy m ≤ 2 thì thỏa

a; \(\dfrac{x}{8}\) = \(\dfrac{y}{-5}\) và \(x\) + y = 15
Áp dụng tính chất dãy tỉ số bằng nhau ta có:
\(\dfrac{x}{8}\) = \(\dfrac{y}{-5}\) = \(\dfrac{x+y}{8-5}\) = \(\dfrac{15}{3}\) = 5
\(x\) = 5.8 = 40
y = 5.(-5)
y = - 25
Vậy (\(x;y\)) = ( 40; - 25)
b; Giải:
Một máy in hết số bao bì trong: 6 x 4 = 24 (giờ)
Thực tế số máy in số bao bì là: 6 - 2 = 4 (máy)
Nếu bị hỏng 4 máy thì sẽ in xong số bao bì trong:
24 : 4 = 6 (giờ)
Kết luận nếu bị hỏng hai máy thì xưởng in sẽ in hết số bao bì trong 6 giờ.


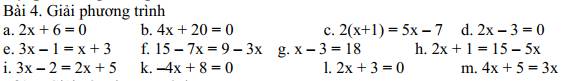




 help me, please~
help me, please~
10% của 1 bộ đồ thể thao là:
280 000 : 100 x 10 = 28 000 (đồng)
20% của 1 hộp bút là:
80 000 : 100 x 20 = 16 000 (đồng)
Tổng số tiền cần trả để mua 1 bộ đồ thể thao và 1 hộp bút khi chưa giảm giá là:
280 000 + 80 000 = 360 000 (đồng)
Khi đã giảm giá, bạn Phúc cần trả:
360 000 - 28 000 - 16 000 = 316 000 (đồng)
Nếu mang theo 400 000 đồng, bạn Phúc còn dư:
400 000 - 316 000 = 84 000 (đồng)
Đáp số: 84 000 đồng