`#040911`

`a)`

`(2x - 1)^2 - (2x + 5)(2x + 1) = 10`

`\Leftrightarrow 4x^2 - 4x + 1 - (4x^2 + 12x + 5) = 10`

`\Leftrightarrow 4x^2 - 4x + 1 - 4x^2 - 12x - 5 = 10`

`\Leftrightarrow (4x^2 - 4x^2) - (4x + 12x) + (1 - 5) = 10`

`\Leftrightarrow -16x - 4 = 10`

`\Leftrightarrow -16x = 10 + 4`

`\Leftrightarrow -16x = 14`

`\Leftrightarrow x = \dfrac{-7}{8}`

Vậy, `x= \dfrac{-7}{8}`

`b)`

`9^2(x - 1) + 25(1 - x) = 0`

`\Leftrightarrow 9^2(x - 1) - 25(x - 1) = 0`

`\Leftrightarrow (x - 1)(9^2 - 25) = 0`

`\Leftrightarrow`\(\left[{}\begin{matrix}x-1=0\\9^2-5^2=0\end{matrix}\right.\)

`\Leftrightarrow`\(\left[{}\begin{matrix}x=1\\\left(9-5\right)\left(9+5\right)=0\end{matrix}\right.\)

\(\Leftrightarrow\left[{}\begin{matrix}x=1\\4\cdot14=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=1\\56=0\left(\text{vô lý}\right)\end{matrix}\right.\\ \text{Vậy, x = 1}\)

`c)`

\(x^2+3x-4=0\)

`\Leftrightarrow x^2 + 4x - x - 4 = 0`

`\Leftrightarrow (x^2 - x) + (4x - 4) = 0`

`\Leftrightarrow x(x - 1) + 4(x - 1) = 0`

`\Leftrightarrow (x + 4)(x - 1) = 0`

\(\Leftrightarrow\left[{}\begin{matrix}x+4=0\\x-1=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=-4\\x=1\end{matrix}\right.\\ \text{ Vậy, }x\in\left(-4;1\right)\)

  2^x + 2^{x + 1} = 96

  2^x + 2^x . 2 = 96

 2^x . (1 + 2) = 96

         2^x . 3 = 96

              2^x = 96 : 3

              2^x = 32

              2^x = 2⁵

                x = 5

2 - \(x\) + \(\dfrac{3}{4}\) = \(\dfrac{55}{63}\) : \(\dfrac{11}{21}\)

2 - \(x\) + \(\dfrac{3}{4}\) = \(\dfrac{55}{63}\) x \(\dfrac{21}{11}\)

2 - \(x\) + \(\dfrac{3}{4}\) = \(\dfrac{5}{3}\)

       2 - \(x\) =  \(\dfrac{5}{3}\) - \(\dfrac{3}{4}\)

       2 - \(x\) = \(\dfrac{20}{12}\) - \(\dfrac{9}{12}\)

       2 - \(x\) = \(\dfrac{11}{12}\)

            \(x\) = 2 - \(\dfrac{11}{12}\)

            \(x\) = \(\dfrac{24}{12}\) - \(\dfrac{11}{12}\)

            x = \(\dfrac{13}{12}\)

\(1,6x-18y\\ =6\left(x-3y\right)\\ 2,-4xy^2+12x^2y\\ =-4xy\left(y-3x\right)\\ 3,5x^2y^3-25x^3y^4+10x^3y^3\\ =5x^2y^3\left(1-5xy+2x\right)\)

\(4,12x^2y-18x^2y^2-36xy^3\\ =6xy\left(2x-3xy-6y^2\right)\\ 5,10x^2\left(2x+1\right)-5x\left(2x+1\right)\\ =5x\left(2x+1\right)\left(2x-1\right)\\ 6,-28x^2\left(2x-y\right)-7x\left(y-2x\right)\\ =28x^2\left(y-2x\right)-7x\left(y-2x\right)\\ =7x\left(y-2x\right)\left(4x-1\right)\)

đáp số a rr

b

Em cần làm gì với hai biểu thức này vậy?

`#040911`

\(2^{10}\div8^5\\ =2^{10} \div\left(2^3\right)^5\\=2^{10}\div2^{15}=2^{-5} \)

Đề có nhầm gì k bạn?

5e6 kn;eorthi[05j[wpojt[e-rryytjdrtrdjdjyjjhhmhghcgcghghcghcghcghmgghghhyjfmfthfdmjsgfggmnfmghgmjghghcmhfdjtyjtytytyjtgfj65jhvc5frmng;fj;pbo/sfj'pjod'pbf/;dwkg

[vDJ[h0ourwj[0gur[0wigjerwipiu0[i[erkerjop;jwo[jeio[hiphjltjro;yh;t[jrperetee65e65e5etjtrjtehre4tr4eregs4e5eyret

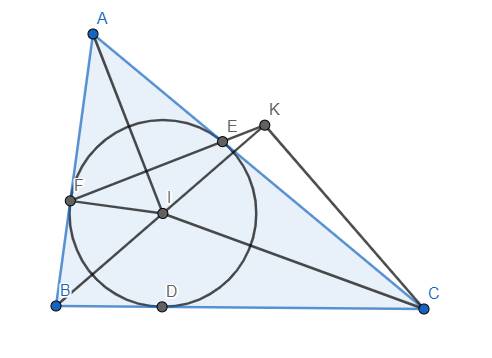
a) Ta dễ chứng minh \(\widehat{BIC}=90^o+\dfrac{\widehat{A}}{2}\). 

Ta thấy \(\widehat{BFK}=\widehat{A}+\widehat{AEF}=\dfrac{\widehat{A}}{2}+\widehat{IAE}+\widehat{AEF}\)  \(=90^o+\dfrac{\widehat{A}}{2}\)

Nên \(\widehat{BIC}=\widehat{BFK}\)

Xét 2 tam giác BIC và BFK, ta có: 

\(\widehat{FBK}=\widehat{IBC}\) (do BI là tia phân giác của \(\widehat{FBC}\)) và \(\widehat{BIC}=\widehat{BFK}\left(cmt\right)\) 

\(\Rightarrow\Delta BIC~\Delta BFK\left(g.g\right)\) (đpcm)

b) Từ \(\Delta BIC~\Delta BFK\Rightarrow\dfrac{BI}{BF}=\dfrac{BC}{BK}\) \(\Rightarrow\dfrac{BI}{BC}=\dfrac{BF}{BK}\)

Xét 2 tam giác BIF và BCK, ta có

\(\dfrac{BI}{BC}=\dfrac{BF}{BK}\) và \(\widehat{IBF}=\widehat{CBK}\)

\(\Rightarrow\Delta BIF~\Delta BCK\left(c.g.c\right)\)

\(\Rightarrow\widehat{BKC}=\widehat{BFI}\)

Mà \(\widehat{BFI}=90^o\) nên \(\widehat{BKC}=90^o\) (đpcm)

ai làm giúp phần a với, mãi ko ra:(((