Chứng minh rằng:
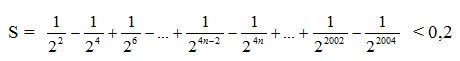
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


2\(xy\) + 6 + \(x\) + 3 = 2024
2\(xy\) + \(x\) + 9 = 2024
2\(xy\) + \(x\) = 2024 - 9
2\(xy\) + \(x\) = 2015
\(x\).(2y + 1) = 2015
\(x\) = 2015 : (2y + 1)
\(x\in\) Z ⇒ 2y + 1 \(\in\) Ư(2015) = { 1; 5; 13; 65; 31;155; 403;2015}
Lập bảng ta có:
| 2y + 1 | 1 | 5 | 13 | 65 | 31 | 155 | 403 | 2015 |
| y | 0 | 2 | 6 | 32 | 15 | 77 | 201 | 1007 |
| \(x\) | 2015 | 403 | 155 | 31 | 65 | 13 | 5 | 1 |
| (\(x\); y) | (2015;0) | (403;2) | (155;6) | (31;32) | (65;15) | (13;77) | (5;201) | (1;1007) |


Số lít nước đã đổ vào bể:
30 . 45 = 1350 (l) = 1,35 (m³)
Chiều cao mực nước:
1,35 : 1,2 : 1,5 = 0,75 (m)
Mặt nước còn cách miệng bể:
0,9 - 0,75 = 0,15 (m) = 15 (cm)

\(A=0,6+\left|\dfrac{1}{2}-x\right|\\ Vì:\left|\dfrac{1}{2}-x\right|\ge\forall0x\in R\\ Nên:A=0,6+\left|\dfrac{1}{2}-x\right|\ge0,6\forall x\in R\\ Vậy:min_A=0,6\Leftrightarrow\left(\dfrac{1}{2}-x\right)=0\Leftrightarrow x=\dfrac{1}{2}\)
\(B=\dfrac{2}{3}-\left|2x+\dfrac{2}{3}\right|\\ Vì:\left|2x+\dfrac{2}{3}\right|\ge0\forall x\in R\\ Nên:B=\dfrac{2}{3}-\left|2x+\dfrac{2}{3}\right|\le\dfrac{2}{3}\forall x\in R\\ Vậy:max_B=\dfrac{2}{3}\Leftrightarrow\left|2x+\dfrac{2}{3}\right|=0\Leftrightarrow x=-\dfrac{1}{3}\)

a) |2,5 - x| = 1,3
*) Với x ≤ 2,5, ta có:
2,5 - x = 1,3
x = 2,5 - 1,3
x = 1,2 (nhận)
*) Với x > 2,5, ta có:
2,5 - x = -1,3
x = 2,5 + 1,3
x = 3,8 (nhận)
Vậy x = 1,2; x = 3,8
b) 1,6 - |x - 0,2| = 0
|x - 0,2| = 1,6
*) Với x ≥ 0,2, ta có:
x - 0,2 = 1,6
x = 1,6 + 02
x = 1,8 (nhận)
*) Với x < 0,2, ta có:
x - 0,2 = -1,6
x = -1,6 + 0,2
x = -1,4
Vậy x = -1,4, x = 1,8
Lời giải:
$S=\frac{1}{2^2}-\frac{1}{2^4}+\frac{1}{2^6}-....+\frac{1}{2^{2002}}-\frac{1}{2^{2004}}$
$2^2S=1-\frac{1}{2^2}+\frac{1}{2^4}-....+\frac{1}{2^{2000}}-\frac{1}{2^{2002}}$
$\Rightarrow S+2^2S=1-\frac{1}{2^{2004}}<1$
$\Rightarrow 5S< 1$
$\Rightarrow S< \frac{1}{5}$
Hay $S<0,2$