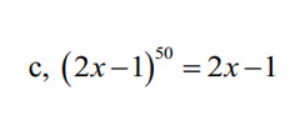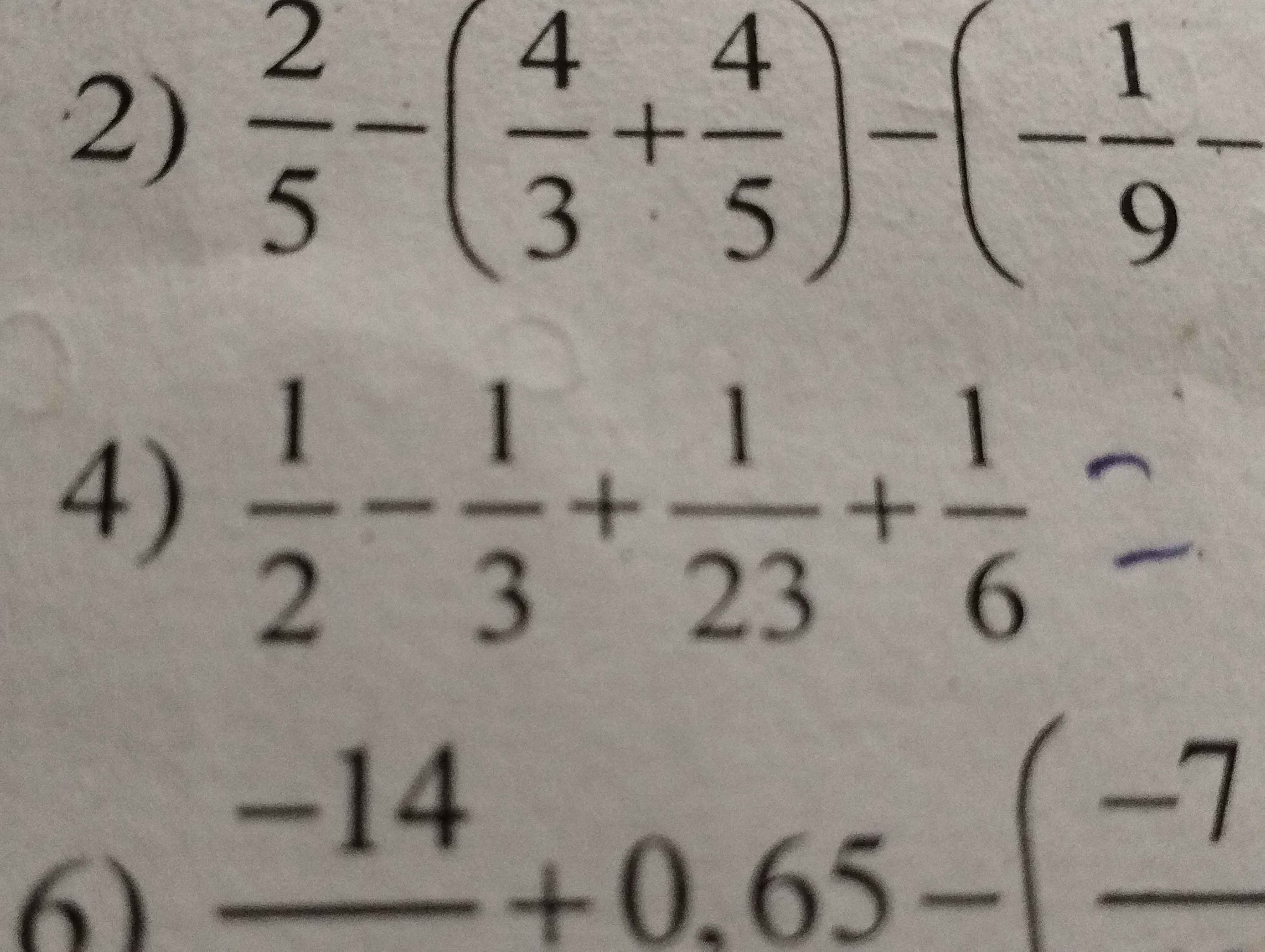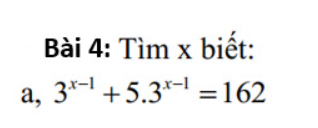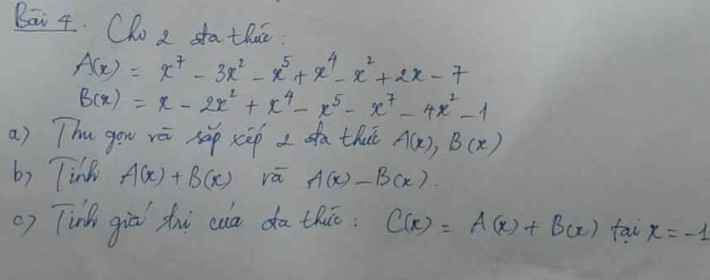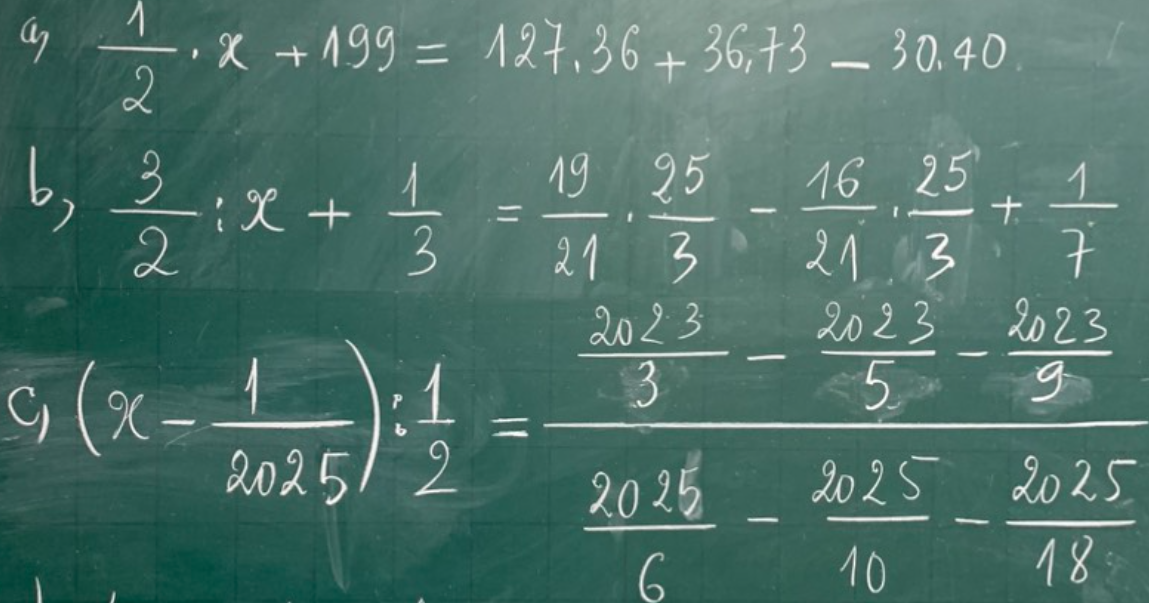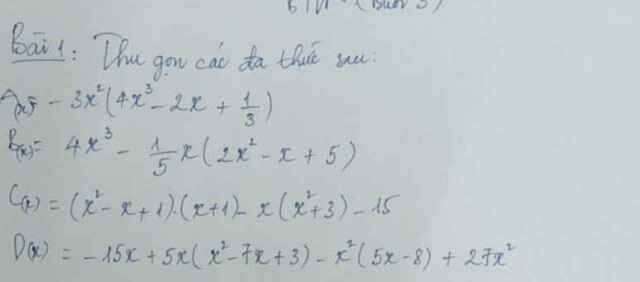\(\left(2x-1\right)^{50}=2x-1\\ =>\left(2x-1\right)^{50}-\left(2x-1\right)=0\\ =>\left(2x-1\right)\left[\left(2x-1\right)^{49}-1\right]=0\)

TH1: 

\(2x-1\\ =>2x=1\\ =>x=\dfrac{1}{2}\)

TH2:

\(\left(2x-1\right)^{49}-1=0\\=>\left(2x-1\right)^{49}=1\\ =>\left(2x-1\right)^{49}=1^{49}\\ =>2x-1=1\\ =>2x=1+1=2\\ =>x=\dfrac{2}{2}\\ =>x=1\)

\(\dfrac{1}{2}-\dfrac{1}{3}+\dfrac{1}{23}+\dfrac{1}{6}\\ =\left(\dfrac{1}{2}-\dfrac{1}{3}\right)+\dfrac{1}{23}+\dfrac{1}{6}\\ =\dfrac{1}{6}+\dfrac{1}{23}+\dfrac{1}{6}\\ =\dfrac{2}{6}+\dfrac{1}{23}\\ =\dfrac{1}{3}+\dfrac{1}{23}\\ =\dfrac{26}{69}\)

\(3^{x-1}+5\cdot3^{x-1}=162\\ =>3^{x-1}\cdot\left(1+5\right)=162\\ =>3^{x-1}\cdot6=162\\ =>3^{x-1}=162:6\\ =>3^{x-1}=27\\ =>3^{x-1}=3^3\\ =>x-1=3\\ =>x=1+3=4\)

a; 8 : \(x\) = 2

         \(x\)  = 8 : 2

         \(x\) = 4; \(x\) \(\in\) N*

⇒ \(x\) \(\in\) A = {1; 2; 3; 4}

Tập A có 4 phần tử

b;    \(x\) + 3 < 5 ⇒ \(x\) < 5 - 3 ⇒ \(x< 2\) vì \(x\in\) N 

  ⇒ \(x\) \(\in\) B = {0; 1} Vậy tập B có 2 phần tử

C;  \(x\) - 2 = \(x+2\)

     \(x\) - \(x\) = 2 + 2

        0   =  4 (vô lí)

   C = \(\varnothing\)

Số phần tử của tập C là 0 phần tử

d;    \(x:2=x:4\)

      \(\dfrac{x}{2}\) - \(\dfrac{x}{4}\) = 0

      \(x\) x (\(\dfrac{1}{2}\) - \(\dfrac{1}{4}\)) = 0

        \(x\times\) \(\dfrac{1}{4}\) = 0

        \(x=0\)

D = {0}

Tập D có 1 phần tử

e;    \(x\) + 0 = \(x\) 

        \(x\) = \(x\)

   Vậy E = {0; 1; 2; 3; 4;...}

Tập E có vô số phần tử

\(a)A\left(x\right)=x^7-3x^2-x^5+x^4-x^2+2x-7\\ =x^7-x^5+x^4-\left(3x^2+x^2\right)+2x-7\\ =x^7-x^5+x^4-4x^2+2x-7\\ B\left(x\right)=x-2x^2+x^4-x^5-x^7-4x^2-1\\ =-x^7-x^5+x^4-\left(2x^2+4x^2\right)+x-1\\ =-x^7-x^5+x^4-6x^2+x-1\) 

\(b)A\left(x\right)+B\left(x\right)\\ =\left(x^7-x^5+x^4-4x^2+2x-7\right)+\left(-x^7-x^5+x^4-6x^2+x-1\right)\\ =\left(x^7-x^7\right)-\left(x^5+x^5\right)+\left(x^4+x^4\right)-\left(4x^2+6x^2\right)+\left(2x+x\right)-\left(7+1\right)\\ =-x^5+2x^4-10x^2+3x-8\) 

\(A\left(x\right)-B\left(x\right)=\left(x^7-x^5+x^4-4x^2+2x-7\right)-\left(-x^7-x^5+x^4-6x^2+x-1\right)\\ =\left(x^7+x^7\right)+\left(x^5-x^5\right)+\left(x^4-x^4\right)-\left(4x^2-6x^2\right)+\left(2x-x\right)-\left(7-1\right)\\ =2x^7+2x^2+x-6\)

c) Thay x = -1  vào C(x) = A(x) + B(x) ta có:

\(C\left(x\right)=-\left(-1\right)^5+2\cdot\left(-1\right)^4-10\cdot\left(-1\right)^2+3\cdot\left(-1\right)-8\\ =-\left(-1\right)+2\cdot1-10\cdot1-3\cdot1-8\\ =1+2-10-3-8\\ =-18\)

\(A\left(x\right)=3x^2\left(4x^3-2x+\dfrac{1}{3}\right)\\ =3x^2\cdot4x^3-3x^2\cdot2x+3x^2\cdot\dfrac{1}{3}\\ =12x^5-6x^3+x^2\\ B\left(x\right)=4x^3-\dfrac{1}{5}x\left(2x^2-x+5\right)\\ =4x^3-\dfrac{1}{5}x\cdot2x^2+\dfrac{1}{5}x\cdot x-\dfrac{1}{5}x\cdot5\\ =4x^3-\dfrac{2}{5}x^3+\dfrac{1}{5}x^2-x\\ =\dfrac{18}{5}x^3+\dfrac{1}{2}x^5-x\\ C\left(x\right)=\left(x^2-x+1\right)\left(x+1\right)-x\left(x^2+3\right)-15\\ =x^3+1-x^3-3x-15\\ =\left(x^3-x^3\right)-3x+\left(1-15\right)\\ =-3x-14\\ D\left(x\right)=-15x+5x\left(x^2-7x+3\right)-x^2\left(5x-8\right)+27x^2\\ =-15x+5x^3-35x^2+15x-5x^3+8x^2+27x^2\\ =\left(-15x+15x\right)+\left(5x^3-5x^3\right)+\left(8x^2-35x^2+27x^2\right)\\ =0\)