Cho tam giác ABC vuông tại A. Kẻ AH vuông tại BC, Từ H kẻ HE vuông tại AB, HF vuông tại AC. Gọi O là giao điểm của AH và EF. Kẻ AM là đường trung tuyến (M là trung điểm của BC). Chứng minh AM vuông EF
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
S
1
PD
2
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
16 tháng 8 2023
1, \(x^2\) - \(x\) + \(\dfrac{1}{4}\) = 0
\(x^2\) - 2.\(x\).\(\dfrac{1}{2}\) + \(\dfrac{1}{4}\) = 0
(\(x\) - \(\dfrac{1}{2}\))2 = 0
\(x\) - \(\dfrac{1}{2}\) =0
\(x\) = \(\dfrac{1}{2}\)
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
16 tháng 8 2023
2, \(x^2\) - 10\(x\) = -25
\(x^2\) - 10\(x\) + 25 = 0
(\(x\) - 5)2 = 0
\(x\) - 5 =0
\(x\) = 5
YH
1
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
16 tháng 8 2023
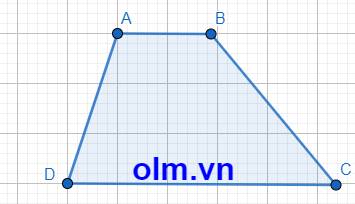
Vì AB//CD nên Góc A và góc D là hai góc trong cùng phía
\(\widehat{A}\)+ \(\widehat{D}\) = 1800 ⇒ \(\widehat{D}\) + 3\(\widehat{D}\) = 1800 ⇒ \(\widehat{D}\) = 1800:4 = 450
\(\widehat{A}\) = 450.3 =1350
\(\widehat{B}\)+\(\widehat{C}\) = 1800 ⇒ \(\widehat{B}\) + \(\widehat{B}\) - 300 = 1800 ⇒2\(\widehat{B}\) =2100 ⇒ \(\widehat{B}\) = 1050
\(\widehat{C}\) = 1050 - 300 = 750
HG
0
VT
1
16 tháng 8 2023
Diện tích hình bình hành ABCD
\(S_{ABCD}=AC.AD=4.3=12\left(cm^2\right)\)
NH
1
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
16 tháng 8 2023
(2\(x\) + 1)3
= (2\(x\))3 + 3.(2\(x\))2 + 3.2\(x\).12 + 13
= 8\(x^3\) + 12\(x^2\) + 6\(x\) + 1
ND
16 tháng 8 2023
Mày ra câu hỏi từ từ người ta trả lới cho chứ cứ hối người ta 😡
16 tháng 8 2023
\(D=-x^2-y^2+xy+2x+2y\)
\(\Rightarrow D=-\dfrac{x^2}{2}+xy-\dfrac{y^2}{2}-\dfrac{x^2}{2}+2x-\dfrac{y^2}{2}+2y\)
\(\Rightarrow D=-\left(\dfrac{x^2}{2}-xy+\dfrac{y^2}{2}\right)-\left(\dfrac{x^2}{2}-2x\right)-\left(\dfrac{y^2}{2}-2y\right)\)
\(\Rightarrow D=-\left(\dfrac{x^2}{2}-2.\dfrac{x}{\sqrt[]{2}}.\dfrac{y}{\sqrt[]{2}}+\dfrac{y^2}{2}\right)-\left(\dfrac{x^2}{2}-2.\dfrac{x}{\sqrt[]{2}}.\sqrt[]{2}+2\right)-\left(\dfrac{y^2}{2}-2.\dfrac{y}{\sqrt[]{2}}.\sqrt[]{2}+2\right)+2+2\)
\(\Rightarrow D=-\left(\dfrac{x}{\sqrt[]{2}}-\dfrac{y}{\sqrt[]{2}}\right)^2-\left(\dfrac{x}{\sqrt[]{2}}-\sqrt[]{2}\right)^2-\left(\dfrac{y}{\sqrt[]{2}}-\sqrt[]{2}\right)^2+4\)
mà \(\left\{{}\begin{matrix}-\left(\dfrac{x}{\sqrt[]{2}}-\dfrac{y}{\sqrt[]{2}}\right)^2\le0,\forall x;y\\-\left(\dfrac{x}{\sqrt[]{2}}-\sqrt[]{2}\right)^2\le0,\forall x\\-\left(\dfrac{y}{\sqrt[]{2}}-\sqrt[]{2}\right)^2\le0,\forall y\end{matrix}\right.\)
\(\Rightarrow D=-\left(\dfrac{x}{\sqrt[]{2}}-\dfrac{y}{\sqrt[]{2}}\right)^2-\left(\dfrac{x}{\sqrt[]{2}}-\sqrt[]{2}\right)^2-\left(\dfrac{y}{\sqrt[]{2}}-\sqrt[]{2}\right)^2+4\le4\)
\(\Rightarrow GTLN\left(D\right)=4\left(tạix=y=2\right)\)