1\

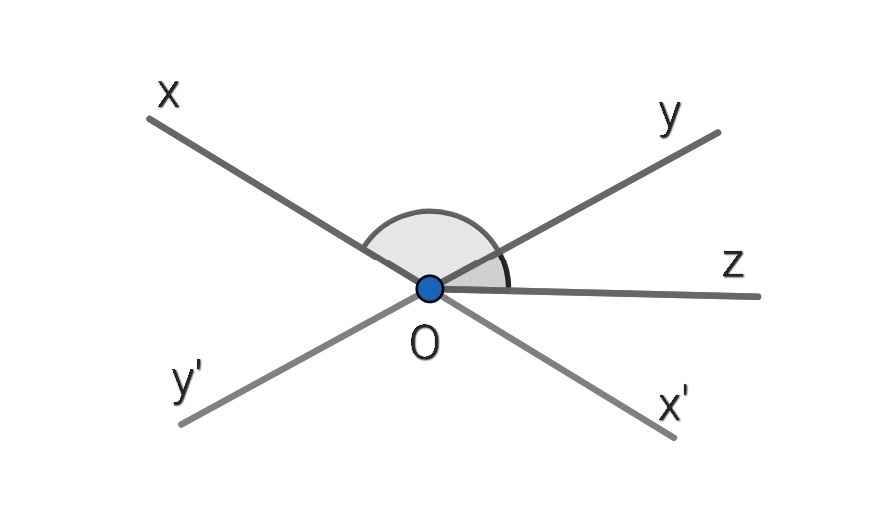 a) Ta có:

∠xOy + ∠yOz = 150⁰

∠xOy - ∠yOz = 90⁰

⇒ ∠xOy = (150⁰ + 90⁰) : 2 = 120⁰

⇒ ∠yOz = 120⁰ - 90⁰ = 30⁰

b) Ta có:

∠xOy + ∠x'Oy = 180⁰ (kề bù)

⇒ ∠x'Oy = 180⁰ - ∠xOy

= 180⁰ - 120⁰

= 60⁰

xoy=(150+90):2=120

yoz=120-90=30

√0,81.(√x - 16/25) = 9/10

0,9.(√x - 16/25) = 9/10

√x - 16/25 = 9/10 : 0,9

√x - 16/25 = 1

√x = 1 + 16/25

√x = 41/25

x = 1681/625 (nhận)

Vậy x = 1681/625

\(\sqrt{0,81}\left(\sqrt{x}-\dfrac{16}{25}\right)=\dfrac{9}{10}\left(x\ge0\right)\\ \Leftrightarrow\sqrt{\dfrac{81}{100}}.\left(\sqrt{x}-\dfrac{16}{25}\right)=\dfrac{9}{10}\\ \Leftrightarrow\dfrac{\sqrt{81}}{\sqrt{100}}.\left(\sqrt{x}-\dfrac{16}{25}\right)=\dfrac{9}{10}\\ \Leftrightarrow\dfrac{9}{10}.\left(\sqrt{x}-\dfrac{16}{25}\right)=\dfrac{9}{10}\\ \Leftrightarrow\sqrt{x}-\dfrac{16}{25}=1\\ \Leftrightarrow\sqrt{x}=\dfrac{41}{25}\\ \Leftrightarrow x=\dfrac{1681}{625}\)

Vậy x = \(\dfrac{1681}{625}\)

M H K P Q I O

a/ Xét tg MKP và tg MHQ có

\(MP=MH\left(gt\right);MK=MQ\left(gt\right)\) (1)

\(\widehat{KMP}=\widehat{HMP}+\widehat{HMK}=90^o+\widehat{HMK}\)

\(\widehat{HMQ}=\widehat{KMQ}+\widehat{HMK}=90^o+\widehat{HMK}\)

\(\Rightarrow\widehat{KMP}=\widehat{HMQ}\) (2)

Từ (1) và (2) => tg MKP = tg MHQ => PK=HQ

b/

Xét tg vuông HMP có

\(\widehat{MPH}+\widehat{MHP}=90^o\)

\(\Rightarrow\widehat{MPK}+\widehat{HPK}+\widehat{MHP}=90^o\)

Ta có

tg MKP = tg MHQ (cmt) \(\Rightarrow\widehat{MPK}=\widehat{MHQ}\)

\(\Rightarrow\widehat{MHQ}+\widehat{HPK}+\widehat{MHP}=90^o\)

\(\Rightarrow\left(\widehat{MHQ}+\widehat{MHP}\right)+\widehat{HPK}=90^o\)

\(\Rightarrow\widehat{PHQ}+\widehat{HPK}=90^o\)

Xét tg HPI có

\(\widehat{PHQ}+\widehat{HPK}=90^o\left(cmt\right)\Rightarrow\widehat{HIP}=90^o\Rightarrow PK\perp HQ\)

c/

∆ABC có:

∠A + ∠ABC + ∠C = 180⁰ (tổng ba góc trong ∆ABC)

⇒ ∠ABC = 180⁰ - (∠A + ∠C)

= 180⁰ - (90⁰ + 40⁰)

= 180⁰ - 130⁰

= 50⁰

⇒ ∠B₁ = ∠B₂ = 50⁰ : 2 = 25⁰

∆ABD có:

∠A + ∠B₁ + ∠ADB = 180⁰ (tổng ba góc trong ∆ABD)

⇒ x = ∠ADB = 180⁰ - (∠A + ∠B₁)

= 180⁰ - (90⁰ + 25⁰)

= 65⁰

⇒ ∠BDE = 90⁰ - ∠ADB

= 90⁰ - 65⁰

= 25⁰

∆BDE có:

∠B₂ + ∠BDE + ∠BED = 180⁰ (tổng ba góc trong ∆BDE)

⇒ y = ∠BED = 180⁰ - (∠B₂ + ∠BDE)

= 180⁰ - (25⁰ + 25⁰)

= 130⁰

mn ơi giup mik vs

gấp lắm r mn ơii

A = \(\dfrac{\dfrac{2022}{1}+\dfrac{2021}{2}+\dfrac{2020}{3}+...+\dfrac{1}{2022}}{\dfrac{1}{2}+\dfrac{1}{3}+\dfrac{1}{4}+...+\dfrac{1}{2023}}\)

Xét TS = \(\dfrac{2022}{1}\) + \(\dfrac{2021}{2}\) \(\dfrac{2020}{3}\) +... + \(\dfrac{1}{2022}\)

      TS = (1 + \(\dfrac{2021}{2}\)) + (1 + \(\dfrac{2020}{3}\)) + ... + ( 1 + \(\dfrac{1}{2022}\)) + 1 

      TS = \(\dfrac{2023}{2}\) + \(\dfrac{2023}{3}\) +...+ \(\dfrac{2023}{2022}\) + \(\dfrac{2023}{2023}\)

      TS =  2023.(\(\dfrac{1}{2}\) + \(\dfrac{1}{3}\) + \(\dfrac{1}{4}\) +...+ \(\dfrac{1}{2023}\))

A = \(\dfrac{2023.\left(\dfrac{1}{2}+\dfrac{1}{3}+\dfrac{1}{4}+...+\dfrac{1}{2023}\right)}{\left(\dfrac{1}{2}+\dfrac{1}{3}+\dfrac{1}{4}+...+\dfrac{1}{2023}\right)}\)

 A = 2023

Em cảm ơn ạ

Lời giải:
a. Thể tích cái bánh: $6\times 8\times 3:2=72$ (cm³)

b.

Độ dài cạnh chéo miếng bánh: $\sqrt{6^2+8^2}=10$ (cm)

Diện tích vật liệu cần dùng chính là diện tích toàn phần của cái bánh và bằng:

$6.8+6.3+3.8+10.3=120$ (cm²)