\(B=\frac{1}{1\cdot 5}+\frac{1}{5\cdot 9}+\dots +\frac{1}{\left(4n-3\right)\cdot \left(4n+1\right)}\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



Phân tích bài văn "Đề Mèn Phiêu Lưu Ký"
"Đề Mèn Phiêu Lưu Ký" là một tác phẩm nổi bật của nhà văn Tô Hoài, được xuất bản lần đầu vào năm 1941. Bài văn này kể về cuộc phiêu lưu của chú Mèn, một con chuột sống trong một khu làng. Qua câu chuyện, tác giả đã gửi gắm những thông điệp về sự trưởng thành, cái nhìn sâu sắc về xã hội, sự gan dạ và lòng dũng cảm, cùng với cái nhìn chân thực về cuộc sống của loài vật.
1. Tóm tắt nội dung chính: Chú Mèn là một con chuột trẻ con, lần đầu tiên được đi xa khỏi nhà, ra ngoài thế giới để khám phá. Chuyến phiêu lưu của Mèn có thể được coi là hành trình trưởng thành, giúp Mèn học được nhiều bài học quý giá về cuộc sống, từ việc đối mặt với hiểm nguy, hiểu rõ giá trị của sự tự do, đến việc nhận thức về bản thân và xã hội.
Trong hành trình đó, Mèn gặp nhiều loài vật khác nhau, mỗi loài đều có đặc điểm và vai trò riêng. Chú trải qua nhiều tình huống, thách thức, mạo hiểm, từ đó thấy được sự khốc liệt của cuộc sống và nhận ra rằng, đôi khi, con người và những loài vật cũng chỉ là một phần trong một chuỗi lớn hơn của tự nhiên.
2. Phân tích nhân vật Mèn: Mèn là một nhân vật có tính cách phức tạp, có thể vừa đáng yêu, vừa ngây thơ, vừa thông minh. Ban đầu, Mèn là một con chuột còn non nớt, chỉ biết chạy theo cảm xúc và sự tò mò. Tuy nhiên, qua các tình huống, Mèn đã dần trưởng thành. Mèn không chỉ thể hiện sự can đảm khi đối mặt với hiểm nguy mà còn cho thấy sự khôn ngoan khi học hỏi từ những trải nghiệm.
Trong suốt hành trình, Mèn đã học được sự khắc nghiệt của cuộc sống, không chỉ từ các mối đe dọa mà còn từ những bài học về tình bạn, tình yêu thương và sự dũng cảm.
3. Ý nghĩa của cuộc phiêu lưu: Cuộc phiêu lưu của Mèn không chỉ là một hành trình vật lý mà còn là hành trình nội tâm của chính nhân vật. Từ một chú chuột ngây thơ, Mèn đã dần nhận ra những giá trị của cuộc sống, từ đó hiểu rõ hơn về bản thân, về những điều xung quanh mình. Điều này phản ánh một thông điệp sâu sắc về sự trưởng thành của mỗi cá nhân qua quá trình trải nghiệm.
4. Ý nghĩa nhân văn trong tác phẩm: Tác phẩm mang một ý nghĩa nhân văn sâu sắc, phản ánh những triết lý về cuộc sống, về sự đối mặt với thử thách và những thay đổi mà mỗi người phải đối mặt trong suốt cuộc đời. "Đề Mèn Phiêu Lưu Ký" cũng đề cập đến những vấn đề xã hội như sự phân biệt giai cấp, lòng dũng cảm và khát vọng tự do.
Qua việc miêu tả cuộc phiêu lưu của Mèn, Tô Hoài không chỉ muốn thể hiện cuộc sống của loài vật mà còn ngầm phản ánh đời sống con người, từ những khó khăn, thử thách đến việc nhận thức rõ hơn về vai trò của mình trong xã hội.
5. Ngôn ngữ và phong cách viết: Ngôn ngữ trong tác phẩm của Tô Hoài rất dễ hiểu, gần gũi và đặc biệt giàu tính hình ảnh. Qua cách miêu tả sinh động, tác giả không chỉ xây dựng được các nhân vật loài vật mà còn khắc họa rõ nét bức tranh về thiên nhiên và xã hội, tạo ra một không gian sống động và hấp dẫn cho người đọc.
Kết luận: Tác phẩm "Đề Mèn Phiêu Lưu Ký" không chỉ là câu chuyện về một chú chuột mà còn là một bài học quý giá về cuộc sống, về sự trưởng thành, về cách đối diện với những thử thách. Bằng những tình huống đầy ý nghĩa và sự quan sát tinh tế, Tô Hoài đã xây dựng một câu chuyện sâu sắc, có giá trị nhân văn cao.

\(-\dfrac{15x^2y^3}{18x^3y^5}=-\dfrac{15}{18}\cdot\dfrac{x^2}{x^3}\cdot\dfrac{y^3}{y^5}=\dfrac{-5}{6\cdot x\cdot y^2}\)

Trường em cũng như rất nhiều các trường học khác thường tổ chức các buổi lao động tập trung hoặc theo lớp để dọn vệ sinh, trồng cây xanh… góp phần xây dựng trường học xanh, sạch, đẹp. Như thường lệ, thứ bảy tuần vừa rồi một buổi lao động đã diễn ra với sự tham gia nhiệt tình của các bạn học sinh.
Đó là một buổi lao động tập trung theo trường và các lớp lao động theo sự phân công của nhà trường. Mỗi lớp một việc, lớp thì quét sân trường, lớp thì lau bàn ghế và cửa kính… Riêng lớp em được phân công tưới chăm sóc các bồn hoa. Cuối buổi học của ngày thứ sáu bạn lớp trưởng đã phân công các bạn mang dụng cụ cho buổi lao động, bạn thì mang xô để xách nước, bạn thì mang bao tải để đựng cỏ…
Đúng tám giờ sáng thứ bảy toàn trường tập trung đầy đủ mang theo dụng cụ đã được phân công. Các bạn tập trung nghe theo thầy giáo phổ biến kế hoạch của buổi lao động một lúc rồi chia ra theo các lớp, bạn lớp trưởng điểm danh rồi chúng em bắt đầu vào công việc.
Các bạn chia ra thành các nhóm nhỏ, rồi mỗi nhóm nhận trách nhiệm một bồn hoa, có bao nhiêu bồn hoa thì chia ra bấy nhiêu nhóm. Mỗi bạn một tay, nhổ sạch cỏ trong các bồn hoa, sau đó lấy xô xách nước để tưới. Mặc dù trời rất nắng và nóng song các bạn làm việc rất nhiệt tình, các lớp khác cũng vậy ai cũng chăm chỉ làm tốt công việc được phân công.
Khi công việc đã làm được khá nhiều, ai nấy đều đã thấm mệt, chúng em tập trung lại và cử một số bạn đi mua nước và một ít hoa quả để giải lao, hầu như lớp nào cũng vậy, Chúng em ăn uống rất vui vẻ, những cốc nước mát như xóa tan đi cái mệt. Các lớp còn mời nhau lại uống nước và ăn hoa quả cùng nhóm của mình. Sau thời gian nghỉ ngơi, ai nấy đều như tỉnh táo hẳn và sẵn sàng cho số công việc còn lại của lớp mình. Mọi người rất tích cực để nhanh chóng hoàn thành công việc. Một số lớp đã làm xong thì vui vẻ ra hỗ trợ các lớp chưa làm xong để mọi người cùng được nghỉ. Tất cả đều vui vẻ giúp đỡ chứ không tỏ ra khó chịu khi phải làm hộ một phần cho các lớp khác.
Đúng mười một giờ, tất cả các lớp đã hoàn thành xong công việc được giao. Các bạn trong mỗi lớp tập trung lại để lớp trưởng đi báo cáo với các thầy cô. Sau khi báo cáo xong, các bạn ra về khi đã có sự cho phép của các thầy cô.
Buổi lao động được đánh giá là rất sôi nổi và có hiệu quả không những góp phần làm đẹp thêm cho trường học mà còn thể hiện sự đoàn kết giữa các lớp với nhau khi gặp khó khăn.

Cho dung dịch potassium hydroxide (KOH) thì quỳ tím sẽ thành xanh bn nhé.

Bài 2:
a: Khi x=4 thì \(M=\dfrac{4+3}{4-2}=\dfrac{7}{2}\)
b: \(M=\dfrac{2}{3}\)
=>\(\dfrac{x+3}{x-2}=\dfrac{2}{3}\)
=>3(x+3)=2(x-2)
=>3x+9=2x-4
=>3x-2x=-4-9
=>x=-13(nhận)
c: Để M là số nguyên dương thì \(\left\{{}\begin{matrix}x+3⋮x-2\\M>0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x-2+5⋮x-2\\\dfrac{x+3}{x-2}>0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}5⋮x-2\\\left[{}\begin{matrix}x>2\\x< -3\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x-2\in\left\{1;-1;5;-5\right\}\\\left[{}\begin{matrix}x>2\\x< -3\end{matrix}\right.\end{matrix}\right.\)
=>\(x\in\left\{3;7\right\}\)
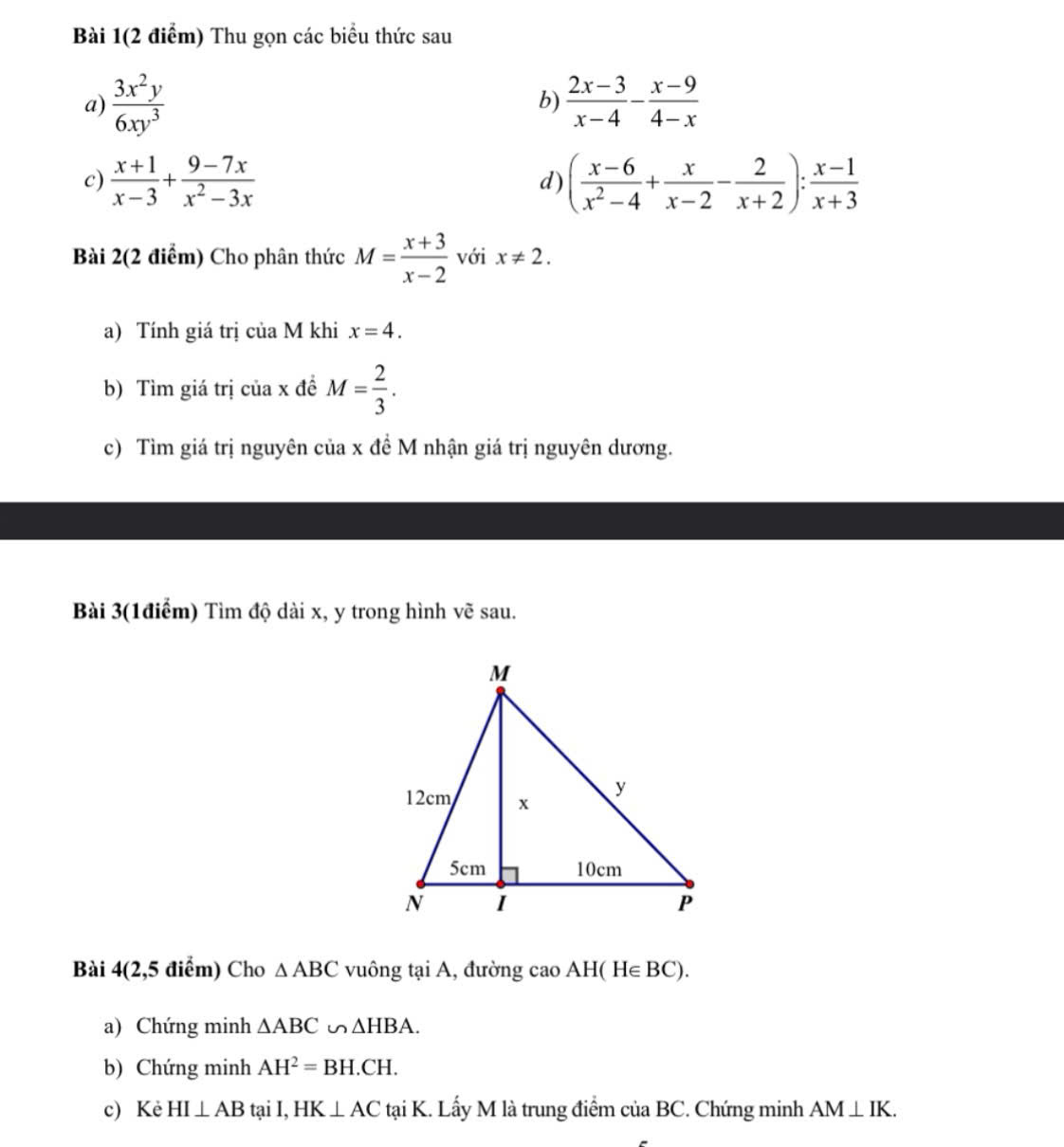
Bài 3:
ΔMIN vuông tại I
=>\(IM^2+IN^2=MN^2\)
=>\(x=MI=\sqrt{12^2-5^2}=\sqrt{144-25}=\sqrt{119}\left(cm\right)\)
ΔMIP vuông tại I
=>\(IM^2+IP^2=PM^2\)
=>\(y=\sqrt{119+100}=\sqrt{219}\left(cm\right)\)
Bài 4:
a: Xét ΔBAC vuông tại A và ΔBHA vuông tại H có
\(\widehat{ABC}\) chung
Do đó: ΔBAC~ΔBHA
b: Xét ΔHBA vuông tại H và ΔHAC vuông tại H có
\(\widehat{HBA}=\widehat{HAC}\left(=90^0-\widehat{HAB}\right)\)
Do đó: ΔHBA~ΔHAC
=>\(\dfrac{HB}{HA}=\dfrac{HA}{HC}\)
=>\(HA^2=HB\cdot HC\)
c: Xét tứ giác AIHK có \(\widehat{AIH}=\widehat{AKH}=\widehat{KAI}=90^0\)
nên AIHK là hình chữ nhật
=>\(\widehat{AKI}=\widehat{AHI}\)
mà \(\widehat{AHI}=\widehat{ABC}\left(=90^0-\widehat{HAB}\right)\)
nên \(\widehat{AKI}=\widehat{ABC}\)
ΔABC vuông tại A
mà AM là đường trung tuyến
nên MA=MC
=>ΔMAC cân tại M
=>\(\widehat{MAC}=\widehat{MCA}\)
\(\widehat{AKI}+\widehat{MAC}=\widehat{ABC}+\widehat{ACB}=90^0\)
=>AM\(\perp\)IK
B =\(\frac{1}{1.5}\) + \(\frac{1}{5.9}\) + ...+ \(\frac{1}{\left(4n-3\right).\left(4n+1\right)}\)
B = \(\frac14\).(\(\frac{4}{1.5}+\frac{4}{5.9}+\cdots+\frac{4}{\left(4n-3\right).\left(4n+1\right)}\)
B = \(\frac14\).(\(\frac11\) - \(\frac15\) + \(\frac15\) - \(\frac19\) + ... + \(\frac{1}{4n-3}-\frac{1}{4n+1}\))
B = \(\frac14\).(\(\frac11\) - \(\frac{1}{4n+1}\))
B = \(\frac14\).\(\frac{4n}{4n+1}\)
B = \(\frac{n}{4n+1}\)