tìm tất cả các giá trị của tham số để hàm số 2 y=mx^2-(m^2+1)x+3 đồng biến trên (1;dương vô cùng)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


đt△ x + 4y - 2 = 0 => y = -\(\dfrac{1}{4}\)x + \(\dfrac{1}{2}\)
Đt d có dạng y = ax + b vì (d) //Δ nên a = -\(\dfrac{1}{4}\); b # \(\dfrac{1}{2}\)
đt (d) có dạng y = \(-\dfrac{1}{4}\) x + b ⇒x+ 4y - 4b = 0
Khoảng cách từ A(-2;3) đến đường thẳng (d) là :
d(A;d) = \(\dfrac{|-2+4.3-4b|}{\sqrt{1^2+4^2}}\) = 3
| 10 - 4b| = 3\(\sqrt{17}\)
10- 4b = 3\(\sqrt{17}\)
b = \(\dfrac{10-3\sqrt{17}}{4}\)
4b - 10 = 3\(\sqrt{17}\)
b = \(\dfrac{10+3\sqrt{17}}{4}\)
pt đt d thỏa mãn đề bài là:
y = - \(\dfrac{1}{4}\) x + \(\dfrac{10-3\sqrt{17}}{4}\) hoặc y = \(-\dfrac{1}{4}\) x + \(\dfrac{10+3\sqrt{17}}{4}\)

a) A(3;-5) ; B(1;0)
=> \(\overrightarrow{AB}\left(-2;5\right)\)
Gọi C(x;y) tọa độ cần tìm
khi đó \(\overrightarrow{OC}\left(x;y\right)\)
\(\overrightarrow{OC}=-3\overrightarrow{AB}\Leftrightarrow\left\{{}\begin{matrix}x=-3.\left(-2\right)=6\\y=-3.5=-15\end{matrix}\right.\)
Vậy C(6;-15)
b) D đối xứng với A qua C
=> C trung điểm AD
Gọi D(x1;y1)
Ta có : \(6=\dfrac{3+x_1}{2}\Leftrightarrow x_1=9\)
\(-15=\dfrac{-5+y_1}{2}\) <=> y1 = -25
Vậy D(9;-25)

ĐKXĐ : \(\left\{{}\begin{matrix}2x^2+5\ge0\\x^2-x+11\ge0\end{matrix}\right.\Leftrightarrow\forall x\inℝ\)
\(\sqrt{2x^2+5}=\sqrt{x^2-x+11}\)
<=> 2x2 + 5 = x2 - x + 11
<=> x2 + x - 6 = 0
<=> (x - 2)(x + 3) = 0
<=> \(\left[{}\begin{matrix}x=2\\x=-3\end{matrix}\right.\)
Tập nghiệm phương trình S = {2;-3}

Theo đề bài, giá bán \(x\) sản phẩm là \(170x\) (nghìn đồng)
Để nhà sản xuất không bị lỗ thì \(P\left(x\right)\le170x\) \(\Leftrightarrow x^2+30x+3300\le170x\) \(\Leftrightarrow x^2-140x+3300\le0\) \(\Leftrightarrow\left(x-110\right)\left(x-30\right)\le0\)
Đặt \(f\left(x\right)=\left(x-110\right)\left(x-30\right)\). Ta lập bảng xét dấu:
| \(x\) | \(-\infty\) \(30\) \(110\) \(+\infty\) |
| \(f\left(x\right)\) | \(+\) \(0\) \(-\) \(0\) \(+\) |
Vậy \(f\left(x\right)\le0\Leftrightarrow x\in\left[30;110\right]\). Do đó, để nhà sản xuất không bị lỗ thì số sản phẩm được sản xuất trong đoạn \(\left[30;110\right]\).
Khi bán hết �x sản phẩm thì số tiền thu được là: 170�170x (nghìn đồng).
Điều kiện để nhà sản xuất không bị lỗ là 170�≥�2+30�+3300⇔�2−140�+3300≤0170x≥x2+30x+3300⇔x2−140x+3300≤0.
Xét �2−140�+3300=0⇒�=30x2−140x+3300=0⇒x=30 hoặc �=110x=110.
Bảng xét dấu �(�)=�2−140�+3300f(x)=x2−140x+3300:
∞!aaaaa + ∞ − + ∞ − xf(x)00 + 30110
Ta có: �2−140�+3300≤0⇔�∈[30;110]x2−140x+3300≤0⇔x∈[30;110].
Vậy nếu nhà sản xuất làm ra từ 3030 đến 110110 sản phẩm thì họ sẽ không bị lỗ.

a) Tọa độ vector pháp tuyến của đường BC là \(\overrightarrow{n_{BC}}=\left(1;-1\right)\)
\(\Rightarrow\) Tọa độ vector pháp tuyến của đường AH là \(\overrightarrow{n_{AH}}=\left(1;1\right)\)
\(\Rightarrow AH:x+y+m=0\) với \(m\inℝ\)
Mà AH đi qua A nên tọa độ điểm A thỏa mãn pt đường thẳng AH \(\Rightarrow-1-2+m=0\) \(\Leftrightarrow m=3\)
Vậy \(AH:x+y+3=0\)
b) Gọi d là đường thẳng chứa đường trung bình ứng với cạnh BC của tam giác ABC. Khi đó \(d//BC\) nên \(\overrightarrow{n_{BC}}=\overrightarrow{n_d}=\left(1;-1\right)\) (với \(\overrightarrow{n_d}\) là vector pháp tuyến của đường thẳng d) \(\Rightarrow d:x-y+n=0\) \(\left(n\inℝ\right)\)
Mặt khác, tọa độ H là nghiệm của hệ \(\left\{{}\begin{matrix}x-y+4=0\\x+y+3=0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x=-\dfrac{7}{2}\\y=\dfrac{1}{2}\end{matrix}\right.\) \(\Rightarrow H\left(-\dfrac{7}{2};\dfrac{1}{2}\right)\)
Gọi \(I\left(x_I;y_I\right)\) là trung điểm AH \(\Rightarrow\) \(\left\{{}\begin{matrix}x_I=\dfrac{x_A+x_H}{2}=\dfrac{-1-\dfrac{7}{2}}{2}=-\dfrac{9}{4}\\y_I=\dfrac{y_A+y_H}{2}=\dfrac{-2+\dfrac{1}{2}}{2}=-\dfrac{3}{4}\end{matrix}\right.\)
Do d là đường trung bình ứng với cạnh BC của tam giác ABC nên d đi qua trung điểm I của đường cao AH \(\Rightarrow-\dfrac{9}{4}-\left(-\dfrac{3}{4}\right)+n=0\) \(\Leftrightarrow n=\dfrac{3}{2}\) \(\Rightarrow d:x-y+\dfrac{3}{2}=0\)

+ Bước 1: Chọn 2 học sinh khối C, 13 học sinh khối B hoặc khối A có C25C1325C52C2513 cách.
+ Bước 2: Chọn 2 học sinh khối C, 13 học sinh khối B và khối A không thỏa mãn yêu cầu.
- Trường hợp 1: Chọn 2 học sinh khối C, 10 học sinh khối B và 3 học sinh khối A có C25C1010C315C52C1010C153 cách.
- Trường hợp 2: Chọn 2 học sinh khối C, 9 học sinh khối B và 4 học sinh khối A có C25C910C415C52C109C154 cách.
Vậy có C25(C1325−C1010C315−C910C415)=51861950C52C2513−C1010C153−C109C154=51861950 cách.

Imagination never end.Every second,million of new imagines appear in the world.I also just imagine something:Nowaday,English is very important to everyone.So,I want to create my new app for everyone.It will be named "Edo".To use this app,like other apps that you always use,you must make an account and set your profile.Then,you start learning.Here,you can learn reading,writing and listening.The app will correct the mistakes in your writing,in your words of speaking ... Also,you can learn more by speaking English with friends all over the world.
English has been one of the most widely spoken languages worldwide for a really long time. If you need to study abroad, you must know how to speak English fluently, especially if you don't know how to speak the country's native language. Because of that, English has become really important to everyone. And for that, I decided to create an app called "EngEdu". EngEdu is an app that will help you learn English online anywhere. It is pretty simple to use this app. To begin with, you must make an account and set up your profile. You can customize your profile as you like. After setting up your profile, you may start learning. This app covers all of the basic skills, including reading, writing, listening, and speaking, and also provides you with vocabulary and grammar lessons. You can also connect with other learners with the app's chatting feature. Besides all of the basic learning features, EngEdu also has multiple more fun features, such as competition, practice, assessment, etc. To conclude, I assure you that this app can help you reach higher levels in your English and will be of great help in your career.
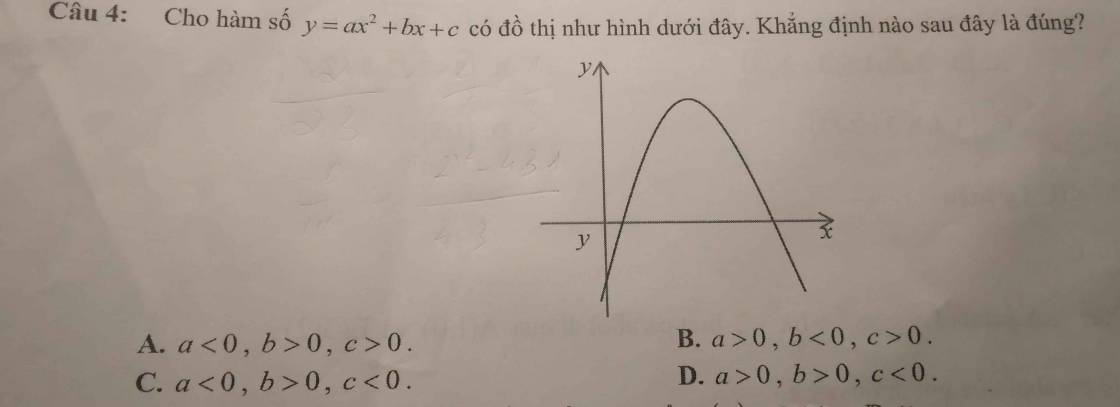
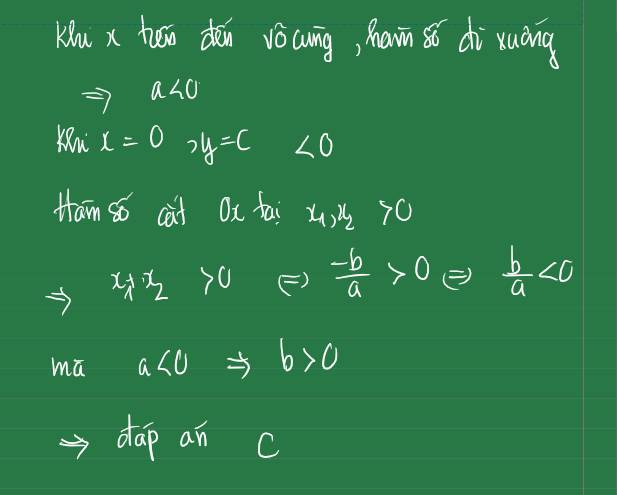




Hàm là \(y=mx^2-\left(m^2+1\right)x+3\) đúng không nhỉ?
- Với \(m=0\) hàm nghịch biến trên R (không thỏa)
- Với \(m\ne0\) hàm số đồng biến trên khoảng đã cho khi:
\(\left\{{}\begin{matrix}m>0\\\dfrac{m^2+1}{2m}\le1\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}m>0\\m^2+1\le2m\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}m>0\\\left(m-1\right)^2\le0\end{matrix}\right.\)
\(\Rightarrow m=1\)
anh ơi tại sao -b\(-\frac{b}{2a}\le1\) ạ