Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
\(K=\frac{a^2}{c\left(a^2+c^2\right)}+\frac{b^2}{a\left(a^2+b^2\right)}+\frac{c^2}{b\left(b^2+c^2\right)}\left(a,b,c>0\right)\).
Ta có:
\(\frac{a^2}{c\left(a^2+c^2\right)}=\frac{\left(a^2+c^2\right)-c^2}{c\left(a^2+c^2\right)}=\frac{a^2+c^2}{c\left(a^2+c^2\right)}-\frac{c^2}{c\left(a^2+c^2\right)}\)\(=\frac{1}{c}-\frac{c^2}{c\left(a^2+c^2\right)}\).
Vì \(a,c>0\)nên áp dụng bất đẳng thức Cô-si cho 2 số dương, ta được:
\(a^2+c^2\ge2ac\).
\(\Leftrightarrow c\left(a^2+c^2\right)\ge2ac^2\).
\(\Rightarrow\frac{1}{c\left(a^2+c^2\right)}\le\frac{1}{2ac^2}\)
\(\Leftrightarrow\frac{c^2}{c\left(a^2+c^2\right)}\le\frac{c^2}{2ac^2}=\frac{1}{2a}\).
\(\Leftrightarrow-\frac{c^2}{c\left(a^2+c^2\right)}\ge-\frac{1}{2a}\).
\(\Leftrightarrow\frac{1}{c}-\frac{c^2}{c\left(a^2+c^2\right)}\ge\frac{1}{c}-\frac{1}{2a}\)
\(\Leftrightarrow\frac{a^2}{c\left(a^2+c^2\right)}\ge\frac{1}{c}-\frac{1}{2a}\left(1\right)\)
Dấu bằng xảy ra \(\Leftrightarrow a=c>0\) .
Chứng minh tương tự, ta được:
\(\frac{b^2}{a\left(a^2+b^2\right)}\ge\frac{1}{a}-\frac{1}{2b}\left(a,b>0\right)\left(2\right)\)
Dấu bằng xảy ra \(\Leftrightarrow a=b>0\)
Chứng minh tương tự, ta dược:
\(\frac{c^2}{b\left(b^2+c^2\right)}\ge\frac{1}{b}-\frac{1}{2c}\left(b,c>0\right)\left(3\right)\).
Dấu bằng xảy ra \(\Leftrightarrow b=c>0\).
Từ \(\left(1\right),\left(2\right),\left(3\right)\), ta được:
\(\frac{a^2}{c\left(a^2+c^2\right)}+\frac{b^2}{a\left(a^2+b^2\right)}+\frac{c^2}{b\left(b^2+c^2\right)}\ge\)\(\frac{1}{c}-\frac{1}{2a}+\frac{1}{a}-\frac{1}{2b}+\frac{1}{b}-\frac{1}{2c}\).
\(\Leftrightarrow K\ge\left(\frac{1}{a}+\frac{1}{b}+\frac{1}{c}\right)-\frac{1}{2}\left(\frac{1}{a}+\frac{1}{b}+\frac{1}{c}\right)\).
\(\Leftrightarrow K\ge\frac{1}{2}\left(\frac{1}{a}+\frac{1}{b}+\frac{1}{c}\right)\).
\(\Leftrightarrow K\ge\frac{1}{2}\left(\frac{ab+bc+ca}{abc}\right)\).
Mà \(ab+bc+ca=3abc\)(theo đề bài).
Do đó \(K\ge\frac{1}{2}.\frac{3abc}{abc}\).
\(\Leftrightarrow K\ge\frac{3abc}{2abc}\).
\(\Leftrightarrow K\ge\frac{3}{2}\).
Dấu bằng xảy ra.
\(\Leftrightarrow\hept{\begin{cases}a=b=c>0\\ab+bc+ca=3abc\end{cases}}\Leftrightarrow a=b=c=1\).
Vậy \(minK=\frac{3}{2}\Leftrightarrow a=b=c=1\).
a) Ta có:
4>3⇔√4>√3⇔2>√3⇔2.2>2.√3⇔4>2√34>3⇔4>3⇔2>3⇔2.2>2.3⇔4>23
Cách khác:
Ta có:
⎧⎨⎩42=16(2√3)2=22.(√3)2=4.3=12{42=16(23)2=22.(3)2=4.3=12
Vì 16>12⇔√16>√1216>12⇔16>12
Hay 4>2√34>23.
b) Vì 5>4⇔√5>√45>4⇔5>4
⇔√5>2⇔5>2
⇔−√5<−2⇔−5<−2 (Nhân cả hai vế bất phương trình trên với −1−1)
Vậy −√5<−2−5<−2.
a, Ta có : \(4=\sqrt{16}\); \(2\sqrt{3}=\sqrt{4.3}=\sqrt{12}\)
Do 12 < 16 hay \(2\sqrt{3}< 4\)
b, Ta có : \(-2=-\sqrt{4}\)
Do \(4< 5\Rightarrow\sqrt{4}< \sqrt{5}\Rightarrow-\sqrt{4}>-\sqrt{5}\)
Vậy \(-2>-\sqrt{5}\)
a) Ta có:
+)√25+9=√34+)25+9=34.
+)√25+√9=√52+√32=5+3+)25+9=52+32=5+3
=8=√82=√64=8=82=64.
Vì 34<6434<64 nên √34<√6434<64
Vậy √25+9<√25+√925+9<25+9
b) Với a>0,b>0a>0,b>0, ta có
+)(√a+b)2=a+b+)(a+b)2=a+b.
+)(√a+√b)2=(√a)2+2√a.√b+(√b)2+)(a+b)2=(a)2+2a.b+(b)2
=a+2√ab+b=a+2ab+b
=(a+b)+2√ab=(a+b)+2ab.
Vì a>0, b>0a>0, b>0 nên √ab>0⇔2√ab>0ab>0⇔2ab>0
⇔(a+b)+2√ab>a+b⇔(a+b)+2ab>a+b
⇔(√a+√b)2>(√a+b)2⇔(a+b)2>(a+b)2
⇔√a+√b>√a+b⇔a+b>a+b (đpcm)
a, Ta có : \(\sqrt{25+9}=\sqrt{34}\)
\(\sqrt{25}+\sqrt{9}=5+3=8=\sqrt{64}\)
mà 34 < 64 hay \(\sqrt{25+9}< \sqrt{25}+\sqrt{9}\)
b, \(\sqrt{a+b}< \sqrt{a}+\sqrt{b}\)
bình phương 2 vế ta được : \(a+b< a+2\sqrt{ab}+b\)
\(\Leftrightarrow2\sqrt{ab}>0\)vì \(a;b>0\)nên đẳng thức này luôn đúng )
Vậy ta có đpcm
a) Điều kiện: x≥0x≥0
√16x=816x=8⇔(√16x)2=82⇔(16x)2=82 ⇔16x=64⇔16x=64 ⇔x=6416⇔x=4⇔x=6416⇔x=4 (thỏa mãn điều kiện)
Vậy x=4x=4.
Cách khác:
√16x=8⇔√16.√x=8⇔4√x=8⇔√x=2⇔x=22⇔x=416x=8⇔16.x=8⇔4x=8⇔x=2⇔x=22⇔x=4
b) Điều kiện: 4x≥0⇔x≥04x≥0⇔x≥0
√4x=√54x=5 ⇔(√4x)2=(√5)2⇔4x=5⇔x=54⇔(4x)2=(5)2⇔4x=5⇔x=54 (thỏa mãn điều kiện)
Vậy x=54x=54.
c) Điều kiện: 9(x−1)≥0⇔x−1≥0⇔x≥19(x−1)≥0⇔x−1≥0⇔x≥1
√9(x−1)=219(x−1)=21⇔3√x−1=21⇔3x−1=21⇔√x−1=7⇔x−1=7 ⇔x−1=49⇔x=50⇔x−1=49⇔x=50 (thỏa mãn điều kiện)
Vậy x=50x=50.
Cách khác:
√9(x−1)=21⇔9(x−1)=212⇔9(x−1)=441⇔x−1=49⇔x=509(x−1)=21⇔9(x−1)=212⇔9(x−1)=441⇔x−1=49⇔x=50
d) Điều kiện: x∈Rx∈R (vì 4.(1−x)2≥04.(1−x)2≥0 với mọi x)x)
√4(1−x)2−6=04(1−x)2−6=0⇔2√(1−x)2=6⇔2(1−x)2=6 ⇔|1−x|=3⇔|1−x|=3 ⇔[1−x=31−x=−3⇔[1−x=31−x=−3 ⇔[x=−2x=4⇔[x=−2x=4
Vậy x=−2;x=4.
a, \(\sqrt{16x}=8\Leftrightarrow4\sqrt{x}=8\Leftrightarrow\sqrt{x}=2\Leftrightarrow x=4\)
b, \(\sqrt{4x}=\sqrt{5}\)ĐK : x \(\ge0\)
bình phương 2 vế ta được : \(4x=5\Leftrightarrow x=\frac{5}{4}\)
c, \(\sqrt{9\left(x-1\right)}=21\Leftrightarrow3\sqrt{x-1}=21\Leftrightarrow\sqrt{x-1}=7\)
bình phương 2 vế ta được : \(x-1=49\Leftrightarrow x=50\)
d, \(\sqrt{4\left(1-x\right)^2}-6=0\Leftrightarrow2\left|1-x\right|=6\Leftrightarrow\left|1-x\right|=3\)
TH1 : \(1-x=3\Leftrightarrow x=-2\)
TH2 : \(1-x=-3\Leftrightarrow x=4\)
a) Ta có:
√4(1+6x+9x2)24(1+6x+9x2)2 =√4.√(1+6x+9x2)2=4.(1+6x+9x2)2
=√4.√(1+2.3x+32.x2)2=4.(1+2.3x+32.x2)2
=√22.√[12+2.3x+(3x)2]2=22.[12+2.3x+(3x)2]2
=2.√[(1+3x)2]2=2.[(1+3x)2]2
=2.∣∣(1+3x)2∣∣=2.|(1+3x)2|
=2(1+3x)2=2(1+3x)2.
(Vì (1+3x)2>0(1+3x)2>0 với mọi xx nên ∣∣(1+3x)2∣∣=(1+3x)2|(1+3x)2|=(1+3x)2)
Thay x=−√2x=−2 vào biểu thức rút gọn trên, ta được:
2[1+3.(−√2)]2=2(1−3√2)22[1+3.(−2)]2=2(1−32)2.
Bấm máy tính, ta được: 2(1−3√2)2≈21,0292(1−32)2≈21,029.
b) Ta có:
√9a2(b2+4−4b)=√32.a2.(b2−4b+4)9a2(b2+4−4b)=32.a2.(b2−4b+4)
=√(3a)2.(b2−2.b.2+22)=(3a)2.(b2−2.b.2+22)
=√(3a)2.√(b−2)2=(3a)2.(b−2)2
=|3a|.|b−2|=|3a|.|b−2|
Thay a=−2a=−2 và b=−√3b=−3 vào biểu thức rút gọn trên, ta được:
|3.(−2)|.∣∣−√3−2∣∣=|−6|.∣∣−(√3+2)∣∣|3.(−2)|.|−3−2|=|−6|.|−(3+2)|
=6.(√3+2)=6√3+12=6.(3+2)=63+12.
Bấm máy tính, ta được: 6√3+12≈22,39263+12≈22,392.
a) Ta có:
.
(Vì với mọi nên )
Thay vào biểu thức rút gọn trên, ta được:
.
Bấm máy tính, ta được: .
b) Ta có:
Thay và vào biểu thức rút gọn trên, ta được:
.
Bấm máy tính, ta được: .
a) (2-\(\sqrt{3}\))(2+\(\sqrt{3}\))=22-(\(\sqrt{3}\))2=4-3=1 (ĐPCM)
Câu a: Ta có:
(2−√3)(2+√3)=22−(√3)2=4−3=1(2−3)(2+3)=22−(3)2=4−3=1
Câu b:
Ta tìm tích của hai số (√2006−√2005)(2006−2005) và (√2006+√2005)(2006+2005)
Ta có:
(√2006+√2005).(√2006−√2005)(2006+2005).(2006−2005)
= (√2006)2−(√2005)2(2006)2−(2005)2
=2006−2005=1=2006−2005=1
Do đó (√2006+√2005).(√2006−√2005)=1(2006+2005).(2006−2005)=1
⇔√2006−√2005=1√2006+√2005⇔2006−2005=12006+2005
Vậy hai số trên là nghịch đảo của nhau.
a) \(\sqrt{13^2-12^2}\)=\(\sqrt{\left(13-12\right)\left(13+12\right)}\)=\(\sqrt{1x25}\)=5
Câu a: Ta có:
√132−122=√(13+12)(13−12)132−122=(13+12)(13−12)
=√25.1=√25=25.1=25
=√52=|5|=5=52=|5|=5.
Câu b: Ta có:
√172−82=√(17+8)(17−8)172−82=(17+8)(17−8)
=√25.9=√25.√9=25.9=25.9
=√52.√32=|5|.|3|=52.32=|5|.|3|.
=5.3=15=5.3=15.
Câu c: Ta có:
√1172−1082=√(117−108)(117+108)1172−1082=(117−108)(117+108)
=√9.225=9.225 =√9.√225=9.225
=√32.√152=|3|.|15|=32.152=|3|.|15|
=3.15=45=3.15=45.
Câu d: Ta có:
√3132−3122=√(313−312)(313+312)3132−3122=(313−312)(313+312)
=√1.625=√625=1.625=625
=√252=|25|=25=252=|25|=25.
1. The conversation takes place on the school's 60th anniversary.
2. Our grandparents used to live in an extended family.
3. Because it explains a lot about how the school was in the past.
4. The roof was made of tiles and some tiles were broken. The window frames were made of wood and some of them were missing
5. They can learn that they are lucky to have such great learning facilities nowadays.
6. My mother is a sympathe woman. She always cares about how we feel.
7. The boys are willing to do what you want the to. They are really obedient.
8. Having students work in groups, she hoped they could learn to be cooperative.
9. She is tolerant with her children even when the misbehave.
10. Nowadays the nuclear family is becoming more commor in the cities
* Theo em thì các câu hỏi thuộc chủ đề .. không nên post vì đã có hướng dẫn giải rồi, members sẽ cop và trả lời lại
Write complete sentences, using the words/ phrases given in their correct forms. You can add some more necessary words, but you have to use all the words given.
1. The conversation / take / place / the school's 60th anniversary.
=> The conversation is taken place on the school's 60th anniversary.
2. Our grandparents / use / live in an extended family.
=> Our grandparents used to live in an extended family.
3. Because / it explain / a lot about how the school / in the past.
=> . Because it explains a lot about how the school was in the past.
4. The roof / made of tiles / some tiles were broken. / The window frames / made of wood / some of them were missing.\
=> The roof was made of tiles and some tiles were broken. The window frames were made of wood and some of them were missing.
5. They / learn that they / lucky / have / such great learning facilities / nowadays.
=> They can learn that they are lucky to have such great learning facilities nowadays.
6. My mother / a sympathe woman. / She always care / how / we feel.
=>My mother is a sympathe woman. She always cares about how we feel.
7. The boys / willing to do / what you want / to. / They / really obedient.
=> The boys are willing to do what you want them to. They are really obedient.
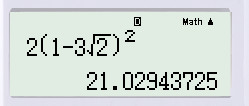
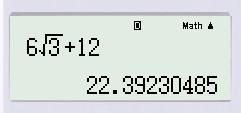
a) Gọi \(A\in Ox;B\in Oy\Rightarrow\Delta OAB\)vuông tại O
Đường thẳng (d) giao Ox tại điểm \(A\left(x;0\right)\)-> thay y=0 vào hàm số ta được: 0=(m+2)x+3 -> (m+2)x=-3 -> \(x=\frac{-3}{m+2}\)
-> Điểm \(A\left(\frac{-3}{m+2};0\right)\)-> \(OA=|\frac{-3}{m+2}|\)(OA>0)
Đường thẳng (d) giao Oy tại điểm \(B\left(0;y\right)\)-> thay x=0 vào hàm số ta được: y=(m+2).0+3=3
-> Điểm \(B\left(0;3\right)\)-> \(OB=3\)
Có: \(S_{\Delta OAB}=\frac{3}{4}=\frac{1}{2}OA\cdot OB=\frac{1}{2}\cdot3\cdot\frac{|-3|}{|m+2|}=\frac{3\cdot3}{2|m+2|}=\frac{9}{2|m+2|}\)
\(\Rightarrow6|m+2|=36\Leftrightarrow|m+2|=6\Leftrightarrow\orbr{\begin{cases}m+2=6\\m+2=-6\end{cases}}\Leftrightarrow\orbr{\begin{cases}m=4\\m=-8\end{cases}}\)(TM)
Vậy...
b) ĐK: OA>0
\(\Delta OAB\)vuông tại O -> \(AB=\sqrt{OA^2+OB^2}=\sqrt{3^2+\left(\frac{-3}{m+2}\right)^2}=\sqrt{9+\frac{9}{\left(m+2\right)^2}}\)
Kẻ \(OH\perp d\)tại H -> OH là khoảng cách từ đường thẳng từ O đến d
Áp dụng htl trong \(\Delta OAB\)vuông tại O, đường cao OH -> \(OA.OB=OH.AB\)
\(\rightarrow3\cdot\frac{|-3|}{|m+2|}=\frac{3\sqrt{2}}{2}.\sqrt{9+\frac{9}{\left(m+2\right)^2}}\)
\(\Leftrightarrow\left(3\cdot\frac{|-3|}{|m+2|}\right)^2=\left(\frac{3\sqrt{2}}{2}\right)^2\left(9+\frac{9}{\left(m+2\right)^2}\right)\)
\(\Leftrightarrow\frac{81}{\left(m+2\right)^2}=\frac{9\cdot9}{2}+\frac{9\cdot9}{2\left(m+2\right)^2}\Leftrightarrow\frac{81}{\left(m+2\right)^2}=\frac{81}{2}+\frac{81}{2\left(m+2\right)^2}\)
\(\Leftrightarrow\frac{1}{\left(m+2\right)^2}-\frac{1}{2}-\frac{1}{2\left(m+2\right)^2}=0\Leftrightarrow\frac{2-\left(m+2\right)^2-1}{2\left(m+2\right)^2}=0\) ( \(2\left(m+2\right)^2>0\))
\(\Rightarrow1-\left(m+2\right)^2=0\Rightarrow\left(m+2\right)^2=1\Leftrightarrow\orbr{\begin{cases}m+2=1\\m+2=-1\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}m=-1\\m=-3\end{cases}}\)(TM)
Vậy...
Hì cậu kiểm tra xem tớ có sai dấu hay sai bước chỗ nào với nhé vì tớ hay cẩu thả lắm:'33