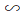\(M=\sqrt{x^2-2x+11}\)

\(=\sqrt{x^2-2x+1+10}\)

\(=\sqrt{\left(x-1\right)^2+10}\)

Nhận thấy (x - 1)² \(\ge0\)

=> (x - 1)² + 10 \(\ge10\)

=> \(\sqrt{\left(x-1\right)^2+10}\ge\sqrt{10}\)

=> Min M = \(\sqrt{10}\)

Dấu "=" xảy ra <=> x - 1 = 0

<=> x = 1

Vậy Min M = \(\sqrt{10}\)khi x = 1

M nhỏ nhất khi \(x^2-2x+11\)nhỏ nhất.

Mà \(x^2-2x+11=\left(x^2-2x+1\right)+10=\left(x-1\right)^2+10\)

Lại có \(\left(x-1\right)^2\ge0\Leftrightarrow\left(x-1\right)^2+10\ge10\Leftrightarrow x^2-2x+11\ge10\)(đẳng thức xảy ra khi x = 1)

Do đó \(min_{x^2-2x+11}=10\Leftrightarrow x=1\)

Khi đó \(M=\sqrt{x^2-2x+11}=\sqrt{10}\)

Vậy GTNN của M là 10 khi x = 1.

BN hok ở thcs Chu văn an à , trường ở đâu ?

Ta có : \(\hept{\begin{cases}\Delta A'B'C'\approx\Delta A''B''C''\\\Delta A''B''C''\approx\Delta ABC\end{cases}}\)

=> \(\hept{\begin{cases}\frac{A'B'}{A''B''}=k_1\\\frac{AB}{A''B''}=\frac{1}{k_2}\end{cases}}\)

=> \(\frac{A'B'}{AB}=k_1.k_2\)

=> Tỉ số đồng dạng khi \(\Delta A'B'C\approx\Delta ABC\) là k₁.k₂

\(P=\frac{x^2-2x+1989}{x^2}\)

\(\Leftrightarrow Px^2=x^2-2x+1989\)

\(\Leftrightarrow x^2\left(1-P\right)-2x+1989=0\)

\(\Delta=4-4\left(1-P\right)1989\ge0\)

\(\Leftrightarrow P\ge\frac{1988}{1989}\)có GTNN là \(\frac{1988}{1989}\)

Dấu '' = '' xảy ra \(\Leftrightarrow x=1989\)

Vậy \(P_{min}=\frac{1988}{1989}\) tại \(x=1989\)

ai lại chụp đề thi lên đây

thế này là gian luộn r nha! ko đc đâu

helppppppppppppppppppppppppppppppppppppppp

Câu a bạn chỉ việc thay x = 9 vào A rồi tính thôi mà.

b) Bạn tự tìm đkxđ nhé.

Ta có: \(x+2\sqrt{x}-3=x-\sqrt{x}+3\sqrt{x}-3\)

\(=\sqrt{x}\left(\sqrt{x}-1\right)+3\left(\sqrt{x}-1\right)=\left(\sqrt{x}-1\right)\left(\sqrt{x}+3\right)\)

\(\Rightarrow B=\frac{3\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}-\frac{2}{\sqrt{x}+3}\)

\(B=\frac{3\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}-\frac{2\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}\)

\(B=\frac{3\sqrt{x}+1-2\sqrt{x}+2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}=\frac{\sqrt{x}+3}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}=\frac{1}{\sqrt{x}-1}\)(đpcm)

c) Ta có \(\frac{A}{B}=\frac{\sqrt{x}+4}{\sqrt{x}-1}:\frac{1}{\sqrt{x-1}}=\frac{\left(\sqrt{x}+4\right)\left(\sqrt{x}-1\right)}{\sqrt{x}-1}=\sqrt{x}+4\)

Để \(\frac{A}{B}=\frac{x}{4}+5\)thì \(\sqrt{x}+4=\frac{x}{4}+5\Leftrightarrow\frac{x}{4}-\sqrt{x}+1=0\)

\(\Leftrightarrow4\left(\frac{x}{4}-\sqrt{x}+1\right)=0\Leftrightarrow x-4\sqrt{x}+4=0\Leftrightarrow\left(\sqrt{x}-2\right)^2=0\Leftrightarrow\sqrt{x}=2\Leftrightarrow x=4\)

Vậy ...