Phân tích đa thức sau thành nhân tử.
(x + 5) ^ 2 - 4x ^ 2
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


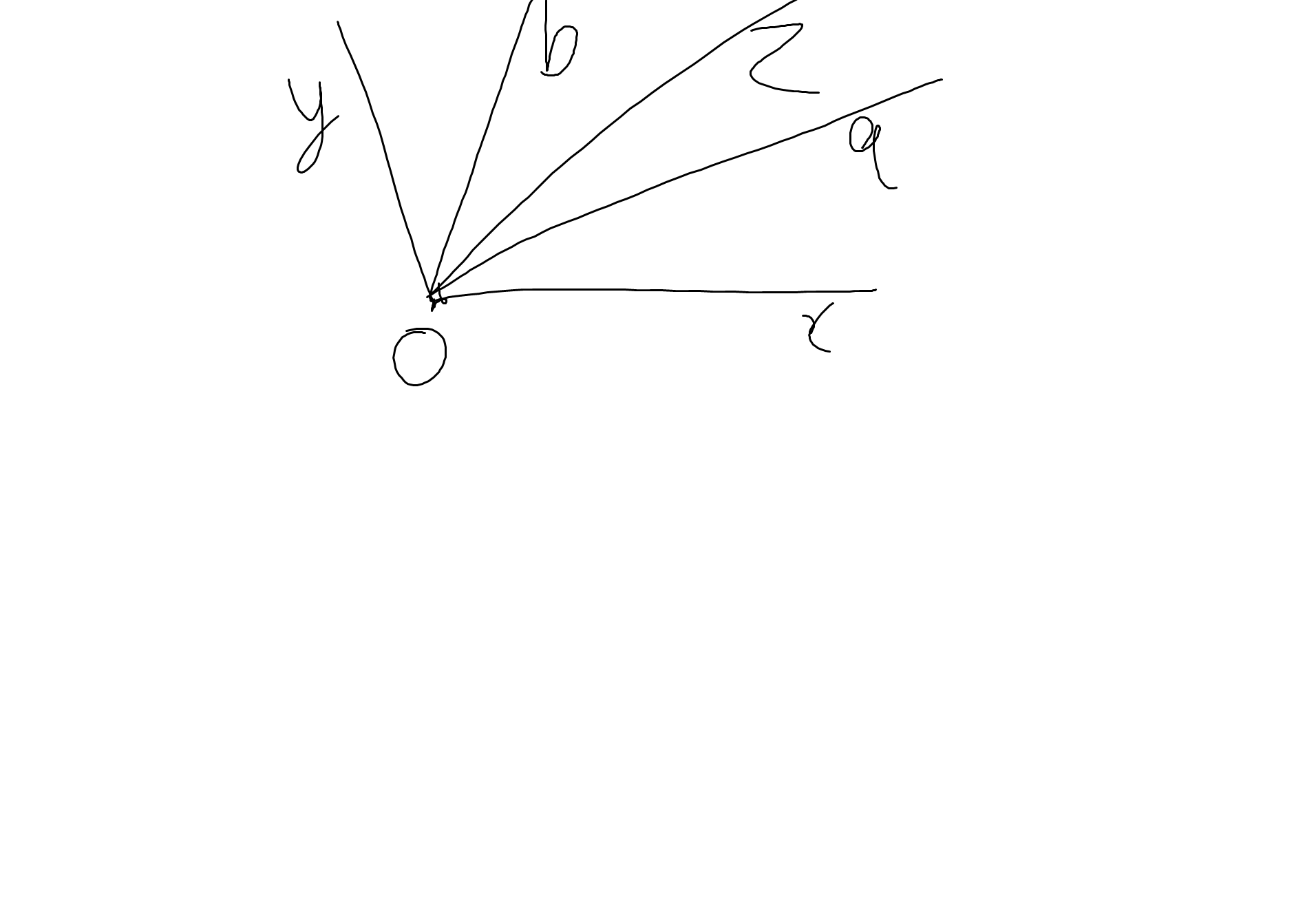
Oa là phân giác của góc xOz
=>\(\widehat{zOa}=\dfrac{\widehat{xOz}}{2}\)
Ob là phân giác của góc zOy
=>\(\widehat{zOb}=\dfrac{\widehat{zOy}}{2}\)
\(\widehat{aOb}=\widehat{zOa}+\widehat{zOb}=\dfrac{1}{2}\left(\widehat{xOz}+\widehat{zOy}\right)\)
\(=\dfrac{1}{2}\cdot\widehat{xOy}=\dfrac{1}{2}\cdot150^0=75^0\)

a: Sau 3 giờ, xe máy đi được: 3x40=120(km)
Hiệu vận tốc hai xe là 60-40=20(km/h)
Hai xe gặp nhau sau khi ô tô đi được: 120:20=6(giờ)
b: Điểm gặp nhau cách A:
6x60=360(km)

Kẻ tia `Ot` là tia đối của tia `Ox`
=> \(\widehat{xOt}=180^o\)
Ta có:
\(\widehat{yOt}=\widehat{xOt}-\widehat{xOy}=180^o-120^o=60^o\)
=> \(\widehat{tOz}=\widehat{zOy}-\widehat{yOt}=134^o-60^o=74^o\)
Mà \(\widehat{xOz};\widehat{zOt}\) là 2 góc kề bù
=> \(\widehat{zOx}+\widehat{zOt}=\widehat{xOt}\)
=> \(\widehat{xOz}=\widehat{xOt}-\widehat{tOz}=180^o-74^o=106^o\)
Vậy ...

Tổng chiều dài và rộng của hình chữ nhật là:
`21,6 : 2 = 10,8 (dm)`
Đổi `80% =` \(\dfrac{4}{5}\)
Ta có sơ đồ:
Chiều dài: (5 phần)
Chiều rộng: (4 phần)
Tổng số phần bằng nhau là:
`5+4 = 9` (phần)
Giá trị 1 phần là:
`10,8 : 9 = 1,2 (dm)`
Chiều dài hình chữ nhật:
`1,2` x `5 = 6 (dm)`
Chiều rộng hình chữ nhật là:
`10,8 - 6 = 4,8 (dm)`
Diện tích hình chữ nhật là:
`6` x `4,8 = 28,8 (dm^2)`
Đáp số: `28,8 dm^2`

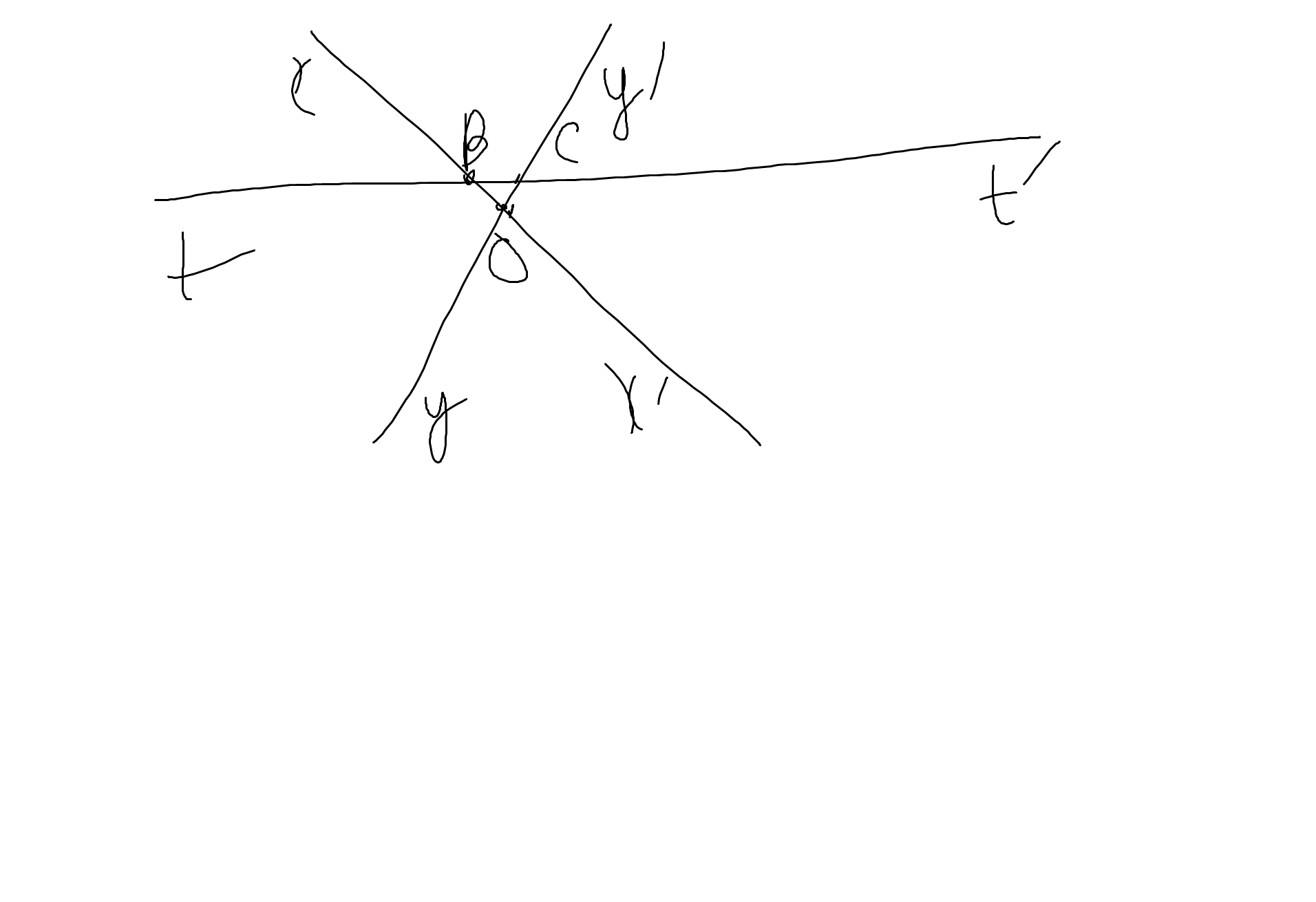
Bài 3: Các cặp góc so le trong là: \(\widehat{tBO};\widehat{BOC}\); \(\widehat{OBC};\widehat{yOB}\); \(\widehat{BCO};\widehat{x'OC}\); \(\widehat{t'CO};\widehat{BOC}\)
Các cặp góc đồng vị là:
\(\widehat{xBt};\widehat{xOy}\); \(\widehat{tBO};\widehat{x'Oy}\); \(\widehat{y'Ct'};\widehat{x'Oy'}\); \(\widehat{t'CO};\widehat{x'Oy}\)
Bài 2:
Các cặp góc so le trong là \(\widehat{FEC};\widehat{ACB}\)
Các cặp góc đồng vị là \(\widehat{ADE};\widehat{ABC}\); \(\widehat{AED};\widehat{ACB}\)
Các cặp góc trong cùng phía là: \(\widehat{BDE};\widehat{B}\); \(\widehat{DEC};\widehat{ECB}\)

\(x^3+ax+b\\ =\left(x^3+4x^2+3x\right)+\left(-4x^2-16x-12\right)+\left(a+13\right)x+\left(b+12\right)\\ =x\left(x^2+4x+3\right)-4\left(x^2+4x+3\right)+\left(a+13\right)x+\left(b+12\right)\\ =\left(x-4\right)\left(x^2+4x+3\right)+\left(a+13\right)x+\left(b+12\right)\)
Để `x^3+ax+b` chia hết cho `x^2+4x+3` thì:
\(\left\{{}\begin{matrix}a+13=0\\b+12=0\end{matrix}\right.=>\left\{{}\begin{matrix}a=-13\\b=-12\end{matrix}\right.\)

`x (2x - 9) = 3x(x - 5) `
`<=> 2x^2 - 9x = 3x^2 - 15x`
`<=> 3x^2 - 2x^2 - 15x + 9x =0`
`<=> x^2 - 6x = 0`
`<=> x(x-6) = 0`
`<=> x = 0` hoặc `x - 6 = 0`
`<=> x = 0` hoặc `x = 6`
Vậy ....

\(\left(4x+2\right)\left(x^2+1\right)=0\)(1)
Ta có: `x^2>=0` với mọi x
`=>x^2+1>=1>0` với mọi x
`=>x^2+1≠0`
\(\left(1\right)\Leftrightarrow4x+2=0\\ \Leftrightarrow4x=-2\\ \Leftrightarrow x=-\dfrac{2}{4}=-\dfrac{1}{2}\)
`(4x + 2)(x^2 + 1) = 0`
Trường hợp 1:
`4x + 2 = 0`
`<=> 4x = -2`
`<=> x =` \(-\dfrac{1}{2}\)
Trường hợp 2:
`x^2 + 1 = 0`
`<=> x^2 = -1` (Không tồn tại `x`)
Vậy `x =` \(-\dfrac{1}{2}\)

\(g.x^3-3x^2+3x-1=0\\ \Leftrightarrow\left(x-1\right)^3=0\\ \Leftrightarrow x-1=0\\ \Leftrightarrow x=1\\ h.x\left(2x-7\right)-4x+14=0\\ \Leftrightarrow x\left(2x-7\right)-2\left(2x-7\right)=0\\ \Leftrightarrow\left(2x-7\right)\left(x-2\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}2x=7\\x=2\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=\dfrac{7}{2}\\x=2\end{matrix}\right.\\ k.\left(2x-5\right)^2\left(x+2\right)^2=0\\ \Leftrightarrow\left[{}\begin{matrix}2x-5=0\\x+2=0\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}2x=5\\x=-2\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{2}\\x=-2\end{matrix}\right.\\ l.x\left(2x-9\right)=3x\left(x-5\right)\\ \Leftrightarrow3x^2-15x-2x^2+9x=0\\ \Leftrightarrow x^2-6x=0\\ \Leftrightarrow x\left(x-6\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x=6\end{matrix}\right.\\ m.\left(x^2-2x+1\right)-4=0\\ \Leftrightarrow\left(x-1\right)^2=2^2\\ \Leftrightarrow\left[{}\begin{matrix}x-1=2\\x-1=-2\end{matrix}\right.\\ \Leftrightarrow\left[{}\begin{matrix}x=2+1=3\\x=-2+1=-1\end{matrix}\right.\)
a: (3x-2)(4x+5)=0
=>\(\left[{}\begin{matrix}3x-2=0\\4x+5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{2}{3}\\x=-\dfrac{5}{4}\end{matrix}\right.\)
c: \(\left(4x+2\right)\left(x^2+1\right)=0\)
mà \(x^2+1>=1>0\forall x\)
nên 4x+2=0
=>4x=-2
=>\(x=-\dfrac{1}{2}\)
d: (2x+7)(x-5)(5x+1)=0
=>\(\left[{}\begin{matrix}2x+7=0\\x-5=0\\5x+1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{7}{2}\\x=5\\x=-\dfrac{1}{5}\end{matrix}\right.\)
f: \(\left(x^2-4\right)\left(x-2\right)\left(3-2x\right)=0\)
=>\(\left(x-2\right)^2\cdot\left(x+2\right)\left(3-2x\right)=0\)
=>\(\left[{}\begin{matrix}x-2=0\\x+2=0\\3-2x=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-2\\x=\dfrac{3}{2}\end{matrix}\right.\)
\(\left(x+5\right)^2-4x^2\\=\left(x+5\right)^2-\left(2x\right)^2\\ =\left[\left(x+5\right)-2x\right]\left[\left(x+5\right)+2x\right]\\ =\left(x+5-2x\right)\left(x+5+2x\right)\\ =\left(-x+5\right)\left(3x+5\right)\)