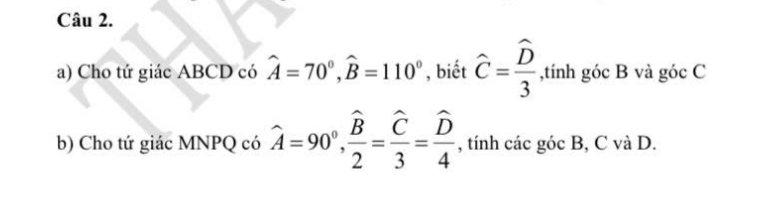
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(\widehat{C}=\widehat{B}+10^0=\widehat{A}+10^0+10^0=\widehat{A}+20^0\)
\(\widehat{D}=\widehat{C}+10^0=\widehat{A}+20^0+10^0=\widehat{A}+30^0\)
Xét tứ giác ABCD có \(\widehat{A}+\widehat{B}+\widehat{C}+\widehat{D}=360^0\)
=>\(\widehat{A}+\widehat{A}+10^0+\widehat{A}+20^0+\widehat{A}+30^0=360^0\)
=>\(4\cdot\widehat{A}=300^0\)
=>\(\widehat{A}=75^0\)
\(\widehat{B}=75^0+10^0=85^0\)
\(\widehat{C}=75^0+20^0=95^0\)
\(\widehat{D}=75^0+30^0=105^0\)

\(499^2+499+500\)
\(=499\cdot\left(499+1\right)+500\)
\(=500\cdot499+500=500\cdot500=250000\)

\(5y-7\) chia hết \(3-2y\)
\(\Rightarrow2\left(5y-7\right)⋮\left(3-2y\right)\)
\(\Rightarrow1-5\left(3-2y\right)⋮\left(3-2y\right)\)
\(\Rightarrow1⋮\left(3-2y\right)\)
\(\Rightarrow3-2y\inƯ\left(1\right)=\left\{-1;1\right\}\)
\(\Rightarrow y\in\left\{2;1\right\}\)
Do `y ∈ Z => {(5y - 7 ∈ Z),(3-2y ∈ Z):}`
Điều kiện: `3 - 2y ne 0 => 2y ne 3 => y ne 3/2 `
`5y - 7 vdots 3 - 2y`
`=> 10y - 14 vdots 3 - 2y`
Do `3 - 2y vdots 3 - 2y => 15 - 10y vdots 3 - 2y`
`=> 10y - 14 + 15 - 10y vdots 3 - 2y`
`=> 1 vdots 3 - 2y`
`=> 3 - 2y ∈ Ư(1) = {-1;1}`
`=> 2y ∈ {4;2}`
`=> y ∈ {2;1}` (Thỏa mãn)
Vậy `y ∈ {2;1}`

\(a+b+c=\dfrac{1}{a}+\dfrac{1}{b}+\dfrac{1}{c}\)
\(\Rightarrow a+b+c=\dfrac{ab+bc+ca}{abc}=ab+bc+ca\)
\(\Rightarrow a+b+c+\left(abc-1\right)=ab+bc+ca\) (do \(abc-1=0\) nên có thể thêm bớt)
\(\Rightarrow abc-ab-bc-ca+a+b+c-1=0\)
\(\Rightarrow ab\left(c-1\right)-b\left(c-1\right)-a\left(c-1\right)+c-1=0\)
\(\Rightarrow\left(c-1\right)\left(ab-b-a+1\right)=0\)
\(\Rightarrow\left(c-1\right)\left[b\left(a-1\right)-\left(a-1\right)\right]=0\)
\(\Rightarrow\left(c-1\right)\left(a-1\right)\left(b-1\right)=0\) (đpcm)

Câu 1: \(x^3+x-2=0\)
=>\(x^3-x^2+x^2-x+2x-2=0\)
=>\(\left(x-1\right)\left(x^2+x+2\right)=0\)
mà \(x^2+x+2=\left(x+\dfrac{1}{2}\right)^2+\dfrac{7}{4}>=\dfrac{7}{4}>0\forall x\)
nên x-1=0
=>x=1
Câu 3: \(x^4-10x^2-11x-10\)
\(=x^4-x^3-10x^2+x^3-x^2-10x+x^2-x-10\)
\(=x^2\left(x^2-x-10\right)+x\left(x^2-x-10\right)+\left(x^2-x-10\right)\)
\(=\left(x^2-x-10\right)\left(x^2+x+1\right)\)
Câu 5: \(x^3-x^2-14x+24\)
\(=x^3+4x^2-5x^2-20x+6x+24\)
\(=x^2\left(x+4\right)-5x\left(x+4\right)+6\left(x+4\right)\)
\(=\left(x+4\right)\left(x^2-5x+6\right)=\left(x+4\right)\left(x-2\right)\left(x-3\right)\)
Câu 6: \(x^3-5x^2+8x-4\)
\(=x^3-x^2-4x^2+4x+4x-4\)
\(=x^2\left(x-1\right)-4x\left(x-1\right)+4\left(x-1\right)\)
\(=\left(x-1\right)\left(x^2-4x+4\right)=\left(x-1\right)\left(x-2\right)^2\)
Câu 7:
\(\left(a-x\right)y^3-\left(a-y\right)x^3+\left(x-y\right)a^3\)
\(=a\cdot y^3-xy^3-a\cdot x^3+y\cdot x^3+\left(x-y\right)\cdot a^3\)
\(=a\left(y^3-x^3\right)-xy\left(y^2-x^2\right)+\left(x-y\right)a^3\)
\(=a\left(y-x\right)\left(y^2+xy+x^2\right)-xy\left(y-x\right)\left(y+x\right)-\left(y-x\right)a^3\)
\(=\left(y-x\right)\left[a\left(x^2+xy+y^2\right)-xy\left(x+y\right)-a^3\right]\)

\(\left(x+y\right)^2-2\left(x+y\right)+1\)
\(=\left(x+y-1\right)^2\) (HĐT số 2)

\(a,M=\left(x+1\right)^3-x^3+1-3x\left(x+1\right)\\ =x^3+3x^2+3x+1-x^3+1-3x^2-3x\\ =\left(x^3-x^3\right)+\left(3x^2-3x^2\right)+\left(3x-3x\right)+\left(1+1\right)\\ =2\)
Vậy giá trị của bt không phụ thuộc vào biến
\(b,\left(2x-1\right)^3-6x\left(2x-1\right)^2+12x^2\left(2x-1\right)-8x^3\\ =\left(2x-1\right)^3-3\cdot\left(2x-1\right)^2\cdot2x+3\cdot\left(2x-1\right)\cdot\left(2x\right)^2-\left(2x\right)^3\\ =\left(2x-1-2x\right)^3\\ =\left(-1\right)^3=-1\)
Vậy giá trị của bt không phụ thuộc vào biến
\(c,P=\left(x+y+1\right)^3-\left(x+y-1\right)^3-6\left(x+y\right)^2\\ =\left(x+y+1-x-y+1\right)\left[\left(x+y+1\right)^2+\left(x+y+1\right)\left(x+y-1\right)+\left(x+y-1\right)^2\right]-6\left(x+y\right)^2\\ =2\left[\left(x+y\right)^2+2\left(x+1\right)+1+\left(x+y\right)^2-1+\left(x+y\right)^2-2\left(x+y\right)+1\right]-6\left(x+y\right)^2\\ =2\left[3\left(x+y\right)^2+1\right]-6\left(x+y\right)^2\\ =6\left(x+y\right)^2+2-6\left(x+y\right)^2\\ =2\)
Vậy giá trị của bt không phụ thuộc vào biến

\(a\left(b^2+c^2\right)+b\left(c^2+a^2\right)+c\left(a^2+b^2\right)+3ab\\ =ab^2+ac^2+bc^2+ba^2+ca^2+cb^2+3abc\\ =\left(a^2b+ab^2+abc\right)+\left(bc^2+b^2c+abc\right)+\left(ca^2+ac^2+abc\right)\\ =ab\left(a+b+c\right)+bc\left(a+b+c\right)+ca\left(a+b+c\right)\\ =\left(a+b+c\right)\left(ab+bc+ca\right)\)

Bài 3:
\(a,\left(x-3\right)^3-x^2\left(x+2\right)+11x=-108\\ \Leftrightarrow x^3-9x^2+27x-27-x^3-2x^2+11x^2=-108\\ \Leftrightarrow-11x^2+27x-27+11x^2=-108\\ \Leftrightarrow27x-27=-108\\ \Leftrightarrow27x=-108+27\\ \Leftrightarrow27x=-81\\ \Leftrightarrow x=-\dfrac{81}{27}\\ \Leftrightarrow x=-3\\ b,\left(2x+3\right)^3-8x\left(x+1\right)\left(x-1\right)=9x\left(4x-3\right)\\ \Leftrightarrow\left(8x^3+36x^2+54x+27\right)-8x\left(x^2-1\right)=36x^2-27x\\ \Leftrightarrow8x^3+36x^2+54x+27-8x^3+8x=36x^2-27x\\ \Leftrightarrow36x^2+62x+27=36x^2-27x\\ \Leftrightarrow62x+27=-27x\\ \Leftrightarrow62x+27x=-27\\ \Leftrightarrow89x=-27\\ \Leftrightarrow x=\dfrac{-27}{89}\)
Bài 4:
a: \(18^3-3\cdot18^2\cdot8+3\cdot18\cdot8^2-2^9\)
\(=18^3-3\cdot18^2\cdot8+3\cdot18\cdot8^2-8^3\)
\(=\left(18-8\right)^3=10^3=1000\)
b: \(997^3+9\cdot997^2+997\cdot27+27\)
\(=997^3+3\cdot997^2\cdot3+3\cdot997\cdot3^2+3^3\)
\(=\left(997+3\right)^3=1000^3=10^9\)
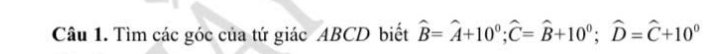
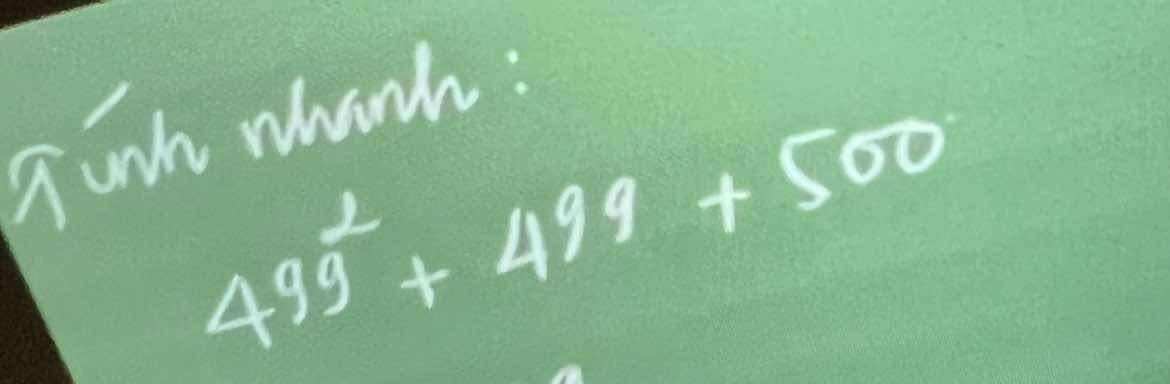



 giúp mình với
giúp mình với
a: Xét tứ giác ABCD có \(\widehat{A}+\widehat{B}+\widehat{C}+\widehat{D}=360^0\)
=>\(\widehat{C}+\widehat{D}=360^0-110^0-70^0=180^0\)
=>\(\dfrac{1}{3}\cdot\widehat{D}+\widehat{D}=180^0\)
=>\(\dfrac{4}{3}\cdot\widehat{D}=180^0\)
=>\(\widehat{D}=135^0\)
\(\widehat{C}=\dfrac{1}{3}\cdot135^0=45^0\)
b:
Sửa đề: Cho tứ giác ABCD.
Đặt \(\widehat{B}=x;\widehat{C}=y;\widehat{D}=z\)
\(\dfrac{\widehat{B}}{2}=\dfrac{\widehat{C}}{3}=\dfrac{\widehat{D}}{4}\)
=>\(\dfrac{x}{2}=\dfrac{y}{3}=\dfrac{z}{4}\)
Xét tứ giác ABCD có \(\widehat{A}+\widehat{B}+\widehat{C}+\widehat{D}=360^0\)
=>\(x+y+z=360^0-90^0=270^0\)
Áp dụng tính chất của dãy tỉ số bằng nhau, ta được:
\(\dfrac{x}{2}=\dfrac{y}{3}=\dfrac{z}{4}=\dfrac{x+y+z}{2+3+4}=\dfrac{270}{9}=30^0\)
=>\(x=2\cdot30^0=60^0;y=3\cdot30^0=90^0;z=4\cdot30^0=120^0\)
Vậy: \(\widehat{B}=x=60^0;\widehat{C}=y=90^0;\widehat{D}=z=120^0\)