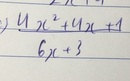
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(VT=\left(1+\frac{1}{2}+\frac{1}{3}+...+\frac{1}{2k}\right)-2\left(\frac{1}{2}+\frac{1}{4}+..+\frac{1}{2k}\right)\)
\(=\left(1+\frac{1}{2}+\frac{1}{3}+..+\frac{1}{k}\right)+\left(\frac{1}{k+1}+\frac{1}{k+2}+..+\frac{1}{2k}\right)-\left(1+\frac{1}{2}+...+\frac{1}{k}\right)=VP\)
có dpcm

\(\left(x^2+x\right)\left(x^2+x+1\right)=6\)
Đặt x^2 + x = t
\(t\left(t+1\right)=6\Leftrightarrow t^2+t=6\)
\(\Leftrightarrow t^2+t-6=0\Leftrightarrow t^2+3t-2t-6=0\)
\(\Leftrightarrow\left(t-2\right)\left(t+3\right)=0\Leftrightarrow t=2;-3\)
Sr tưởng giải PT
\(\left(x^2+x\right)\left(x^2+x+x\right)=6\)
\(\Leftrightarrow x^4+x^3+x^2+x^3+x^2+x=6\)
\(\Leftrightarrow x^4+2x^3+2x^2+x=6\)
\(\Leftrightarrow x^4+2x^3+2x^2+x-6=0\)
\(\Leftrightarrow\left(x-1\right)\left(x+2\right)\left(x^2+x+3\ne0\right)=0\Leftrightarrow x=1;-2\)

a, \(A=x\left(x+4\right)-6\left(x-1\right)\left(x+1\right)+\left(2x-1\right)^2\)
\(=x^2+4x-6\left(x^2-1\right)+4x^2-4x+1\)
\(=5x^2+1-6x^2+6=-x^2+7\)
b, Ta co :A = 3 hay \(-x^2+7=3\)
\(\Leftrightarrow-x^2=-4\Leftrightarrow x^2=4\Leftrightarrow x=\pm2\)

a, \(5x-15y=5\left(x-3y\right)\)
b, \(12y\left(2x-5y\right)+6xy\left(5-2x\right)=12y\left(2x-5\right)-6xy\left(2x-5\right)\)
\(=6y\left(2-x\right)\left(2x-5\right)\)
c, \(x^2-7x+12=x^2-3x-4x+12=\left(x-4\right)\left(x-3\right)\)

\(2x\left(x-7\right)+7-x=0\Leftrightarrow2x\left(x-7\right)-\left(x-7\right)=0\)
\(\Leftrightarrow\left(2x-1\right)\left(x-7\right)=0\Leftrightarrow x=\frac{1}{2};7\)

\(\left(x^2-1\right)^3-\left(x^4+x^2+1\right)\left(x^2-1\right)=0\)
\(\Leftrightarrow x^5-3x^4+3x^2-1-\left(x^6-x^4+x^4-x^2+x^2-1\right)=0\)
\(\Leftrightarrow x^5-3x^4+3x^2-x^6=0\)
\(\Leftrightarrow x^5\left(1-x\right)-3x^2\left(x^2-1\right)=0\Leftrightarrow x^5\left(1-x\right)-3x^2\left(x-1\right)\left(x+1\right)=0\)
\(\Leftrightarrow-x^5\left(x-1\right)-3x^2\left(x+1\right)\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left[-x^5-3x^3-3x^2\ne0\right]=0\Leftrightarrow x=1\)
4x2+4x+1/6x+3=(2x+1)2/2*(2x+1)=2x+1/2
Bai lam
\(\frac{4x^2+4x+1}{6x+3}=\frac{\left(2x+1\right)^2}{3\left(2x+1\right)}=\frac{2x+1}{3}\)
Hoc tot