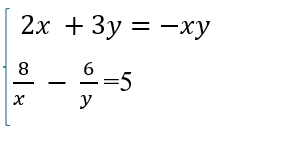 giải hệ phương trình
giải hệ phương trình
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


bài giải
số lớn chia số bé được 3 dư 3, số bé bằng 1/3 số lớn
số lớn là:
33 : ( 3 -1 ) x 3 + 3 = 53,5
số bé là:
33: ( 3 - 1 ) = 16,5
đ/s

Gọi chiều dài là a => chiều rộng = a-4
Theo bài ra: a+(a-4)=80
<=> 2a = 84
=> a = 42
=> chiều rộng = a-4 = 42-4 = 38
Diện tích tấm màn là: 38x42= 1596 m2

\(\left\{{}\begin{matrix}\dfrac{8}{\sqrt{x^2+1}}+\dfrac{4}{\sqrt{y^2+1}}=9\\\dfrac{1}{\sqrt{x^2+1}}-\dfrac{1}{\sqrt{y^2+1}}=\dfrac{3}{4}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{8}{\sqrt{x^2+1}}+\dfrac{4}{\sqrt{y^2+1}}=9\\\dfrac{4}{\sqrt{x^2+1}}-\dfrac{4}{\sqrt{y^2+1}}=3\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\dfrac{8}{\sqrt{x^2+1}}+\dfrac{4}{\sqrt{y^2+1}}+\dfrac{4}{\sqrt{x^2+1}}-\dfrac{4}{\sqrt{y^2+1}}=9+3\\\dfrac{1}{\sqrt{x^2+1}}-\dfrac{1}{\sqrt{y^2+1}}=\dfrac{3}{4}\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\dfrac{12}{\sqrt{x^2+1}}=12\\\dfrac{1}{\sqrt{y^2+1}}=1-\dfrac{3}{4}=\dfrac{1}{4}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x^2+1=1\\y^2+1=16\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x^2=0\\y^2=15\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=0\\y=\pm\sqrt{15}\end{matrix}\right.\)

TH1: x<1
Phương trình sẽ trở thành:
\(1-x+2-x+3-x=-4x\)
=>-4x=-3x+6
=>-x=6
=>x=-6(nhận)
TH2: 1<=x<2
Phương trình sẽ trở thành:
\(x-1+2-x+3-x=-4x\)
=>-4x=-x+4
=>-3x=4
=>\(x=-\dfrac{4}{3}\left(loại\right)\)
TH3: 2<=x<3
Phương trình sẽ trở thành:
\(x-1+x-2+3-x=-4x\)
=>x=-4x
=>x=0(loại)
TH4: x>=3
Phương trình sẽ trở thành:
x-1+x-2+x-3=-4x
=>-4x=3x-6
=>-7x=-6
=>\(x=\dfrac{6}{7}\left(loại\right)\)

\(170=17\cdot2\cdot5;290=29\cdot2\cdot5\)
=>\(BCNN\left(170;290\right)=17\cdot29\cdot2\cdot5=4930\)
\(a⋮170;a⋮290\)
=>\(a\in BC\left(170;290\right)\)
mà a nhỏ nhất
nên a=BCNN(170;290)
=>a=4930

a: Oy nằm giữa Ox và Ot
=>\(\widehat{xOy}+\widehat{yOt}=\widehat{xOt}\)
=>\(\widehat{yOt}+30^0=50^0\)
=>\(\widehat{yOt}=20^0\)
b: Vì Ox là tia đối của tia Oz nên \(\widehat{xOz}=180^0\)

\(\left(2,5x-\dfrac{4}{7}\right):\dfrac{8}{21}=-1,5\)
=>\(\left(\dfrac{5}{2}x-\dfrac{4}{7}\right):\dfrac{8}{21}=-\dfrac{3}{2}\)
=>\(\dfrac{5}{2}x-\dfrac{4}{7}=\dfrac{-3}{2}\cdot\dfrac{8}{21}=\dfrac{-24}{42}=\dfrac{-4}{7}\)
=>\(\dfrac{5}{2}x=-\dfrac{4}{7}+\dfrac{4}{7}=0\)
=>x=0

\(\left\{{}\begin{matrix}2x+3y=-xy\\\dfrac{8}{x}-\dfrac{6}{y}=5\end{matrix}\right.\left(x;y\ne0\right)\Leftrightarrow\left\{{}\begin{matrix}2x+3y=-xy\\8y-6x=5xy\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}2x+3y=-xy\\6x-8y=-5xy\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2x+3y=-xy\\6x-8y=5\left(2x+3y\right)\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}2x+3y=-xy\\6x-8y=10x+15y\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2x+3y=-xy\\-4x=23y\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}2\cdot\dfrac{-23}{4}y+3y=\dfrac{-23}{4}y\cdot y\\x=\dfrac{-23}{4}y\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}23y^2-43y=0\\x=\dfrac{-23}{4}y\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}y\left(23y-43\right)=0\\x=\dfrac{-23}{4}y\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}y=0\left(ktm\right)\\y=\dfrac{43}{23}\left(tm\right)\end{matrix}\right.\\x=\dfrac{-23}{4}\cdot\dfrac{43}{23}=\dfrac{-43}{4}\end{matrix}\right.\)