
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(E=\frac{\sqrt{15}-\sqrt{12}}{\sqrt{5}-2}-\frac{1}{2-\sqrt{3}}=\frac{\sqrt{3}\left(\sqrt{5}-2\right)}{\sqrt{5}-2}-\frac{1}{2-\sqrt{3}}\)
\(=\sqrt{3}-\frac{2+\sqrt{3}}{4-3}=\sqrt{3}-2-\sqrt{3}=-2< 5^{\sqrt{74}}\)
hay Vậy \(E< G\)


ĐK: \(x\ne-1;y\ne-1\)
Ta có: \(x=\frac{1}{2+\sqrt{3}}=\frac{2-\sqrt{3}}{4-3}=2-\sqrt{3}\left(tm\right)\)
\(y=\frac{1}{2-\sqrt{3}}=\frac{2+\sqrt{3}}{4-3}=2+\sqrt{3}\left(tm\right)\)
Thay \(x=2-\sqrt{3};y=2+\sqrt{3}\) vào biểu thức ta có:
\(\frac{1}{2-\sqrt{3}+1}+\frac{1}{2+\sqrt{3}+1}=\frac{1}{3-\sqrt{3}}+\frac{1}{3+\sqrt{3}}=\frac{3+\sqrt{3}+3-\sqrt{3}}{9-3}=\frac{6}{6}=1\)
Vậy ....
\(\frac{1}{x+1}+\frac{1}{y+1}=\frac{1}{\frac{1}{2+\sqrt{3}}+1}+\frac{1}{\frac{1}{2-\sqrt{3}}+1}\)
\(=\frac{1}{\frac{1+2+\sqrt{3}}{2+\sqrt{3}}}+\frac{1}{\frac{1+2-\sqrt{3}}{2-\sqrt{3}}}\)
\(=\frac{2+\sqrt{3}}{3+\sqrt{3}}+\frac{2-\sqrt{3}}{3-\sqrt{3}}\)
\(=\frac{\left(2+\sqrt{3}\right)\left(\sqrt{3}-1\right)+\left(2-\sqrt{3}\right)\left(\sqrt{3}+1\right)}{\sqrt{3}\left(\sqrt{3}+1\right)\left(\sqrt{3}-1\right)}\)
\(=\frac{2\sqrt{3}}{2\sqrt{3}}=1\)

Ta có : \(n^2-6n+5=\left(n-5\right)\left(n-1\right)\)(*)
Để (*) là số nguyên tố khi \(n-5=1\)và \(n-1\)là số nguyên tố
\(\Leftrightarrow n=6\left(tm\right)\)
Vậy n = 6 thì (*) là số nguyên tố

Ta có: \(\frac{a}{\sqrt{a^2-b^2}}-\left(1+\frac{a}{\sqrt{a^2-b^2}}\right)\div\frac{b}{a-\sqrt{a^2-b^2}}\)
\(=\frac{a}{\sqrt{a^2-b^2}}-\frac{a+\sqrt{a^2-b^2}}{\sqrt{a^2-b^2}}\cdot\frac{a-\sqrt{a^2-b^2}}{b}\)
\(=\frac{a}{\sqrt{a^2-b^2}}-\frac{a^2-a^2+b^2}{b\sqrt{a^2-b^2}}\)
\(=\frac{a}{\sqrt{a^2-b^2}}-\frac{b^2}{b\sqrt{a^2-b^2}}\)
\(=\frac{a-b}{\sqrt{a^2-b^2}}=\frac{a-b}{\sqrt{\left(a-b\right)\left(a+b\right)}}=\sqrt{\frac{a-b}{a+b}}\)

Với \(x>0;x\ne4\)
\(\left(\frac{2}{\sqrt{x}-2}+\frac{3}{2\sqrt{x}+1}-\frac{5\sqrt{x}-7}{2x-3\sqrt{x}-2}\right):\frac{2\sqrt{x}+3}{5x-10\sqrt{x}}\)
\(=\left(\frac{2\left(2\sqrt{x}+1\right)+3\left(\sqrt{x}-2\right)-5\sqrt{x}+7}{\left(2\sqrt{x}+1\right)\left(\sqrt{x}-2\right)}\right):\frac{2\sqrt{x}+3}{5\sqrt{x}\left(\sqrt{x}-2\right)}\)
\(=\left(\frac{4\sqrt{x}+2+3\sqrt{x}-6-5\sqrt{x}+7}{\left(2\sqrt{x}+1\right)\left(\sqrt{x}-2\right)}\right):\frac{2\sqrt{x}+3}{5\sqrt{x}\left(\sqrt{x}-2\right)}\)
\(=\left(\frac{2\sqrt{x}+3}{\left(2\sqrt{x}+1\right)\left(\sqrt{x}-2\right)}\right).\frac{5\sqrt{x}\left(\sqrt{x}-2\right)}{2\sqrt{x}+3}=\frac{5\sqrt{x}}{2\sqrt{x}+1}\)
\(A=\left[\frac{2\left(2\sqrt{x}+1\right)}{\left(\sqrt{x}-2\right)\left(2\sqrt{x}+1\right)}+\frac{3\left(\sqrt{x}-2\right)}{\left(\sqrt{x}-2\right)\left(2\sqrt{x}+1\right)}-\frac{5\sqrt{x}-7}{\left(\sqrt{x}-2\right)\left(2\sqrt{x}+1\right)}\right]\times\frac{5\sqrt{x}\left(\sqrt{x}-2\right)}{2\sqrt{x}+3}\)
\(=\frac{4\sqrt{x}+2+3\sqrt{x}-6-5\sqrt{x}+7}{\left(\sqrt{x}-2\right)\left(2\sqrt{x}+1\right)}\times\frac{5\sqrt{x}\left(\sqrt{x}-2\right)}{2\sqrt{x}+3}\)
\(=\frac{5\sqrt{x}\left(2\sqrt{x}+3\right)\left(\sqrt{x}-2\right)}{\left(\sqrt{x}-2\right)\left(2\sqrt{x}+1\right)\left(2\sqrt{x}+3\right)}=\frac{5\sqrt{x}}{2\sqrt{x}+1}\)

Đề bạn ghi có vẻ sai.
Khi cho \(a,b\)dương càng nhỏ thì \(\frac{1}{a}+\frac{1}{b}\)đạt giá trị càng lớn nên \(\frac{1}{a}+\frac{1}{b}\)không có giá trị lớn nhất.
Sửa đề. Tìm GTNN.
Ta có: \(\frac{1}{a}+\frac{1}{b}\ge\frac{4}{a+b}\ge\frac{4}{2\sqrt{2}}=\sqrt{2}\)
Dấu \(=\)khi \(\hept{\begin{cases}\frac{1}{a}=\frac{1}{b}\\a+b=2\sqrt{2}\end{cases}}\Leftrightarrow a=b=\sqrt{2}\).
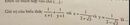

\(P=\frac{x\sqrt{x}-1}{1+x+\sqrt{x}}\cdot\left(\frac{\sqrt{x}+1}{x-1}-\frac{\sqrt{x}-2}{x-\sqrt{x}-2}\right)\)
\(P=\frac{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}{x+\sqrt{x}+1}\cdot\left(\frac{\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}-\frac{\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+1\right)}\right)\)
\(P=\left(\sqrt{x}-1\right)\cdot\left(\frac{1}{\sqrt{x}-1}-\frac{1}{\sqrt{x}+1}\right)\)
\(P=\left(\sqrt{x}-1\right)\cdot\left(\frac{\sqrt{x}+1-\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\right)=\left(\sqrt{x}-1\right)\cdot\frac{2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}=\frac{2}{\sqrt{x}+1}\)
\(P=\frac{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}{x+\sqrt{x}+1}.\left(\frac{\sqrt{x}+1}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}-\frac{\sqrt{x}-2}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-2\right)}\right)\)
\(P=\left(\sqrt{x}-1\right).\left(\frac{1}{\sqrt{x}-1}-\frac{1}{\sqrt{x}+1}\right)\)
\(P=\left(\sqrt{x}-1\right).\left(\frac{\sqrt{x}+1-\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\right)\)
\(P=\left(\sqrt{x}-1\right).\frac{2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(P=\frac{2}{\sqrt{x}+1}\)