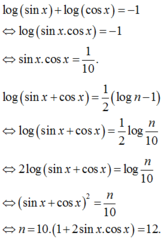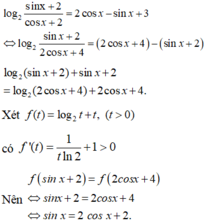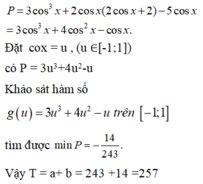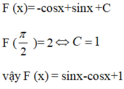Cho các số thực x,y thay đổi thỏa mãn . Gọi - tối giản là giá trị nhỏ nhất của biểu thức P= . Tính T=a+b.
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

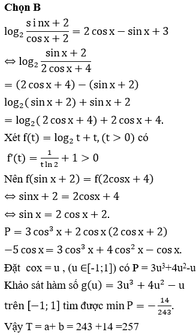

2.1
a.
\(\Leftrightarrow sinx-cosx=\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow\sqrt{2}sin\left(x-\dfrac{\pi}{4}\right)=\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow sin\left(x-\dfrac{\pi}{4}\right)=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x-\dfrac{\pi}{4}=\dfrac{\pi}{6}+k2\pi\\x-\dfrac{\pi}{4}=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5\pi}{12}+k2\pi\\x=\dfrac{13\pi}{12}+k2\pi\end{matrix}\right.\)
b.
\(cosx-\sqrt{3}sinx=1\)
\(\Leftrightarrow\dfrac{1}{2}cosx-\dfrac{\sqrt{3}}{2}sinx=\dfrac{1}{2}\)
\(\Leftrightarrow cos\left(x+\dfrac{\pi}{3}\right)=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x+\dfrac{\pi}{3}=\dfrac{\pi}{3}+k2\pi\\x+\dfrac{\pi}{3}=-\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=k2\pi\\x=-\dfrac{2\pi}{3}+k2\pi\end{matrix}\right.\)

Đặt t = sin x − cos x = 2 sin x − π 4 .
Điều kiện − 2 ≤ t ≤ 2 .
Ta có t 2 = sin x − cos x 2 = sin 2 x + cos 2 x − 2 sin x cos x ⇒ sin 2 x = 1 − t 2 .
Phương trình đã cho trở thành 1 − t 2 + t = 1 ⇔ t 2 − t = 0 ⇔ t = 0 t = 1 .
Với t = 1, ta được 2 sin x − π 4 = 1 ⇔ sin x − π 4 = 1 2 .
Với t = 0, ta được 2 sin x − π 4 = 0 ⇔ sin x − π 4 = 0.
Chọn đáp án B.

Chọn B
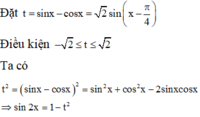

Bổ trợ kiến thức: Ta có thế giải bằng máy tính cầm tay CASIO fx-570VN PLUS như sau, đâu tiên dùng lệnh SHIFT SOLVE để xem 1 nghiệm bất kì có thể có của phương trình đã cho:
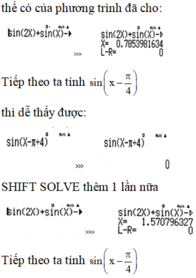

Đến đây ta dễ dàng chọn được phương án B là phương án đúng thay cho lời giải tự luận nhiều phức tạp.

\(\frac{sinx}{1+cosx}+\frac{1+cosx}{sinx}=\frac{sin^2x+\left(1+cosx\right)^2}{sinx\left(1+cosx\right)}=\frac{sin^2x+cos^2x+2cosx+1}{sinx\left(1+cosx\right)}\)
\(=\frac{2+2cosx}{sinx\left(1+cosx\right)}=\frac{2\left(1+cosx\right)}{sinx\left(1+cosx\right)}=\frac{2}{sinx}\)
\(\frac{cosx}{1-sinx}=\frac{cos2.\frac{x}{2}}{1-sin2.\frac{x}{2}}=\frac{cos^2\frac{x}{2}-sin^2\frac{x}{2}}{sin^2\frac{x}{2}+cos^2\frac{x}{2}-2sin\frac{x}{2}.cos\frac{x}{2}}=\frac{\left(cos\frac{x}{2}-sin\frac{x}{2}\right)\left(cos\frac{x}{2}+sin\frac{x}{2}\right)}{\left(cos\frac{x}{2}-sin\frac{x}{2}\right)^2}\)
\(=\frac{sin\frac{x}{2}+cos\frac{x}{2}}{cos\frac{x}{2}-sin\frac{x}{2}}=\frac{\sqrt{2}cos\left(\frac{\pi}{4}-\frac{x}{2}\right)}{\sqrt{2}sin\left(\frac{\pi}{4}-\frac{x}{2}\right)}=cot\left(\frac{\pi}{4}-\frac{x}{2}\right)\)
@Nguyễn Việt Lâm cho mình hỏi dấu = thứ 2 từ cuối bài 2 đếm lên sao r đc như v