Cho bất phương trình
3
+
x
+
6
-
x
-
18
+
3
x
-
x
2
≤
m
2
-
m
+
1
(m là tham số). Có bao nhiêu giá trị nguyên của m thuộc[-5;5] để bất phương trình...
Đọc tiếp
Cho bất phương trình 3 + x + 6 - x - 18 + 3 x - x 2 ≤ m 2 - m + 1 (m là tham số). Có bao nhiêu giá trị nguyên của m thuộc[-5;5] để bất phương trình nghiệm đúng với mọi x ∈ - 3 ; 6 ?
A. 3
B. 5
C. 9
D. 10
Đặt
Suy ra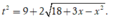
Ta có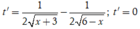
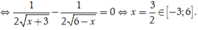
Ta có bảng biến thiên
Từ bảng biến thiên ta suy ra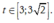
Khi đó bất phương trình trở thành: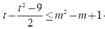
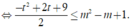
Xét hàm số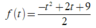
với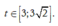 
Ta có
Suy ra hàm số f(t) nghịch biến trên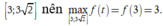
Chọn C.