
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Các câu hỏi dưới đây có thể giống với câu hỏi trên
UN
7

TC
4

2 tháng 3 2017
Ta có: \(\left|x-1\right|+\left|x-5\right|=\left|x-1\right|+\left|5-x\right|\)
Nhận thấy: \(\left[{}\begin{matrix}\left|x-1\right|\ge x-1\\\left|5-x\right|\ge5-x\end{matrix}\right.\)
\(\Rightarrow\left|x-1\right|+\left|5-x\right|\ge x-1+5-x\)
\(\Rightarrow\left|x-1\right|+\left|5-x\right|\ge4\)
Dấu \("="\) xảy ra khi:
\(\left[{}\begin{matrix}x-1\ge0\\5-x\ge0\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x\ge1\\x\le5\end{matrix}\right.\) \(\Rightarrow1\le x\le5\)
Vậy \(1\le x\le5.\)
2 tháng 3 2017
Cho mk thêm cái ạ:
\(x\in\left\{1;2;3;4;5\right\}\)
Vậy \(x\in\left\{1;2;3;4;5\right\}\)

DN
12 tháng 5 2017
| Giá trị (x) | Tần số (n) | Các tích (x.n) | |
| 17 | 3 | 51 | |
| 18 | 5 | 90 | |
| 19 | 4 | 76 | |
| 20 | 2 | 40 | |
| 21 | 3 | 63 | |
| 22 | 2 | 44 | |
| 24 | 3 | 72 | |
| 26 | 3 | 78 | |
| 28 | 1 | 28 | |
| 30 | 1 | 30 | |
| 31 | 2 | 62 | |
| 32 | 1 | 32 | |
| N = 30 | Tổng: 666 |

25 tháng 10 2017
| Phép tính | Ước lương kết quả | ĐS đúng |
| 24.68:12 | 20.70:10 = 140 | 136 |
| 7,8.3,1:1,6 | 8.3:2=12 | 15,1125 |
| 6,9.72:24 | 7.70:20 = 24,5 | 20,7 |
| 56.9,9:8,8 | 60.10:9 = 66,(6) | 63 |
| 0,38.0,45:0,95 | 0.0:1=0 | 0,18 |




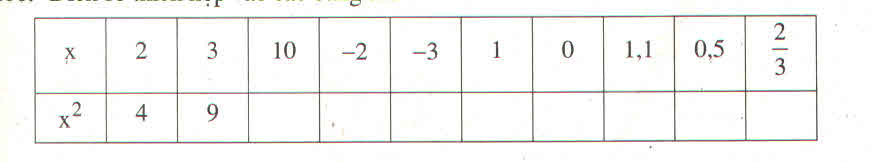
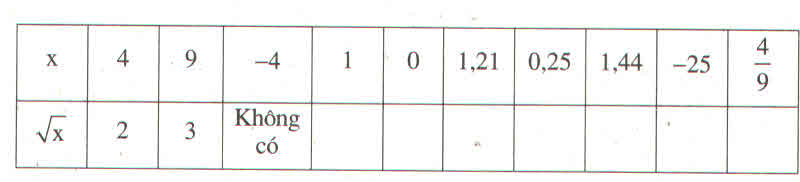







Bài 2:
a.
$P=M+N=-xy^2+3x^2y-x^2y^2+\frac{1}{2}x^2y-xy^2+\frac{-2}{3}x^2y^2$
$=(-xy^2-xy^2)+(3x^2y+\frac{1}{2}x^2y)+(-x^2y^2+\frac{-2}{3}x^2y^2)$
$=-2xy^2+\frac{7}{2}x^2y-\frac{5}{3}x^2y^2$
b.
$Q=N-M=(\frac{1}{2}x^2y-xy^2+\frac{-2}{3}x^2y^2)-(-xy^2+3x^2y-x^2y^2)$
$=(\frac{1}{2}x^2y-3x^2y)-xy^2+xy^2+(\frac{-2}{3}x^2y^2+x^2y^2)$
$=\frac{-5}{2}x^2y+\frac{1}{3}x^2y^2$
c.
$Q=\frac{-5}{2}(-1)^2.\frac{1}{2}+\frac{1}{3}(-1)^2.(\frac{1}{2})^2=\frac{-7}{6}$
Bài 3:
a.
$A(x)=\frac{1}{3}x^2-2x^3+2x-\frac{4}{3}x^2-x-1$
$=-2x^3-x^2+x-1$
$A(x)$ có hệ số cao nhất là $-2$ và hệ số tự do là $-1$
$B(x)=2x^3+x^2+1$
$B(x)$ có hệ số cao nhất là $2$ và hệ số tự do là $1$
b.
$B(x)=(2x^3+2x^2)-(x^2-1)=2x^2(x+1)-(x-1)(x+1)$
$=(x+1)(2x^2-x+1)$
$B(-1)=(-1+1)(2x^2-x+1)=0$ nên $-1$ là nghiệm của $B(x)$
c.
$C(x)=A(x)+B(x)=-2x^3-x^2+x-1+(2x^3+x^2+1)$
$=x$
d.
$C(x)=0\Leftrightarrow x=0$
Vậy $x=0$ là nghiệm của $C(x)$