Giúp mik Câu 3.2 với ạ
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


1.2 với \(x\ge0,x\in Z\)
A=\(\dfrac{2\sqrt{x}+7}{\sqrt{x}+2}=2+\dfrac{3}{\sqrt{x}+2}\in Z< =>\sqrt{x}+2\inƯ\left(3\right)=\left(\pm1;\pm3\right)\)
*\(\sqrt{x}+2=1=>\sqrt{x}=-1\)(vô lí)
*\(\sqrt{x}+2=-1=>\sqrt{x}=-3\)(vô lí
*\(\sqrt{x}+2=3=>x=1\)(TM)
*\(\sqrt{x}+2=-3=\sqrt{x}=-5\)(vô lí)
vậy x=1 thì A\(\in Z\)

Bài 1.2
\(A=\dfrac{2\sqrt{x}+7}{\sqrt{x}+2}=2+\dfrac{3}{\sqrt{x}+2}\)
C1:Bạn dùng pp chặn như bài 2.2
C2: (Gợi ý)\(\sqrt{x}+2\ge2\) và \(\sqrt{x}+2\inƯ\left(3\right)\)\(\Rightarrow\sqrt{x}+2=3\Leftrightarrow x=1\)
Vậy x=1 thì A nguyên
Bài 2.2
\(A=\dfrac{\sqrt{x}+7}{\sqrt{x}+2}=1+\dfrac{5}{\sqrt{x}+2}\)
Do \(\sqrt{x}\ge0;\forall x\)\(\Rightarrow\sqrt{x}+2\ge2\) \(\Rightarrow\dfrac{5}{\sqrt{x}+2}\le\dfrac{5}{2}\)\(\Rightarrow A\le\dfrac{7}{2}\) (1)
mà \(\dfrac{5}{\sqrt{x}+2}>0;\forall x\Rightarrow A>1\) (2)
Từ (1) (2) \(\Rightarrow1< A\le\dfrac{7}{2}\) mà A nguyên
\(\Rightarrow\left[{}\begin{matrix}A=2\\A=3\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}1+\dfrac{5}{\sqrt{x}+2}=2\\1+\dfrac{5}{\sqrt{x}+2}=3\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{x}+2=5\\\sqrt{x}+2=\dfrac{5}{2}\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{x}=3\\\sqrt{x}=\dfrac{1}{2}\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=9\\x=\dfrac{1}{4}\end{matrix}\right.\)
Vậy...
Bài 3.2
\(A=\dfrac{-x-2\sqrt{x}-5}{\sqrt{x}+2}\)\(=\dfrac{-\sqrt{x}\left(\sqrt{x}+2\right)-5}{\sqrt{x}+2}=-\sqrt{x}-\dfrac{5}{\sqrt{x}+2}\)
\(=2-\left(\sqrt{x}+2+\dfrac{5}{\sqrt{x}+2}\right)\)
Áp dụng bđt cosi: \(\sqrt{x}+2+\dfrac{5}{\sqrt{x}+2}\ge2\sqrt{\left(\sqrt{x}+2\right).\dfrac{5}{\sqrt{x}+2}}=2\sqrt{5}\)
\(\Rightarrow A\le2-2\sqrt{5}\)
Dấu = xảy ra \(\Leftrightarrow\sqrt{x}+2=\dfrac{5}{\sqrt{x}+2}\Leftrightarrow x=9-4\sqrt{5}\)

3.2:
Theo vi ét: \(\left\{{}\begin{matrix}x_1+x_2=2m+2\\x_1x_2=m^2+m\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left(x_1+x_2\right)^2=\left(2m+2\right)^2=4m^2+8m+4\\4x_1x_2=4m^2+4m\end{matrix}\right.\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-4x_1x_2=4m+4=2\left(2m+2\right)=2\left(x_1+x_2\right)\)
\(\Rightarrow\left(x_1+x_2\right)^2-4x_1x_2-2\left(x_1+x_2\right)=4m^2+8m+4-4m^2-4m-4m-4=0\)
Vậy hệ thức liên hệ giữa \(x_1\) và \(x_2\) mà không phụ thuộc vào tham số m là \(\left(x_1+x_2\right)^2-4x_1x_2-2\left(x_1+x_2\right)\)
2: x1+x2=2m+2; x1x2=m^2+m
(x1+x2)^2-4x1x2
=4m^2+8m+4-4m^2-4m=4m+4
=>(x1+x2)^2-4x1x2-2(x1+x2)=4m+4-4m-4=0 ko phụ thuộc m

Câu 3:
a: \(BD=\sqrt{BC^2-DC^2}=4\left(cm\right)\)
b: \(\widehat{A}=180^0-2\cdot70^0=40^0< \widehat{B}\)
nên BC<AC=AB
c: Xét ΔEBC vuông tại E và ΔDCB vuông tại D có
BC chung
\(\widehat{EBC}=\widehat{DCB}\)
Do đó:ΔEBC=ΔDCB
d: Xét ΔOBC có \(\widehat{OBC}=\widehat{OCB}\)
nên ΔOBC cân tại O
Câu 2
a) Thay y = -2 vào biểu thức đã cho ta được:
2.(-2) + 3 = -1
Vậy giá trị của biểu thức đã cho tại y = -2 là -1
b) Thay x = -5 vào biểu thức đã cho ta được:
2.[(-5)² - 5] = 2.(25 - 5) = 2.20 = 40
Vậy giá trị của biểu thức đã cho tại x = -5 là 40

3.2
\(\Delta'=\left(a+1\right)^2-2a=a^2+1>0;\forall a\Rightarrow\) pt luôn có 2 nghiệm pb với mọi a
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2\left(a+1\right)\\x_1x_2=2a\end{matrix}\right.\)
Do \(x_1\) là nghiệm nên: \(x_1^2-2\left(a+1\right)x_1+2a=0\Rightarrow x_1^2=2\left(a+1\right)x_1-2a\)
Thay vào bài toán:
\(2\left(a+1\right)x_1-2a+x_1-x_2=3-2a\)
\(\Leftrightarrow\left(2a+3\right)x_1-x_2=3\)
\(\Rightarrow x_2=\left(2a+3\right)x_1-3\)
Thế vào \(x_1+x_2=2\left(a+1\right)\)
\(\Rightarrow x_1+\left(2a+3\right)x_1-3=2\left(a+1\right)\)
\(\Rightarrow\left(2a+4\right)x_1=2a+5\Rightarrow x_1=\dfrac{2a+5}{2a+4}\Rightarrow x_2=2a+2-\dfrac{2a+5}{2a+4}=\dfrac{4a^2+10a+3}{2a+4}\) (\(a\ne-2\))
Thế vào \(x_1x_2=2a\)
\(\Rightarrow\dfrac{\left(2a+5\right)\left(4a^2+10a+3\right)}{\left(2a+4\right)^2}=2a\)
\(\Rightarrow8a^2+24a+15=0\Rightarrow a=...\)

a: \(=\dfrac{x^3+2x+2x-2-x^2-x-1}{\left(x-1\right)\left(x^2+x+1\right)}\)
\(=\dfrac{x^3-x^2+3x-3}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{x^2+3}{x^2+x+1}\)
b: \(=\dfrac{x^2-2x-3+x^2+2x-3+2x-2x^2}{\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{2x-6}{\left(x-3\right)\left(x+3\right)}=\dfrac{2}{x+3}\)
c: \(=\dfrac{6-7+x}{3\left(x-1\right)}=\dfrac{x-1}{3\left(x-1\right)}=\dfrac{1}{3}\)
d: \(=\dfrac{x^3+2x+2x-2-x^2-x-1}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{x^3-x^2+3x-3}{\left(x-1\right)\left(x^2+x+1\right)}=\dfrac{x^2+3}{x^2+x+1}\)






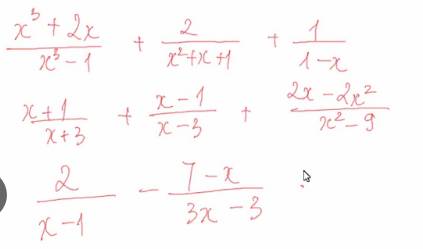
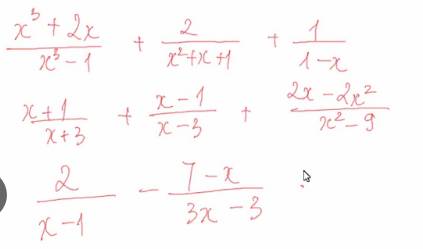
Có \(ac=1.\left(-2\right)=-2\)<0
=>Pt luôn có hai nghiệm pb trái dấu
Theo viet: \(\left\{{}\begin{matrix}x_1+x_2=m-1\\x_1x_2=-2\end{matrix}\right.\)
Do x1;x2 là hai nghiệm của pt \(\Rightarrow\left\{{}\begin{matrix}x_1^2-3=\left(m-1\right)x_1-1\\x_2^2-3=\left(m-1\right)x_2-1\end{matrix}\right.\)
Có \(\dfrac{x_1}{x_2}=\dfrac{x_2^2-3}{x_1^2-3}\)(đk: \(x^2\ne3\) thay vào pt ban đầu => \(m\ne\dfrac{3+\sqrt{3}}{3}\))
\(\Rightarrow x_1\left(x_1^2-3\right)=x_2\left(x_2^2-3\right)\)
\(\Leftrightarrow x_1\left[\left(m-1\right)x_1-1\right]=x_2\left[\left(m-1\right)x_2-1\right]\)
\(\Leftrightarrow x_1^2\left(m-1\right)-x_1=x_2^2\left(m-1\right)-x_2\)
\(\Leftrightarrow\left(m-1\right)\left(x_1^2-x_2^2\right)-\left(x_1-x_2\right)=0\)
\(\Leftrightarrow\left(m-1\right)\left(x_1+x_2\right)-1=0\) (vì \(x_1\ne x_2\))
\(\Leftrightarrow\left(m-1\right)^2=1\) \(\Leftrightarrow\left[{}\begin{matrix}m=0\\m=2\end{matrix}\right.\) (thỏa mãn)
Vậy...