câu này mình cần gấp
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


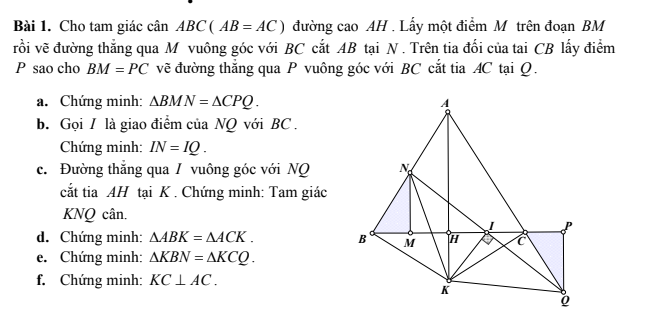
a, Vì tam giác ABC cân tại A nên \(\widehat{NBM}=\widehat{ACB}\)
Mà \(\widehat{ACB}=\widehat{PCQ}\left(đối.đỉnh\right)\Rightarrow\widehat{NBM}=\widehat{PCQ}\)
Mà \(\widehat{NMB}=\widehat{CPQ}=90^0;BM=PC\)
Do đó \(\Delta BMN=\Delta CPQ\left(g.c.g\right)\)
b, Vì \(BM//PQ\left(\perp BP\right)\) nên \(\widehat{MNI}=\widehat{IQP}\)
Mà \(\widehat{NMI}=\widehat{IPQ}=90^0;MN=PQ\left(\Delta BMN=\Delta CPQ\right)\)
Do đó \(\Delta IMN=\Delta IPQ\left(g.c.g\right)\)
\(\Rightarrow IN=IQ\)
c, Vì IK là đường cao cũng là trung tuyến tam giác KNQ nên tam giác KNQ cân tại K

uses crt;
var st:string;
d,i:integer;
begin
clrscr;
readln(st);
d:=length(st);
for i:=1 to d do
if (st[i] in ['0'..'9']) then write(st[i]);
readln;
end.


Bài 6:
-Thay \(x=1\) vào \(f\left(x\right)=x\left(x+1\right)+\left(x+1\right)\left(x+2\right)+...+\left(x+49\right)\left(x+50\right)\), ta được:
\(f\left(1\right)=1\left(1+1\right)+\left(1+1\right)\left(1+2\right)+...+\left(1+49\right)\left(1+50\right)\)
\(=1.2+2.3+...+50.51\)
\(=\dfrac{1.2.3+2.3.3+...+50.51.3}{3}\)
\(=\dfrac{1.2.3+2.3.\left(4-1\right)+...+50.51.\left(52-49\right)}{3}\)
\(=\dfrac{1.2.3+2.3.4-1.2.3+...+50.51.52-49.50.51}{3}\)
\(=\dfrac{50.51.52}{3}=44200\)

Phương trình tương đương
\(\left[{}\begin{matrix}x=\dfrac{5\pi}{12}+k\pi\\x=-\dfrac{\pi}{4}+k\pi\end{matrix}\right.,k\in Z\)
Xét họ nghiệm \(x=\dfrac{5\pi}{12}+k\pi,k\in Z\).
Do \(-\dfrac{\pi}{2}< \dfrac{5\pi}{12}+k\pi< \dfrac{8\pi}{3}\) nên \(-\dfrac{11\pi}{12}< k\pi< \dfrac{9\pi}{4}\)
⇒ \(-\dfrac{11}{12}< k< \dfrac{9}{4}\). Mà k ∈ Z nên k ∈ {0 ; 1}
Vậy các nghiệm thỏa mãn phương trình là các phần tử của tập hợp :
S1 = \(\left\{\dfrac{5\pi}{12};\dfrac{17\pi}{12}\right\}\)
Xét họ nghiệm \(x=-\dfrac{\pi}{4}+k\pi\) với k ∈ Z.
Do \(-\dfrac{\pi}{2}< \dfrac{-\pi}{4}+k\pi< \dfrac{8\pi}{3}\) nên \(-\dfrac{\pi}{4}< k\pi< \dfrac{35\pi}{12}\)
nên \(-\dfrac{1}{4}< k< \dfrac{35}{12}\). Mà k ∈ Z nên k∈ {0 ; 1 ; 2}
Vậy các nghiệm thỏa mãn phương trình là các phần tử của tập hợp
S2 = \(\left\{-\dfrac{\pi}{4};\dfrac{3\pi}{4};\dfrac{7\pi}{4}\right\}\)
Vậy các nghiệm thỏa mãn phương trình là các phần tử của tập hợp
S = S1 \(\cup\) S2 = \(\left\{\dfrac{5\pi}{12};\dfrac{17\pi}{12};-\dfrac{\pi}{4};\dfrac{3\pi}{4};\dfrac{7\pi}{4}\right\}\)

a: \(\widehat{\left(SC;\left(ABCD\right)\right)}=\widehat{CS;CA}=\widehat{SCA}\)
Ta có: SA\(\perp\)(ABCD)
=>SA\(\perp\)AC
=>ΔSAC vuông tại A
Vì ABCD là hình vuông
nên \(AC=AD\cdot\sqrt{2}=a\sqrt{2}\)
Xét ΔSAC vuông tại A có \(tanSCA=\dfrac{SA}{AC}=\dfrac{a\sqrt{6}}{a\sqrt{2}}=\sqrt{3}\)
nên \(\widehat{SCA}=60^0\)
=>\(\widehat{SC;\left(ABCD\right)}=60^0\)
b: Ta có: BD\(\perp\)AC
BD\(\perp\)SA
SA,AC cùng thuộc mp(SAC)
Do đó: BD\(\perp\)(SAC)
\(\widehat{SB;\left(SAC\right)}=\widehat{SB;SD}=\widehat{BSD}\)
Vì ABCD là hình vuông
nên \(AC=BD=a\sqrt{2}\)
ΔSAD vuông tại A
=>\(SA^2+AD^2=SD^2\)
=>\(SD^2=\left(a\sqrt{6}\right)^2+a^2=7a^2\)
=>\(SD=a\sqrt{7}\)
ΔSAB vuông tại A
=>\(SA^2+AB^2=SB^2\)
=>\(SB=a\sqrt{7}\)
Xét ΔSBD có \(cosBSD=\dfrac{SB^2+SD^2-BD^2}{2\cdot SB\cdot SD}\)
\(=\dfrac{7a^2+7a^2-2a^2}{2\cdot a\sqrt{7}\cdot a\sqrt{7}}=\dfrac{6}{7}\)
=>\(sinBSD=\sqrt{1-\left(\dfrac{6}{7}\right)^2}=\dfrac{\sqrt{13}}{7}\)
=>\(\widehat{BSD}\simeq31^0\)
=>\(\widehat{SB;\left(SAC\right)}\simeq31^0\)

\(\left(x-3\right)^{30}=\left(x-3\right)^{10}\)
\(\Leftrightarrow\left(x-3\right)\left(x-2\right)\left(x-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=3\\x=2\\x=4\end{matrix}\right.\)


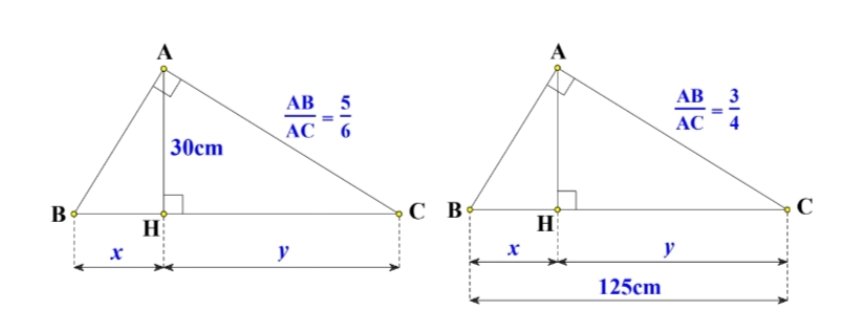
a: AB/AC=5/6
=>HB/HC=25/36
=>HB/25=HC/36=k
=>HB=25k; HC=36k
AH^2=HB*HC
=>25k*36k=30^2
=>900k^2=900
=>k=1
=>x=25cm; y=25cm
b: AB/AC=3/4
=>HB/HC=9/16
=>x/y=9/16
=>x/9=y/16=(x+y)/(9+16)=125/25=5
=>x=45cm; y=80cm

\(\left(x+1\right)\left(x^2-x+1\right)-2x=x\left(x-1\right)\left(x+1\right)\\ \Leftrightarrow x^3+1-2x=x\left(x^2-1\right)\\ \Leftrightarrow x^3+1-2x=x^3-x\\ \Leftrightarrow1-x=0\\ \Leftrightarrow x=1\)
\(d,\left(x+1\right)\left(x^2-x+1\right)-2x=x\left(x-1\right)\left(x+1\right)\)
\(\Leftrightarrow x^3+1-2x=x\left(x^2-1\right)\)
\(\Leftrightarrow x^3+1-2x=x^3-x\)
\(\Leftrightarrow x^3-x^3-2x+x+1=0\)
\(\Leftrightarrow x+1=0\)
\(\Leftrightarrow x=-1\)
Vậy \(S=\left\{-1\right\}\)
* Áp dụng hằng thẳng thức tổng hai lập phương : \(\left(x+1\right)\left(x^2-x+1\right)=x^3+1\)
* vế phải áp dụng hằng thẳng thức hiệu hai bình phương : \(x\left(x+1\right)\left(x+1\right)=x\left(x^2-1\right)=x^3-x\)






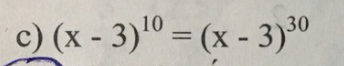 giúp mình câu này với mình đang cần gấp
giúp mình câu này với mình đang cần gấp
chim to sẻ ra ăn được đấy
Câu nào