tim x: 7-5.(x-2)=3+2.(4-x)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
13 tháng 3 2021
a)\(\sqrt{3x+1}+2x=\sqrt{x-4}-5\left(ĐKXĐ:x\ge4\right)\)
\(\Leftrightarrow\left(\sqrt{3x+1}-\sqrt{x-4}\right)+\left(2x+5\right)=0\)
\(\Leftrightarrow\frac{3x+1-x+4}{\sqrt{3x+1}+\sqrt{x-4}}+\left(2x+5\right)=0\)
\(\Leftrightarrow\frac{2x+5}{\sqrt{3x+1}+\sqrt{x-4}}+\left(2x+5\right)=0\)
\(\Leftrightarrow\left(2x+5\right)\left(\frac{1}{\sqrt{3x+1}+\sqrt{x-4}}+1\right)=0\)
13 tháng 3 2021
a') (tiếp)
\(\Leftrightarrow\orbr{\begin{cases}2x+5=0\\\frac{1}{\sqrt{3x+1}+\sqrt{x-4}}+1=0\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=-2,5\left(KTMĐKXĐ\right)\\\frac{1}{\sqrt{3x+1}+\sqrt{x-4}}+1=0\end{cases}}\)
Xét phương trình \(\frac{1}{\sqrt{3x+1}+\sqrt{x-4}}+1=0\)(1)
Với mọi \(x\ge4\), ta có:
\(\sqrt{3x+1}>0\); \(\sqrt{x-4}\ge0\)
\(\Rightarrow\sqrt{3x+1}+\sqrt{x-4}>0\Rightarrow\frac{1}{\sqrt{3x+1}+\sqrt{x-4}}>0\)
\(\Rightarrow\frac{1}{\sqrt{3x+1}+\sqrt{x-4}}+1>0\)
Do đó phương trình (1) vô nghiệm.
Vậy phương trình đã cho vô nghiệm.
TT
2
5 tháng 1 2022
-(x - 3 + 84) = (x + 70 - 68) - 5 => 0 - (x - 3 + 84) = x + 70 - 68 - 5 => 0 - x + 3 - 84 = x + 70 - 68 - 5 => 0 + (-x) + 3 + (-84) = x + 70 + (-68) + (-5) => (-x) + (-81) = x + (-3) => (-81) = x + (-3) - (-x) = x + (-3) + x = 2x + (-3) => 2x = (-81) - (-3) = -78 => x = (-78) : 2 = -39. Chúc bạn học tốt nhe (Note: chuyển vế thì bạn chú ý dấu trước số nếu đang là + thì sang vế kia phải là - và ngược lại)
17 tháng 6 2021
a) \(\sqrt{2x-3}=x-3\) (ĐK: \(x\ge\dfrac{3}{2}\))
<=> \(\left\{{}\begin{matrix}x\ge3\\2x-3=\left(x-3\right)^2\left(1\right)\end{matrix}\right.\)
(1) <=> \(2x-3=x^2-6x+9\)
<=> \(x^2-8x+12=0\)
<=> (x-2)(x-6) = 0 <=> \(\left[{}\begin{matrix}x=2\left(l\right)\\x=6\left(c\right)\end{matrix}\right.\)
KL: Phương trình có nghiệm duy nhất x = 6
b) \(\sqrt{10-x}+\sqrt{x+3}=5\) (ĐK: \(-3\le x\le10\))
<=> \(\left(\sqrt{10-x}+\sqrt{x+3}\right)^2=25\)
<=> \(10-x+x+3+2\sqrt{\left(10-x\right)\left(x+3\right)}=25\)
<=> \(\sqrt{\left(10-x\right)\left(x+3\right)}=6\)
<=> (10-x)(x+3) = 36
<=> 7x - x2 + 30 = 36
<=> x2 -7x + 6 = 0
<=> (x-1)(x-6) = 0
<=> \(\left[{}\begin{matrix}x=1\left(c\right)\\x=6\left(c\right)\end{matrix}\right.\)
KL: Phương trình có nghiệm S = {1;6}
c) \(\sqrt{x+3}-\sqrt{x-4}=1\) (ĐK: \(x\ge4\))
<=> \(\sqrt{x+3}=\sqrt{x-4}+1\)
<=> \(x+3=x-4+1+2\sqrt{x-4}\)
<=> \(\sqrt{x-4}=3\)
<=> x-4 = 9 <=> x = 13 (c)
KL: Phương trình có nghiệm duy nhất x = 13
TA
17 tháng 6 2021
a) ĐK: `x≥3`
`\sqrt(2x-3)=x-3`
`<=>2x-3=(x-3)^2`
`<=>2x-3=x^2-6x+9`
`<=>x^2-8x+12=0`
`<=>` \(\left[{}\begin{matrix}x=6\left(TM\right)\\x=2\left(L\right)\end{matrix}\right.\)
Vậy `x=2`.
b) ĐK: `-3<=x<=10`
`\sqrt(10-x)+\sqrt(x-3)=5`
`<=>10-x+x-3+2\sqrt((10-x)(x-3))=25`
`<=>2\sqrt((10-x)(x-3))=18`
`<=>\sqrt((10-x)(x-3))=9`
`<=>(10-x)(x-3)=81`
`<=>-x^2+13x-30=81`
`<=>x^2-13x+111=0` (VN)
KK
9 tháng 1 2019
a) x - (-3) + 5 = -8 c) |x| - 5 = 0
=> x + 3 + 5 = -8 => |x| = 5
=> x + 8 = -8 => \(\orbr{\begin{cases}x=5\\x=-5\end{cases}}\)
=> x = -8 - 8 Vậy ...
=> x = -16
b) 20 - (3 - x) = 2x - 4 d) |5 - x| + (-4) = 8
=> 20 - 3 + x = 2x - 4 => |5 - x| = 8 + 4
=> 17 + x = 2x - 4 => |5 - x| = 12
=> 17 + 4 = 2x - x => \(\orbr{\begin{cases}5-x=12\\5-x=-12\end{cases}}\)
=> x = 21 => \(\orbr{\begin{cases}x=-7\\x=17\end{cases}}\)
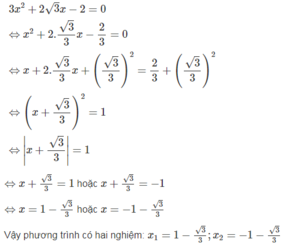
7 - 5.(\(x-2\)) = 3 + 2.(4 - \(x\))
7 - 5\(x\) + 10 = 3 + 8 - 2\(x\)
- 5\(x\) + 2\(x\) = 3 + 8 - 7 - 1
- 3\(x\) = 11 - 7 - 10
- 3\(x\) = 4 - 10
- 3\(x\) = - 6
\(x=-6:\left(-3\right)\)
\(x\) = 2