(x+3)/2021 + (x+2)/2020 + (x+3)/2013 =-3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
7 tháng 9 2021
a: \(A=\left(2x-5\right)^2-4x\left(x-5\right)\)
\(=4x^2-20x+25-4x^2+20x\)
=25
b: \(B=\left(4-3x\right)\left(4+3x\right)+\left(3x+1\right)^2\)
\(=16-9x^2+9x^2+6x+1\)
=6x+17
c: \(C=\left(x+1\right)^3-x\left(x^2+3x+3\right)\)
\(=x^3+3x^2+3x+1-x^3-3x^2-3x\)
=1
d: \(D=\left(2021x-2020\right)^2-2\left(2021x-2020\right)\left(2020x-2021\right)+\left(2020x-2021\right)^2\)
\(=\left(2021x-2020-2020x+2021\right)^2\)
\(=\left(x+1\right)^2\)
\(=x^2+2x+1\)
PH
3
NM
Nguyễn Minh Quang
Giáo viên
22 tháng 10 2021
Dễ thấy A chia hết cho 10 nên A có tận cùng là 0
còn 1x 3 x 5 x... x 2021 là một số lẻ và chia hết cho 5 nên có tận cùng là 5
H
23 tháng 3 2023
thêm \(\dfrac{1}{2021}+\dfrac{1}{2020}+\dfrac{1}{2019}+\dfrac{1}{2}\ne0\) nên nó z nha :Đ giải thích ấy
NH
23 tháng 3 2023
`<=>(x+1)/2021+1+(x+2)/2020+1+(x+3)/2019+1+(x+2028)/2-3=0`
`<=>(x+2022)/2021+(x+2022)/2020+(x+2022)/2019+(x+2022)/2=0`
`<=>(x+2022)(1/2021+1/2020+1/2019+1/2)=0`
`<=>x+2022=0`
`<=>x=-2022`
BL
0
BL
1
27 tháng 1 2022
\(=2021\cdot2\cdot\left(1+\dfrac{1}{2}:\dfrac{3}{2}-\dfrac{4}{3}\right)=4042\cdot\left(1+\dfrac{1}{3}-\dfrac{4}{3}\right)=0\)
25 tháng 2 2023
\(\dfrac{x-4}{2022}+\dfrac{x-3}{2021}+\dfrac{x-2}{2020}+\dfrac{x-1}{2019}\text{=}-4\)
\(\dfrac{x-4}{2022}+\dfrac{x-3}{2021}+\dfrac{x-2}{2020}+\dfrac{x-1}{2019}+4\text{=}0\)
\(\left(\dfrac{x-4}{2022}+1\right)+\left(\dfrac{x-3}{2021}+1\right)+\left(\dfrac{x-2}{2020}+1\right)+\left(\dfrac{x-1}{2019}+1\right)\text{=}0\)
\(\dfrac{x-2018}{2022}+\dfrac{x-2018}{2021}+\dfrac{x-2018}{2020}+\dfrac{x-2018}{2019}\text{=}0\)
\(\left(x-2018\right)\left(\dfrac{1}{2022}+\dfrac{1}{2021}+\dfrac{1}{2020}+\dfrac{1}{2019}\right)\text{=}0\)
\(Do:\) \(\dfrac{1}{2022}+\dfrac{1}{2021}+\dfrac{1}{2020}+\dfrac{1}{2019}\ne0\)
\(x-2018\text{=}0\)
\(x\text{=}2018\)
\(Vậy...\)
I
5 tháng 1 2023
\(\dfrac{x+1}{2023}+\dfrac{x+2}{2022}=\dfrac{x+3}{2021}+\dfrac{x+4}{2020}\\ \Leftrightarrow\dfrac{x+1}{2023}+1+\dfrac{x+2}{2022}+1=\dfrac{x+3}{2021}+1+\dfrac{x+4}{2020}+1\\ \Leftrightarrow\dfrac{x+1+2023}{2023}+\dfrac{x+2+2022}{2022}-\dfrac{x+3+2021}{2021}-\dfrac{x+4+2020}{2020}=0\\ \Leftrightarrow\left(x+2024\right)\times\left(\dfrac{1}{2023}+\dfrac{1}{2022}-\dfrac{1}{2021}-\dfrac{1}{2020}\right)=0\\ \Rightarrow x+2024=0:\left(\dfrac{1}{2023}+\dfrac{1}{2022}-\dfrac{1}{2021}-\dfrac{1}{2020}\right)\\ \Rightarrow x+2024=0\\ \Rightarrow x=-2024\)
XO
1 tháng 10 2020
Ta có :\(\frac{x+4}{2018}+\frac{x+3}{2019}=\frac{x+2}{2020}+\frac{x+1}{2021}\)
=> \(\left(\frac{x+4}{2018}+1\right)+\left(\frac{x+3}{2019}+1\right)=\left(\frac{x+2}{2020}+1\right)+\left(\frac{x+1}{2021}+1\right)\)
=> \(\frac{x+2022}{2018}+\frac{x+2022}{2019}=\frac{x+2022}{2020}+\frac{x+2022}{2021}\)
=> \(\frac{x+2022}{2018}+\frac{x+2022}{2019}-\frac{x+2022}{2020}-\frac{x+2022}{2021}=0\)
=> \(\left(x+2022\right)\left(\frac{1}{2018}+\frac{1}{2019}-\frac{1}{2020}-\frac{1}{2021}\right)=0\)
Vì \(\frac{1}{2018}+\frac{1}{2019}-\frac{1}{2020}-\frac{1}{2021}\ne0\)
=> x + 2022 = 0
=> x = -2022
Vậy x = -2022
1 tháng 10 2020
\(\frac{x+4}{2018}+\frac{x+3}{2019}=\frac{x+2}{2020}+\frac{x+1}{2021}\)
\(\frac{x+4}{2018}+1+\frac{x+3}{2019}+1=\frac{x+2}{2020}+1+\frac{x+1}{2021}+1\)
\(\frac{x+4}{2018}+\frac{2018}{2018}+\frac{x+3}{2019}+\frac{2019}{2019}=\frac{x+2}{2020}+\frac{2020}{2020}+\frac{x+1}{2021}+\frac{2021}{2021}\)
\(\frac{x+2022}{2018}+\frac{x+2022}{2019}=\frac{x+2022}{2020}+\frac{x+2022}{2021}\)
\(\frac{x+2022}{2018}+\frac{x+2022}{2019}-\frac{x+2022}{2020}-\frac{x+2022}{2021}=0\)
\(\left(x+2022\right)\left(\frac{1}{2018}+\frac{1}{2019}-\frac{1}{2020}-\frac{1}{2021}\right)=0\)
\(x+2022=0\left(\frac{1}{2018}+\frac{1}{2019}-\frac{1}{2020}-\frac{1}{2021}\ne0\right)\)
\(x=0-2022\)
\(x=-2022\)
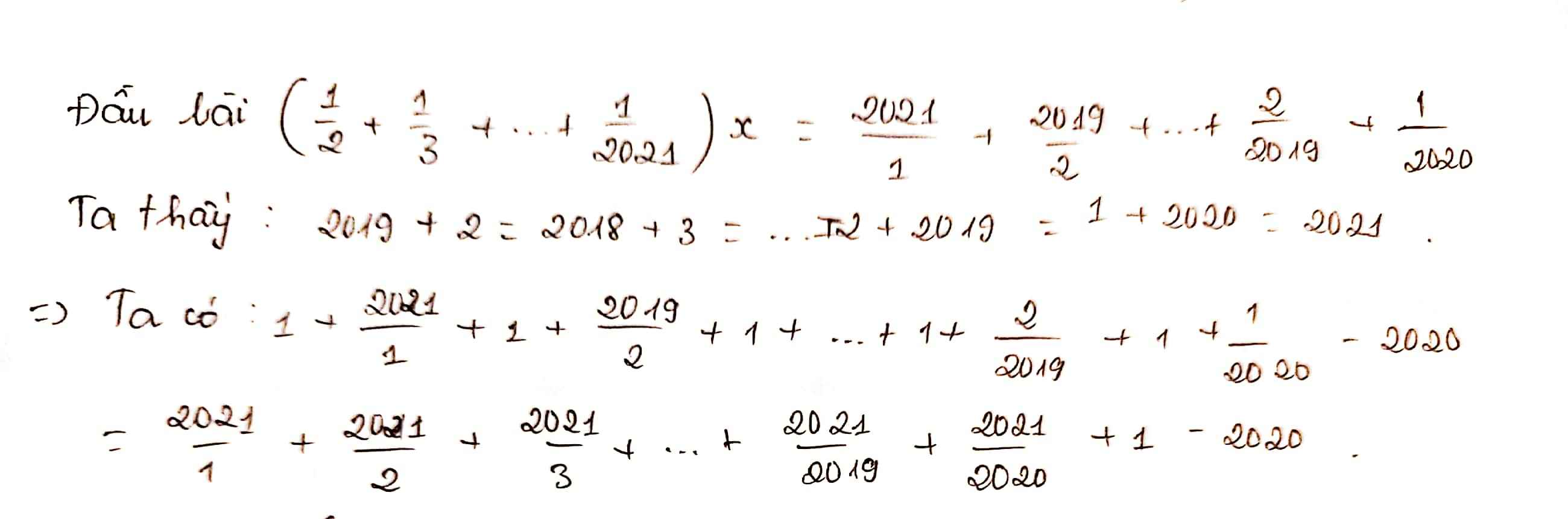
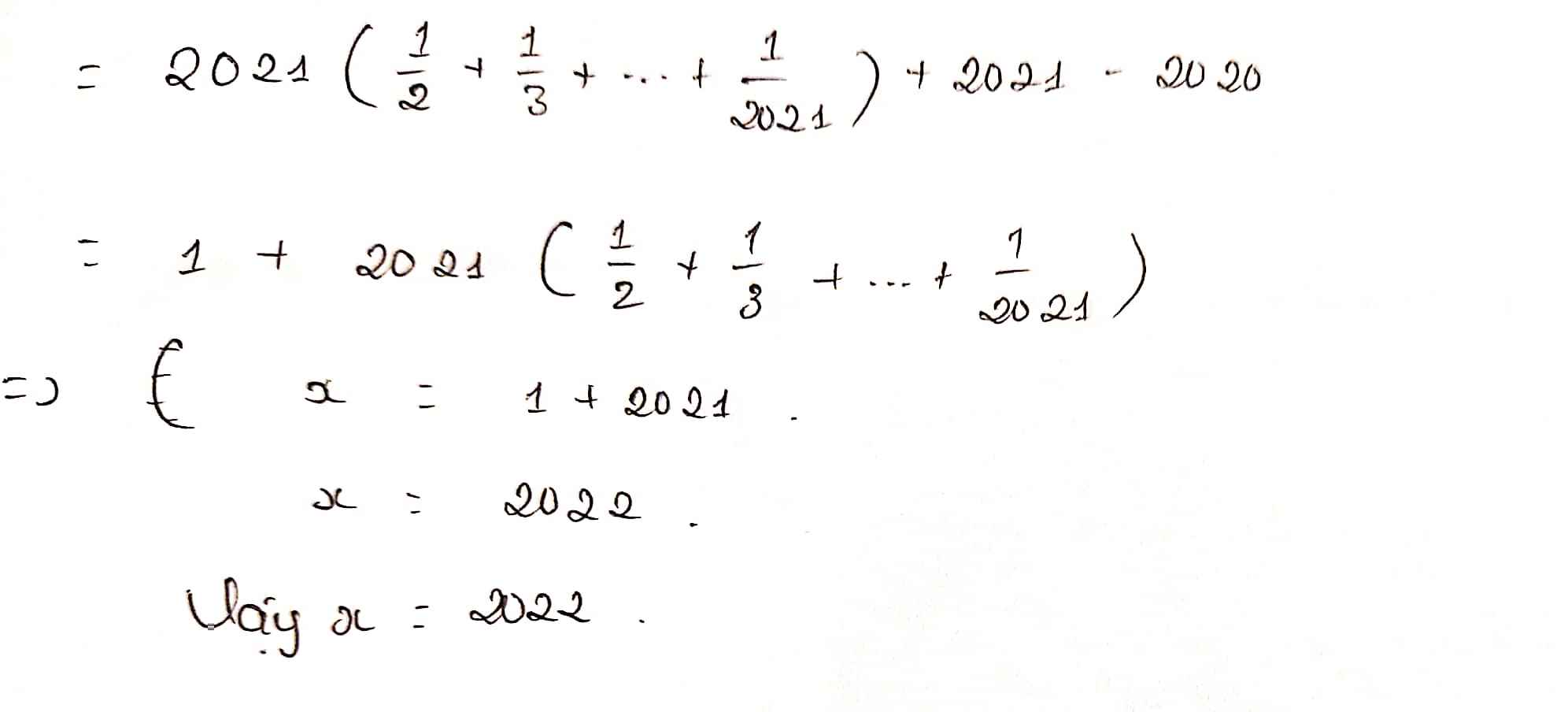
có sai đề ko bn