(8x-4)(-x2+2x-2)=0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
CM
9 tháng 9 2017
+ 2x2 + y2 – 8x + 2y – 1 = 0 không phải phương trình đường tròn vì hệ số của x2 khác hệ số của y2.
+ Phương trình x2 + y2 + 2x – 4y – 4 = 0 có :
a = –1; b = 2; c = –4 ⇒ a2 + b2 – c = 9 > 0
⇒ phương trình trên là phương trình đường tròn.
+ Phương trình x2 + y2 – 2x – 6y + 20 = 0 có :
a = 1; b = 3; c = 20 ⇒ a2 + b2 – c = –10 < 0
⇒ phương trình trên không là phương trình đường tròn.
+ Phương trình x2 + y2 + 6x + 2y + 10 = 0 có :
a = –3; b = –1; c = 10 ⇒ a2 + b2 – c = 0 = 0
⇒ phương trình trên không là phương trình đường tròn.
16 tháng 12 2021
anh ơi, vậy là sai đề hả anh, chứ đề kêu chứng minh phương trình vô nghiệm mà em thấy anh ghi x=2
CM
10 tháng 10 2019
a) Cách 1: Khai triển HĐT rút gọn được 3 x 2 + 6x + 7 = 0
Vì (3( x 2 + 2x + 1) + 4 < 0 với mọi x nên giải được x ∈ ∅
Cách 2. Chuyển vế đưa về ( x + 3 ) 3 = ( x - 1 ) 3 Û x + 3 = x - 1
Từ đó tìm được x ∈ ∅
b) Đặt x 2 = t với t ≥ 0 ta được t 2 + t - 2 = 0
Giải ra ta được t = 1 (TM) hoặc t = -2 (KTM)
Từ đó tìm được x = ± 1
c) Biến đổi được 
d) Biến đổi về dạng x(x - 2) (x - 4) = 0. Tìm được x ∈ {0; 2; 4}
NN
2
Y
11 tháng 4 2022
\(x^2-2x+1< 9\)
\(\Leftrightarrow\left(x-1\right)^2< 9\)
\(\Leftrightarrow x-1< 3\)
\(\Leftrightarrow x< 4\)
\(\left(x-1\right)\left(4-x^2\right)\ge0\)
\(\Leftrightarrow\left(x-1\right)\left(2-x\right)\left(2+x\right)\ge0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=0\\2-x=0\\2+x=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=2\\x=-2\end{matrix}\right.\)
\(\dfrac{x+2}{x-5}< 0\)
\(\Leftrightarrow x+2< 0\)
\(\Leftrightarrow x< -2\)
11 tháng 4 2022
a)\(x^2-2x+1< 9\)
\(\Leftrightarrow\left(x-1\right)^2< 9\)
\(\Leftrightarrow\left(x-1\right)^2-9< 0\)
\(\Leftrightarrow\left(x-1-3\right)\left(x-1+3\right)< 0\)
\(\Leftrightarrow\left(x-4\right)\left(x+2\right)< 0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-4< 0\\x+2>0\end{matrix}\right.hay\left[{}\begin{matrix}x-4>0\\x+2< 0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x< 4\\x>-2\end{matrix}\right.hay\left[{}\begin{matrix}x>4\\x< -2\end{matrix}\right.\)(vô lý)
-Vậy nghiệm của BĐT là \(-2< x< 4\).
b) \(\left(x-1\right)\left(4-x^2\right)\ge0\)
\(\Leftrightarrow\left(x-1\right)\left(2-x\right)\left(x+2\right)\ge0\)
\(\Leftrightarrow\left(x-1\right)\left(x-2\right)\left(x+2\right)\le0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1< 0\\x-2>0\\x+2>0\end{matrix}\right.\) hay \(\left[{}\begin{matrix}x-1>0\\x-2< 0\\x+2>0\end{matrix}\right.\) hay \(\left[{}\begin{matrix}x-1>0\\x-2 >0\\x+2< 0\end{matrix}\right.\) hay \(\left[{}\begin{matrix}x-1< 0\\x-2< 0\\x+2< 0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x< 1\\x>2\\x>-2\end{matrix}\right.\) (vô lí) hay \(\left[{}\begin{matrix}x>1\\x< 2\\x>-2\end{matrix}\right.\) (có thể xảy ra) hay
\(\left[{}\begin{matrix}x>1\\x>2\\x< -2\end{matrix}\right.\) (vô lí) hay \(\left[{}\begin{matrix}x< 1\\x< 2\\x< -2\end{matrix}\right.\) (có thể xảy ra)
-Vậy nghiệm của BĐT là \(x< -2\) hay \(1< x< 2\).
c) ĐKXĐ: \(x\ne5\)
\(\dfrac{x+2}{x-5}< 0\Leftrightarrow\left[{}\begin{matrix}x+2< 0\\x-5>0\end{matrix}\right.hay\left[{}\begin{matrix}x+2>0\\x-5< 0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x< -2\\x>5\end{matrix}\right.\)(vô lí) hay
\(\left[{}\begin{matrix}x>-2\\x< 5\end{matrix}\right.\) (có thể xảy ra)
-Vậy nghiệm của BĐT là \(-2< x< 5\)
PT
2
MN
12 tháng 2 2020
Đề bài sai ! Sửa ạ :
a) \(\left(x^2+x+1\right)\left(6-2x\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x^2+x+1=0\\6-2x=0\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}\left(x+\frac{1}{2}\right)^2+\frac{3}{4}=0\left(ktm\right)\\x=3\left(tm\right)\end{cases}}\)
Vậy tập nghiệm của phương trình là \(S=\left\{3\right\}\)
b) \(\left(8x-4\right)\left(x^2+2x+2\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}8x-4=0\\x^2+2x+2=0\end{cases}}\)
\(\Leftrightarrow\orbr{\begin{cases}x=\frac{1}{2}\left(tm\right)\\\left(x+1\right)^2+1=0\left(ktm\right)\end{cases}}\)
Vậy tập nghiệm của phương trình là \(S=\left\{\frac{1}{2}\right\}\)
13 tháng 2 2020
\(\left(x^2+x+1\right)\left(6-2x\right)=0\)
Mà \(x^2+x+1=\left(x+\frac{1}{2}\right)^2+\frac{1}{4}>0\)
\(\Rightarrow6-2x=0\Rightarrow x=3\)
NT
0
CM
9 tháng 3 2019
Đáp án: D
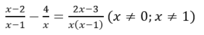
có hai nghiệm là 
. Ta thấy 
không thỏa mãn điều kiện của phương trình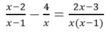 
nên 
không là nghiệm của phương trình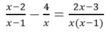 
hay 
là nghiệm ngoại lai.
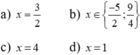
Ta nhận thấy
\(-x^2+2x-2=-\left[\left(x^2-2x+1\right)+1\right]\)
Ta có
\(x^2-2x+1\ge0\Rightarrow\left(x^2-2x+1\right)+1\ge1\)
\(\Rightarrow-\left[\left(x^2-2x+1\right)+1\right]\le-1\)
\(\Rightarrow PT\Leftrightarrow8x-4=0\Leftrightarrow x=\dfrac{1}{2}\)
\(\left(8x-4\right)\left(-x^2+2x-2\right)=0\Leftrightarrow\left(8x-4\right)\left(x^2-2x+2\right)=0\Leftrightarrow\left[{}\begin{matrix}8x-4=0\\x^2-2x+2=0\left(loai\right)\end{matrix}\right.\Leftrightarrow x=\dfrac{1}{4}\)
do \(x^2-2x+2=x^2-2x+1+1=\left(x-1\right)^2+1>0\)