(3x+2)(2x+3)(12x+7)^2=3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
SK
0
CM
30 tháng 8 2018
Điều kiện xác định: x ≠ -7; x ≠ 3/2.
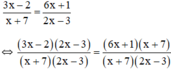
Suy ra: (3x – 2)(2x – 3) = (6x + 1)(x + 7)
⇔ 6x2 – 9x – 4x + 6 = 6x2 + 42x + x + 7
⇔ - 4x - 9x - 42x - x = 7 - 6
⇔ - 56x = 1
⇔ x = -1/56 (thỏa mãn đkxđ)
Vậy phương trình có tập nghiệm S = {-1/56}.
MP
1
NV
Nguyễn Việt Lâm
Giáo viên
8 tháng 1 2021
ĐKXĐ: ...
\(VT\le\sqrt{2\left(2x-3+5-2x\right)}=2\)
\(VP=3\left(x-2\right)^2+2\ge2\)
Dấu "=" xảy ra khi và chỉ khi:
\(\left\{{}\begin{matrix}2x-3=5-2x\\x-2=0\end{matrix}\right.\) \(\Leftrightarrow x=2\)
NT
0
LP
2
5 tháng 3 2022
1.\(\left(x+2\right)\left(2x-3\right)=x^2-4\)
\(\Leftrightarrow\left(x+2\right)\left(2x-3\right)-\left(x+2\right)\left(x-2\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(2x-3-x+2\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-2\\x=1\end{matrix}\right.\)
2.\(x^2+3x+2=0\)
\(\Leftrightarrow x^2+x+2x+2=0\)
\(\Leftrightarrow x\left(x+1\right)+2\left(x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=-2\end{matrix}\right.\)
3.\(2x^2+5x+3=0\)
\(\Leftrightarrow2x^2+2x+3x+3=0\)
\(\Leftrightarrow2x\left(x+1\right)+3\left(x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(2x+3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=-\dfrac{3}{2}\end{matrix}\right.\)
4.\(x^3+x^2-12x=0\)
\(\Leftrightarrow x\left(x^2+x-12\right)=0\)
\(\Leftrightarrow x\left(x+4\right)\left(x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-4\\x=3\end{matrix}\right.\)
5 tháng 3 2022
a: \(\Leftrightarrow\left(x+2\right)\left(2x-3\right)-\left(x+2\right)\left(x-2\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(2x-3-x+2\right)=0\)
=>(x+2)(x-1)=0
=>x=-2 hoặc x=1
b: =>(x+1)(x+2)=0
=>x=-1 hoặc x=-2
c: =>(2x+3)(x+1)=0
=>x=-1 hoặc x=-3/2
d: =>x(x+4)(x-3)=0
hay \(x\in\left\{0;-4;3\right\}\)
HP
23 tháng 1 2017
a,2x(8x-1)2(4x-1)=9(1)
<=>(8x-2)(8x-1)2.x=9
<=>8x(8x-1)2(8x-2)=8.9=72(2)
Đặt 8x-1=y ,pt (2) trở thành (y+1)y2(y-1)=72 ....... tới đây tự giải
b, tương tự ý a ,nhan 4 vào (3x+2) ,nhân 6 vào (2x+3)
c, nhân 2 vào (x+1)
12 tháng 2 2016
b/ (12x + 7)2(3x + 2)(2x + 1) = 3
=> (144x2 + 168x + 49) (6x2 + 7x + 2) = 3
- Nhân 2 vế cho 24 ta đc:
(144x2 + 168x + 49) (144x2 + 168x + 48) = 72
- Đặt a = 144x2 + 168x + 48 , ta đc phương trình:
(a + 1).a = 72
=> a2 + a - 72 = 0
=> (a + 9)(a - 8) = 0
=> a = -9 hoặc a = 8
- Với a = -9 <=> 144x2 + 168x + 48 = -9 => 144x2 + 168x + 57 = 0 , mà 144x2 + 168x + 57 > 0 => pt vô nghiệm
- Với a = 8 <=> 144x2 + 168x + 48 = 8 => 144x2 + 168x + 40 = 0 => (3x + 1)(6x + 5) = 0 => x = -1/3 hoặc x = -5/6
Vậy x = -1/3 , x = -5/6
20 tháng 1
a: \(2^{x^2-2x+1}=1\)
=>\(2^{\left(x-1\right)^2}=2^0\)
=>\(\left(x-1\right)^2=0\)
=>x-1=0
=>x=1
b: \(7^{x^2+7x}=5764801\)
=>\(7^{x^2+7x}=7^8\)
=>\(x^2+7x=8\)
=>\(x^2+7x-8=0\)
=>(x+8)(x-1)=0
=>\(\left[{}\begin{matrix}x+8=0\\x-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-8\\x=1\end{matrix}\right.\)
c: \(6^{x^2+12x}=6^{7x}\)
=>\(x^2+12x=7x\)
=>\(x^2+5x=0\)
=>x(x+5)=0
=>\(\left[{}\begin{matrix}x=0\\x+5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-5\end{matrix}\right.\)
d: \(\left(\dfrac{1}{3}\right)^{x-1}=3^{2x-5}\)
=>\(3^{-x+1}=3^{2x-5}\)
=>-x+1=2x-5
=>-x-2x=-5-1
=>-3x=-6
=>x=2
e: \(\left(\dfrac{1}{5}\right)^{3x+5}=5^{2x+1}\)
=>\(5^{-3x-5}=5^{2x+1}\)
=>-3x-5=2x+1
=>-5x=6
=>\(x=-\dfrac{6}{5}\)
NN
0