tìm GTNN
a) x2+5x+8
b) x(x-6)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
22 tháng 9 2021
\(A=\left|3-x\right|+8\ge8\)
\(minA=8\Leftrightarrow x=3\)
\(B=\left|x+2\right|-4\ge-4\)
\(minB=-4\Leftrightarrow x=-2\)
MM
0
20 tháng 10 2021
\(a,A=\left(x^2+5x+\dfrac{25}{4}\right)+\dfrac{7}{4}=\left(x+\dfrac{5}{2}\right)^2+\dfrac{7}{4}\ge\dfrac{7}{4}\\ A_{min}=\dfrac{7}{4}\Leftrightarrow x=-\dfrac{5}{2}\\ b,B=x^2-6x+9-9=\left(x-3\right)^2-9\ge9\\ B_{min}=-9\Leftrightarrow x=3\)
CM
3 tháng 3 2017
a) √16x = 8 (điều kiện: x ≥ 0)
⇔ 16x = 82 ⇔ 16x = 64 ⇔ x = 4
(Hoặc: √16x = 8 ⇔ √16.√x = 8
⇔ 4√x = 8 ⇔ √x = 2 ⇔ x = 4)
b) điều kiện: x ≥ 0
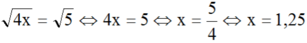
c) điều kiện: x - 1 ≥ 0 ⇔ x ≥ 1 (*)
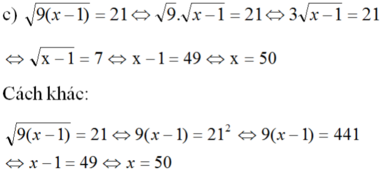
x = 50 thỏa mãn điều kiện (*) nên x = 50 là nghiệm của phương trình.
d) Vì (1 - x)2 ≥ 0 ∀x nên phương trình xác định với mọi giá trị của x.
- Khi 1 – x ≥ 0 ⇔ x ≤ 1
Ta có: 2|1 – x| = 6 ⇔ 2(1 – x) = 6 ⇔ 2(1 – x) = 6
⇔ –2x = 4 ⇔ x = –2 (nhận)
- Khi 1 – x < 0 ⇔ x > 1
Ta có: 2|1 – x| = 6 ⇔ 2[– (1 – x)] = 6
⇔ x – 1 = 3 ⇔ x = 4 (nhận)
Vậy phương trình có hai nghiệm: x = - 2; x = 4
8 tháng 11 2021
\(A=\left|x-201\right|+\left|x-204\right|=\left|x-201\right|+\left|204-x\right|\ge\left|x-201+204-x\right|=\left|3\right|=3\)
\(minA=3\Leftrightarrow\left(x-201\right)\left(204-x\right)\ge0\Leftrightarrow204\ge x\ge201\)
19 tháng 7 2021
a) Ta có: \(x^2-2x+1=25\)
\(\Leftrightarrow\left(x-1\right)^2=25\)
\(\Leftrightarrow\left[{}\begin{matrix}x-1=5\\x-1=-5\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=6\\x=-4\end{matrix}\right.\)
b) Ta có: \(\left(5x+1\right)^2-\left(5x-3\right)\left(5x+3\right)=30\)
\(\Leftrightarrow25x^2+10x+1-25x^2+9=30\)
\(\Leftrightarrow10x=20\)
hay x=2
c) Ta có: \(\left(x-1\right)\left(x^2+x+1\right)-x\left(x+2\right)\left(x-2\right)=5\)
\(\Leftrightarrow x^3-1-x\left(x^2-4\right)=5\)
\(\Leftrightarrow x^3-1-x^3+4x=5\)
\(\Leftrightarrow4x=6\)
hay \(x=\dfrac{3}{2}\)
d) Ta có: \(\left(x-2\right)^3-\left(x-3\right)\left(x^2+3x+9\right)+6\left(x+1\right)^2=15\)
\(\Leftrightarrow x^3-6x^2+12x-8-x^3+27+6\left(x^2+2x+1\right)=15\)
\(\Leftrightarrow-6x^2+12x+19+6x^2+12x+6=15\)
\(\Leftrightarrow24x=-10\)
hay \(x=-\dfrac{5}{12}\)
12 tháng 8 2021
a,\(< =>\left(x-1\right)^2-5^2=0< =>\left(x-1-5\right)\left(x-1+5\right)=0\)
\(< =>\left(x-6\right)\left(x+4\right)=0=>\left[{}\begin{matrix}x=6\\x=-4\end{matrix}\right.\)
b,\(< =>25x^2+10x+1-25x^2+9-30=0\)
\(< =>10x-20=0< =>10\left(x-2\right)=0< =>x=2\)
c,\(< =>x^3-1-x\left(x^2-4\right)-5=0\)
\(< =>x^3-1-x^2+4x-5=0< =>4x-6=0< =>x=\dfrac{6}{4}\)\(d,< =>\left(x-2\right)^3-x^3+3^3+6x^2+12x+6-15=0\)
\(< =>x^3-6x^2+12x-x^3+6x^2+12x+10=0\)
\(< =>24x+10=0< =>x=-\dfrac{5}{12}\)
12 tháng 8 2021
a: Ta có: \(x^2-2x+1=25\)
\(\Leftrightarrow\left(x-4\right)\left(x-6\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=4\\x=6\end{matrix}\right.\)
b: Ta có: \(\left(5x+1\right)^2-\left(5x-3\right)\left(5x+3\right)=30\)
\(\Leftrightarrow25x^2+10x+1-25x^2+9=30\)
\(\Leftrightarrow10x=20\)
hay x=2
c: Ta có: \(\left(x-1\right)\left(x^2+x+1\right)-x\left(x+2\right)\left(x-2\right)=5\)
\(\Leftrightarrow x^3-1-x\left(x^2-4\right)=5\)
\(\Leftrightarrow x^3-1-x^3+4x=5\)
\(\Leftrightarrow4x=6\)
hay \(x=\dfrac{3}{2}\)
NV
Nguyễn Việt Lâm
Giáo viên
27 tháng 7 2021
\(A=\dfrac{x^2-4x+1}{x^2}=\dfrac{1}{x^2}-\dfrac{4}{x}+1=\left(\dfrac{1}{x^2}-\dfrac{4}{x}+4\right)-3=\left(\dfrac{1}{x}-2\right)^2-3\ge-3\)
\(A_{min}=-3\) khi \(x=\dfrac{1}{2}\)
21 tháng 9 2021
2) \(A=-x^2-y^2+2x-6y+9=-\left(x^2-2x+1\right)-\left(y^2+6y+9\right)+19=-\left(x-1\right)^2-\left(y+3\right)^2+19\)
\(maxA=19\Leftrightarrow\)\(\left\{{}\begin{matrix}x=1\\y=-3\end{matrix}\right.\)
MM
0
x2+5x+8
=x2+2.x.5/2+25/4+7/4
=(x+5/2)2+7/4 \(\ge\)7/4 ( vì (x+5/2)2\(\ge\)0 )
Dấu "=" xảy ra khi:
x+5/2=0
<=>x=-5/2
Vậy GTNN của x2+5x+8 là 7/4 tại x=-5/2
x(x-6)=x2-6x
=x2-6x+9-9
=(x-3)2-9\(\ge\)-9( vì (x-3)2\(\ge\)0 )
Dấu "=" xảy ra khi:
x-3=0
<=>x=3
Vậy GTNN của x(x-6) là -9 tại x=3