x2 = 16
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a) \(=\left(x-2\right)^2\)
b) \(=\left(3x-2\right)^2\)
c) \(=\left(x-3y\right)^2\)
d) \(=\left(\dfrac{x}{2}+1\right)^2\)
e) \(=\left(x-4\right)^2\)
f) \(=\left(\dfrac{1}{2}xy^2+1\right)^2\)
g) \(=\left(x-1\right)\left(x+1\right)\)
h) \(=\left(5x-4\right)\left(5x+4\right)\)

Để olm giúp em em nhé!
a, \(\dfrac{x+2}{7x+42}\) = \(\dfrac{x+2}{7.\left(x+6\right)}\) = \(\dfrac{\left(x+2\right)\left(x-6\right)}{7\left(x-6\right)\left(x+6\right)}\) (đk \(x\ne\) \(\mp\) 6)
\(\dfrac{-13x}{x^2-36}\) = \(\dfrac{-13x}{\left(x-6\right)\left(x+6\right)}\) = \(\dfrac{-7.13.x}{7.\left(x-6\right).\left(x+6\right)}\) = \(\dfrac{-91x}{7.\left(x-6\right)\left(x+6\right)}\)
b, \(\dfrac{7}{4x+16}\) = \(\dfrac{7\left(x-4\right)}{4.\left(x+4\right).\left(x-4\right)}\) (đk \(x\ne\) \(\pm\) 4)
\(\dfrac{15}{x^2-16}\) = \(\dfrac{15.4}{\left(x-4\right)\left(x+4\right).4}\) = \(\dfrac{60}{4.\left(x-4\right).\left(x+4\right)}\)

a: \(16x^5-25x^3\)
\(=x^3\left(16x^2-25\right)\)
\(=x^3\left(4x-5\right)\left(4x+5\right)\)
b: \(x^2-2xy+y^2-16\)
\(=\left(x-y\right)^2-16\)
\(=\left(x-y-4\right)\left(x-y+4\right)\)
c: \(x^2-5y-xy+5x\)
\(=x\left(x-y\right)+5\left(x-y\right)\)
\(=\left(x-y\right)\left(x+5\right)\)
d: \(x^2\left(x^2+4\right)-x^2+4\)
\(=\left(x^2+4\right)\left(x^2-1\right)\)
\(=\left(x^2+4\right)\left(x-1\right)\left(x+1\right)\)

4:
\(P=\left(x+4\right)\left(x^2-4x+16\right)-\left(64-x^3\right)\)
\(=x^3+64-64+x^3=2x^3\)
Khi x=-20 thì \(P=2\cdot\left(-20\right)^3=-16000\)
=>Chọn C
2: Đề khó hiểu quá bạn ơi

x 2 − 9 − x 2 − 16 = 1 ( 1 )
ĐK: x2 ≥ 16 ⇔ x ≥ 4 hoặc x ≤ –4.
( I ) < = > x 2 − 9 = x 2 − 16 + 1 < = > x 2 − 9 = x 2 − 16 + 2 x 2 − 16 + 1 < = > 6 = 2 x 2 − 16 < = > 3 = x 2 − 16 < = > x 2 − 25 = 0 < = > x = ± 5
(thỏa mãn điều kiện)
Vậy tập nghiệm của phương trình (1) là S={–5;5}.

a: ĐKXĐ của A là x<>1; x<>-3
ĐKXĐ của B là x<>4
ĐKXĐ của C là x<>0; x<>2
ĐKXĐ của D là x<>3
ĐKXĐ của E là x<>0; x<>2
b: \(A=\dfrac{2x\left(x+3\right)}{\left(x-1\right)\left(x+3\right)}=\dfrac{2x}{x-1}\)
Để A=0 thì 2x=0
=>x=0
\(B=\dfrac{\left(x-4\right)\left(x+4\right)}{\left(x-4\right)^2}=\dfrac{x+4}{x-4}\)
Để B=0 thì x+4=0
=>x=-4
\(C=\dfrac{x\left(x+2\right)}{x\left(x-2\right)}=\dfrac{x+2}{x-2}\)
Để C=0 thì x+2=0
=>x=-2
\(D=\dfrac{\left(x+4\right)\left(x-3\right)}{\left(x-3\right)\left(x^2+3x+9\right)}=\dfrac{x+4}{x^2+3x+9}\)
Để D=0 thi x+4=0
=>x=-4
\(E=\dfrac{2x\left(x^2+2x+1\right)}{2x\left(x-2\right)}=\dfrac{\left(x+1\right)^2}{x-2}\)
Để E=0 thì (x+1)^2=0
=>x=-1

nhầm ý b, \(...+18x=\left(x+9\right)^2\)
nhìm nhầm dấu ý a + nhé bạn
d, \(..+\dfrac{1}{16}x^2\)

\(\left(x+4\right)\left(x^2-4x+16\right)=x^3+64\)
\(\left(x-3y\right)\left(x^2+3xy+9y^2\right)=x^3-27y^3\)
\(\left(x^2-\dfrac{1}{3}\right)\left(x^4+\dfrac{1}{3}x^2+\dfrac{1}{9}\right)=x^6-\dfrac{1}{27}\)
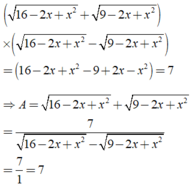
tìm x
\(x^2=16\)
\(x^2=4^2\)
\(x=\pm4\)
Vậy: ...