Tìm các giá trị n để 2n2-n+2 chia hết cho 2n+1
Giúp mình với ạ
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bài 1:
\(=-5^{22}+222+[-122-(100-5^{22})+2022]\)
\(=-5^{22}+222-122-100+5^{22}+2022\\ =(-5^{22}+5^{22})+(222-122-100)+2022\\ =0+0+2022=2022\)
Bài 2:
$2n^2+n-6\vdots 2n+1$
$\Rightarrow n(2n+1)-6\vdots 2n+1$
$\Rightarrow 6\vdots 2n+1$
$\Rightarrow 2n+1\in Ư(6)$
Mà $2n+1$ lẻ nên $2n+1\in \left\{\pm 1; \pm 3\right\}$
$\Rightarrow n\in \left\{0; -1; 1; -2\right\}$

\(A=2n^2\left(2n-1\right)-3\left(2n-1\right)+2=\left(2n^2-3\right)\left(2n-1\right)+2\)
Do \(\left(2n^2-3\right)\left(2n-1\right)⋮2n-1\)
\(\Rightarrow2⋮2n-1\)
\(\Rightarrow2n-1=Ư\left(2\right)\)
Mà 2n-1 luôn lẻ \(\Rightarrow2n-1=\left\{-1;1\right\}\)
\(\Rightarrow n=\left\{0;1\right\}\)
2.
\(Q=-\left(x^2+4x+4\right)-\left(y^2-2y+1\right)+7\)
\(Q=-\left(x+2\right)^2-\left(y-1\right)^2+7\le7\)
\(Q_{max}=7\) khi \(\left(x;y\right)=\left(-2;1\right)\)

Cách 1: Thực hiện phép chia 2n2 – n + 2 cho 2n + 1 ta có:
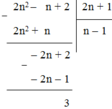
2n2 – n + 2 chia hết cho 2n + 1
⇔ 3 ⋮ (2n + 1) hay (2n + 1) ∈ Ư(3)
⇔ 2n + 1 ∈ {±1; ±3}
+ 2n + 1 = 1 ⇔ 2n = 0 ⇔ n = 0
+ 2n + 1 = -1 ⇔ 2n = -2 ⇔ n = -1
+ 2n + 1 = 3 ⇔ 2n = 2 ⇔ n = 1
+ 2n + 1 = -3 ⇔ 2n = -4 ⇔ n = -2.
Vậy n ∈ {-2; -1; 0; 1.}
Cách 2:
Ta có:
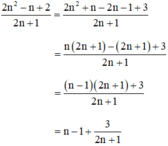
2n2 – n + 2 chia hết cho 2n + 1
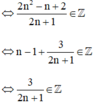
⇔ 2n + 1 ∈ Ư(3) = {±1; ± 3}.
+ 2n + 1 = 1 ⇔ 2n = 0 ⇔ n = 0
+ 2n + 1 = -1 ⇔ 2n = -2 ⇔ n = -1
+ 2n + 1 = 3 ⇔ 2n = 2 ⇔ n = 1
+ 2n + 1 = -3 ⇔ 2n = -4 ⇔ n = -2.
Vậy n ∈ {-2; -1; 0; 1.}
Chú ý: Đa thức A chia hết cho đa thức B khi phần dư của phép chia bằng 0.

Thực hiện phép chia 2n2 – n + 2 cho 2n + 1 ta có:
2n2 – n + 2 chia hết cho 2n + 1
<=> 3 \(⋮\)( 2n + 1 ) hay ( 2n + 1 ) \(\in\) Ư(3)
<=> 2n + 1 \(\in\) {\(\pm\)1; \(\pm\)3 }
+ 2n + 1 = 1 <=> 2n = 0 <=> n = 0
+ 2n + 1 = -1 <=> 2n = -2 <=> n = -1
+ 2n + 1 = 3 <=> 2n = 2 <=> n = 1
+ 2n + 1 = -3 <=> 2n = -4 <=> n = -2.
Vậy n \(\in\) { -2 ; -1 ; 0 ; 1 }

Bài 3:
Ta có: \(2n^2+n-7⋮n-2\)
\(\Leftrightarrow2n^2-4n+5n-10+3⋮n-2\)
\(\Leftrightarrow n-2\in\left\{1;-1;3;-3\right\}\)
hay \(n\in\left\{3;1;5;-1\right\}\)

\(a,A=\left(x^2-4xy+4y^2\right)+10\left(x-2y\right)+25+\left(y^2-2y+1\right)+2\\ A=\left(x-2y\right)^2+10\left(x-2y\right)+5+\left(y-1\right)^2+2\\ A=\left(x-2y+5\right)^2+\left(y-1\right)^2+2\ge2\)
Dấu \("="\Leftrightarrow\left\{{}\begin{matrix}x=2y-5\\y=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-3\\y=1\end{matrix}\right.\)
\(b,\Leftrightarrow3x^3+10x^2-5+n=\left(3x+1\right)\cdot a\left(x\right)\)
Thay \(x=-\dfrac{1}{3}\Leftrightarrow3\left(-\dfrac{1}{27}\right)+10\cdot\dfrac{1}{9}-5+n=0\)
\(\Leftrightarrow-\dfrac{1}{9}+\dfrac{10}{9}-5+n=0\\ \Leftrightarrow-4+n=0\Leftrightarrow n=4\)
\(c,\Leftrightarrow2n^2-4n+5n-10+3⋮n-2\\ \Leftrightarrow2n\left(n-2\right)+5\left(n-2\right)+3⋮n-2\\ \Leftrightarrow n-2\inƯ\left(3\right)=\left\{-3;-1;1;3\right\}\\ \Leftrightarrow n\in\left\{-1;1;3;5\right\}\)
\(2n^2-n+2⋮2n+1\)
=>\(2n^2+n-2n-1+3⋮2n+1\)
=>\(3⋮2n+1\)
=>\(2n+1\in\left\{1;-1;3;-3\right\}\)
=>\(n\in\left\{0;-1;1;-2\right\}\)