Giải giúp em phương trình này với ạ
X+1/2023 + x+3/2021= x+5/2019 + x+7/2017
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


gọi 2021-x = a
2023-x=b
2x-4044=c
ta có a + b + c=2021-x+2023-x+2x-4044=0
suy ra a + b = -c
suy ra (a+b)^3 =-c^3
ta có a^3 + b^3 + c^3=(a+b)^3 -3ab(a+b) + c^3 = -c^3 +3abc +c^3 = 3abc
ta có (2021-x)^3 + (2023-x)^3 + (2x-4044)^3 = 0
=> 3(2021-x)(2023-x)(2x-4044)=0
=> th 1 x = 2021, th 2 x = 2023; th3 x = 2022

\(\frac{x+1}{2019}+\frac{x+2}{2018}+\frac{x+3}{2017}=\frac{x-1}{2021}+\frac{x-2}{2022}+\frac{x-3}{2023}\)
\(\Leftrightarrow\left(\frac{x+1}{2019}+1\right)+\left(\frac{x+2}{2018}+1\right)+\left(\frac{x+3}{2017}+1\right)=\left(\frac{x-1}{2021}+1\right)+\left(\frac{x-2}{2022}+1\right)+\left(\frac{x-3}{2023}+1\right)\)
\(\Leftrightarrow\left(\frac{x+1+2019}{2019}\right)+\left(\frac{x+2+2018}{2018}\right)+\left(\frac{x+3+2017}{2017}\right)=\left(\frac{x-1+2021}{2021}\right)+\left(\frac{x-2+2022}{2022}\right)+\left(\frac{x-3+2023}{2023}\right)\)
\(\Leftrightarrow\frac{x+2020}{2019}+\frac{x+2020}{2018}+\frac{x+2020}{2017}=\frac{x+2020}{2021}+\frac{x+2020}{2022}+\frac{x+2020}{2023}\)
\(\Leftrightarrow\frac{x+2020}{2019}+\frac{x+2020}{2018}+\frac{x+2020}{2017}-\frac{x+2020}{2021}-\frac{x+2020}{2022}-\frac{x+2020}{2023}=0\)
\(\Leftrightarrow\left(x+2020\right)\left(\frac{1}{2019}+\frac{1}{2018}+\frac{1}{2017}-\frac{1}{2021}-\frac{1}{2022}-\frac{1}{2023}\right)=0\)
Vì \(\frac{1}{2019}+\frac{1}{2018}+\frac{1}{2017}-\frac{1}{2021}-\frac{1}{2022}-\frac{1}{2023}\ne0\)
=> x + 2020 = 0
=> x = -2020
Bài làm :
Ta có :
\(\frac{x+1}{2019}+\frac{x+2}{2018}+\frac{x+3}{2017}=\frac{x-1}{2021}+\frac{x-2}{2022}+\frac{x-3}{2023}\)
\(\Leftrightarrow\left(\frac{x+1}{2019}+1\right)+\left(\frac{x+2}{2018}+1\right)+\left(\frac{x+3}{2017}+1\right)=\left(\frac{x-1}{2021}+1\right)+\left(\frac{x-2}{2022}+1\right)+\left(\frac{x-3}{2023}+1\right)\)
\(\Leftrightarrow\left(\frac{x+1+2019}{2019}\right)+\left(\frac{x+2+2018}{2018}\right)+\left(\frac{x+3+2017}{2017}\right)=\left(\frac{x-1+2021}{2021}\right)+\left(\frac{x-2+2022}{2022}\right)+\left(\frac{x-3+2023}{2023}\right)\)
\(\Leftrightarrow\frac{x+2020}{2019}+\frac{x+2020}{2018}+\frac{x+2020}{2017}=\frac{x+2020}{2021}+\frac{x+2020}{2022}+\frac{x+2020}{2023}\)
\(\Leftrightarrow\frac{x+2020}{2019}+\frac{x+2020}{2018}+\frac{x+2020}{2017}-\frac{x+2020}{2021}-\frac{x+2020}{2022}-\frac{x+2020}{2023}=0\)
\(\Leftrightarrow\left(x+2020\right)\left(\frac{1}{2019}+\frac{1}{2018}+\frac{1}{2017}-\frac{1}{2021}-\frac{1}{2022}-\frac{1}{2023}\right)=0\)
\(\text{Vì : }\frac{1}{2019}+\frac{1}{2018}+\frac{1}{2017}-\frac{1}{2021}-\frac{1}{2022}-\frac{1}{2023}\ne0\)
\(\Rightarrow x+2020=0\Leftrightarrow x=-2020\)
Vậy x=-2020

Hướng làm:
Thấy cả tử mẫu cộng lại đều bằng 2021 → Cộng thêm 1 rồi quy đồng với mỗi phân thức
\(\dfrac{x+2}{2019}+1+\dfrac{x+3}{2018}+1=\dfrac{x+4}{2017}+1+\dfrac{x}{2021}+1\\ \Leftrightarrow\dfrac{x+2021}{2019}+\dfrac{x+2021}{2018}-\dfrac{x+2021}{2017}-\dfrac{x+2021}{2021}=0\\ \Leftrightarrow\left(x+2021\right)\left(\dfrac{1}{2019}+\dfrac{1}{2018}-\dfrac{1}{2017}-\dfrac{1}{2021}\right)=0\\ \Leftrightarrow x+2021=0\Leftrightarrow x=-2021\)
\(< =>\dfrac{x+2}{2019}+1+\dfrac{x+3}{2018}+1=\dfrac{x+4}{2017}+1+\dfrac{x}{2021}+1\)
\(< =>\dfrac{x+2+2019}{2019}+\dfrac{x+3+2018}{2018}=\dfrac{x+4+2017}{2017}+\dfrac{x+2021}{2021}\)
\(< =>\dfrac{x+2021}{2019}+\dfrac{x+2021}{2018}-\dfrac{x+2021}{2017}-\dfrac{x+2021}{2021}=0\)
\(< =>\left(x+2021\right)\left(\dfrac{1}{2019}+\dfrac{1}{2018}-\dfrac{1}{2017}-\dfrac{1}{2021}=\right)=0\)
\(< =>x+2021=0< =>x=-2021\)
Vậy....

a, TK: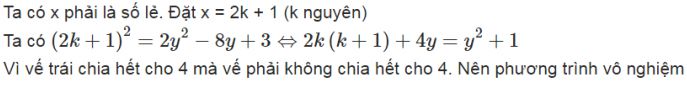
(x lẻ do \(2y^2-8y+3=2\left(y^2-4y\right)+3=x^2\) lẻ)
\(b,\Leftrightarrow\left(x^2-4x+4\right)+\left(y^2+4y+4\right)=9\\ \Leftrightarrow\left(x-2\right)^2+\left(y+2\right)^2=9\)
Vậy pt vô nghiệm do 9 ko phải tổng 2 số chính phương

\(\frac{x+2}{2019}+\frac{x+3}{2018}=\frac{x+4}{2017}+\frac{x}{2021}\)
\(\Leftrightarrow\frac{x+2}{2019}+1+\frac{x+3}{2018}+1=\frac{x+4}{2017}+1+\frac{x}{2021}+1\)
\(\Leftrightarrow\frac{x+2021}{2019}+\frac{x+2021}{2018}=\frac{x+2021}{2017}+\frac{x+2021}{2021}\)
\(\Leftrightarrow x+2021=0\)
\(\Leftrightarrow x=-2021\)

\(\dfrac{x-1}{2023}+\dfrac{x-2}{2022}=\dfrac{x-3}{2021}+\dfrac{x-4}{2020}\)
`<=>(x-1)/2023-1+(x-2)/2022-1=(x-3)/2021-1+(x-4)/2020-1`
`<=>(x-2024)/2023+(x-2024)/2022=(x-2024)/2021+(x-2024)/2020`
`<=>(x-2024)(1/2023+1/2022-1/2021-1/2020)=0`
`<=>x-2024=0(1/2023+1/2022-1/2021-1/2020>0)`
`<=>x=2024`
=>\(\left(\dfrac{x-1}{2023}-1\right)+\left(\dfrac{x-2}{2022}-1\right)=\left(\dfrac{x-3}{2021}-1\right)+\left(\dfrac{x-4}{2020}-1\right)\)
=>x-2024=0
=>x=2024

\(\dfrac{x-1}{2019-2}+\dfrac{x-3}{2019}=\dfrac{x-5}{2021}+\dfrac{x-7}{2023}\)
\(\Leftrightarrow\dfrac{x-1}{2017}+\dfrac{x-3}{2019}=\dfrac{x-5}{2021}+\dfrac{x-7}{2023}\)
\(\Leftrightarrow\left(\dfrac{x-1}{2017}+1\right)+\left(\dfrac{x-3}{2019}+1\right)=\left(\dfrac{x-5}{2021}+1\right)+\left(\dfrac{x-7}{2023}+1\right)\)
=>x+2016=0
hay x=-2016

Sửa đề:
A = 1 - 3 - 5 + 7 + 9 - 11 - 13 + 15 + ... + (2017 - 2019 - 2021 + 2023)
Số số hạng của A:
(2023 - 1) : 2 + 1 = 1012 (số)
Do 1012 chia hết cho 4 nên ta nhóm các số hạng thành từng nhóm, mỗi nhóm có 4 số hạng như sau:
A = 1 - 3 - 5 + 7 + 9 - 11 - 13 + 15 + ... + 2017 - 2019 - 2021 + 2023
= (1 - 3 - 5 + 7) + (9 - 11 - 13 + 15) + ... + (2017 - 2018 - 2021 + 2023)
= 0 + 0 + ... + 0
= 0
\(\dfrac{x+1}{2023}+\dfrac{x+3}{2021}=\dfrac{x+5}{2019}+\dfrac{x+7}{2017}\)
\(\Leftrightarrow\dfrac{x+1}{2023}+1+\dfrac{x+3}{2021}+1=\dfrac{x+5}{2019}+1+\dfrac{x+7}{2019}+1\)
\(\Leftrightarrow\dfrac{x+2024}{2023}+\dfrac{x+2024}{2021}=\dfrac{x+2024}{2019}+\dfrac{x+2024}{2027}\)
\(\Leftrightarrow\left(x+2024\right)\left(\dfrac{1}{2023}+\dfrac{1}{2021}-\dfrac{1}{2019}-\dfrac{1}{2017}\right)=0\)
\(\Leftrightarrow x+2024=0\)
\(\Leftrightarrow x=-2024\)