tìm x biết
b) /2x-3/ + ( y-1)^2+ ( 27-1 )^2 \(\le\) 0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
5 tháng 8 2017
a) hình như thiếu đề nha (theo mk nghỉ thì đề như thế này) : tìm x nguyên
(nếu đề đúng là : "tìm x nguyên" thì lời giải đây nha)
bài làm
ta có \(\left(đk:x\in Z\right)\) : x nguyên \(\Rightarrow x-1\) nguyên
\(x^2-2x+3\) chia hết cho \(x-1\) \(\Leftrightarrow\dfrac{x^2-2x+3}{x-1}\) là số nguyên
\(=\dfrac{x^2-2x+1+2}{x-1}=\dfrac{\left(x-1\right)^2+2}{x-1}=x-1+\dfrac{2}{x-1}\)
\(\Rightarrow\) \(x-1\) là ước của 2 là \(\pm1;\pm2\)
ta có : * \(x-1=1\Leftrightarrow x=2\left(tmđk\right)\)
* \(x-1=-1\Leftrightarrow x=0\left(tmđk\right)\)
* \(x-1=2\Leftrightarrow x=3\left(tmđk\right)\)
* \(x-1=-2\Leftrightarrow x=-1\left(tmđk\right)\)
vậy \(x=0;x=-1;x=2;x=3\)
NK
0
LH
3
KN
30 tháng 7 2019
\(\left(y-2\right)\left(y-3\right)+\left(y-2\right)-1=0\)
\(\Leftrightarrow\left(y-2\right)\left(y-3\right)+\left(y-3\right)=0\)
\(\Leftrightarrow\left(y-3\right)^2=0\)
\(\Leftrightarrow y=3\)
\(x^3+27+\left(x+3\right)\left(x-9\right)=0\)
\(\Leftrightarrow\left(x+3\right)\left(x^2-3x+9\right)+\left(x+3\right)\left(x-9\right)=0\)
\(\Leftrightarrow\left(x+3\right)\left(x^2-3x+9+x-9\right)=0\)
\(\Leftrightarrow\left(x+3\right)\left(x^2-2x\right)=0\)
\(\Leftrightarrow\left(x+3\right)x\left(x-2\right)=0\)
\(\Leftrightarrow x\in\left\{0;-3;2\right\}\)
19 tháng 2 2021
Bài 1:tính
a)65.(-19)+19.(-35)
=65.(-19)+(-19).35
=(-19).(65+35)
=(-19).100
=-1900
b)85.(35-27)-35.(85-27)
=85.35-85.27-35.85+35.27
=(85.35-35.85)+(-85.27+35.27)
=27.(-85+35)
=27.(-50)
=1350
c)47.(45-15)-47.(45+15)
=47.[(45-15)-(45+15)]
=47.[30-60]
=47.(-30)
=-1410
Bài2: Tìm các số nguyên x biết
a)(-2).(x+6)+6.(x-10)=8
-2x-12+6x-60=8
4x-72=8
4x=72+8
4x=50
x=\(\frac{25}{2}\)
b)(-4).(2x+9)-(-8x+3)-(x+13)=0
-6x-36+8x-3-x-13=0
x-41=0
x=41
LT
19 tháng 2 2021
Bài 1: Tính
a) \(65.\left(-19\right)+19.\left(-35\right)\)
= \(-1235+-665\)
= \(-1900\)
b) \(85.\left(35-27\right)-35.\left(85-27\right)\)
= \(-1350\)
c) \(47.\left(45-15\right)-47.\left(45+15\right)\)
=\(-1410\)
Bài 2: Tìm x:
\(\left(-2\right).\left(x+6\right)+6.\left(x-10\right)=8\)
\(x=20\)
S
9 tháng 6 2017
a, Ta thấy: \(\hept{\begin{cases}\left|x+\frac{3}{4}\right|\ge0\\\left|y-\frac{2}{5}\right|\ge0\\\left|z+\frac{1}{2}\right|\ge0\end{cases}\Rightarrow\left|x+\frac{3}{4}\right|+\left|y-\frac{2}{5}\right|+\left|z+\frac{1}{2}\right|\ge0}\)
Mà đề cho là \(\left|x+\frac{3}{4}\right|+\left|y-\frac{2}{5}\right|+\left|z+\frac{1}{2}\right|\le0\)
\(\Rightarrow\hept{\begin{cases}x+\frac{3}{4}=0\\y-\frac{2}{5}=0\\z+\frac{1}{2}=0\end{cases}\Rightarrow\hept{\begin{cases}x=\frac{-3}{4}\\y=\frac{2}{5}\\z=\frac{-1}{2}\end{cases}}}\)
Vậy....
b, (x - 2)2 = 1
=> x - 2 = 1 hoặc x - 2 = -1
=> x = 3 hoặc x = 1
Vậy...
c, (2x - 1)3 = -27
=> 2x - 1 = -3
=> 2x = -2
=> x = -1
KK
25 tháng 5 2017
4) Áp dụng bất đẳng thức Bunyakovsky
\(\Rightarrow\left(x^4+yz\right)\left(1+1\right)\ge\left(x^2+\sqrt{yz}\right)^2\)
\(\Rightarrow\dfrac{x^2}{x^4+yz}\le\dfrac{2x^2}{\left(x^2+\sqrt{yz}\right)^2}\)
Tượng tự ta có \(\left\{{}\begin{matrix}\dfrac{y^2}{y^4+xz}\le\dfrac{2y^2}{\left(y^2+\sqrt{xz}\right)^2}\\\dfrac{z^2}{z^4+xy}\le\dfrac{2z^2}{\left(z^2+\sqrt{xy}\right)^2}\end{matrix}\right.\)
\(\Rightarrow VT\le2\left[\dfrac{x^2}{\left(x^2+\sqrt{yz}\right)^2}+\dfrac{y^2}{\left(y^2+\sqrt{xz}\right)^2}+\dfrac{z^2}{\left(z^2+\sqrt{xy}\right)}\right]\)
Chứng minh rằng \(2\left[\dfrac{x^2}{\left(x^2+\sqrt{yz}\right)^2}+\dfrac{y^2}{\left(y^2+\sqrt{xz}\right)^2}+\dfrac{z^2}{\left(z^2+\sqrt{xy}\right)}\right]\le\dfrac{3}{2}\)
\(\Leftrightarrow\dfrac{x^2}{\left(x^2+\sqrt{yz}\right)^2}+\dfrac{y^2}{\left(y^2+\sqrt{xz}\right)^2}+\dfrac{z^2}{\left(z^2+\sqrt{xy}\right)^2}\le\dfrac{3}{4}\)
Áp dụng bất đẳng thức Cauchy
\(\Rightarrow x^2+\sqrt{yz}\ge2\sqrt{x^2\sqrt{yz}}=2x\sqrt{\sqrt{yz}}\)
\(\Rightarrow\left(x^2+\sqrt{yz}\right)^2\ge4x^2\sqrt{yz}\)
\(\Rightarrow\dfrac{x^2}{\left(x^2+\sqrt{yz}\right)^2}\le\dfrac{x^2}{4x^2\sqrt{yz}}=\dfrac{1}{4\sqrt{yz}}\)
Tượng tự ta có \(\left\{{}\begin{matrix}\dfrac{y^2}{\left(y^2+\sqrt{xz}\right)^2}\le\dfrac{1}{4\sqrt{xz}}\\\dfrac{z^2}{\left(z^2+\sqrt{xy}\right)^2}\le\dfrac{1}{4\sqrt{xy}}\end{matrix}\right.\)
\(\Leftrightarrow\dfrac{x^2}{\left(x^2+\sqrt{yz}\right)^2}+\dfrac{y^2}{\left(y^2+\sqrt{xz}\right)^2}+\dfrac{z^2}{\left(z^2+\sqrt{xy}\right)^2}\le\dfrac{1}{4}\left(\dfrac{1}{\sqrt{xy}}+\dfrac{1}{\sqrt{yz}}+\dfrac{1}{\sqrt{xz}}\right)\)
Chứng minh rằng \(\dfrac{1}{4}\left(\dfrac{1}{\sqrt{xy}}+\dfrac{1}{\sqrt{yz}}+\dfrac{1}{\sqrt{xz}}\right)\le\dfrac{3}{4}\)
\(\Leftrightarrow\dfrac{1}{\sqrt{xy}}+\dfrac{1}{\sqrt{yz}}+\dfrac{1}{\sqrt{xz}}\le3\)
Theo đề bài ta có \(x^2+y^2+z^2=3xyz\)
\(\Rightarrow\dfrac{x}{yz}+\dfrac{y}{xz}+\dfrac{z}{xy}=3\)
\(\Rightarrow\dfrac{1}{\sqrt{xy}}+\dfrac{1}{\sqrt{yz}}+\dfrac{1}{\sqrt{xz}}\le3\)
\(\Leftrightarrow\dfrac{1}{\sqrt{xy}}+\dfrac{1}{\sqrt{yz}}+\dfrac{1}{\sqrt{xz}}\le\dfrac{x}{yz}+\dfrac{y}{xz}+\dfrac{z}{xy}\)
Áp dụng bất đẳng thức Cauchy
\(\Rightarrow\dfrac{1}{\sqrt{xy}}\le\dfrac{\dfrac{1}{x}+\dfrac{1}{y}}{2}\)
Tượng tự ta có \(\left\{{}\begin{matrix}\dfrac{1}{\sqrt{xz}}\le\dfrac{\dfrac{1}{x}+\dfrac{1}{z}}{2}\\\dfrac{1}{\sqrt{yz}}\le\dfrac{\dfrac{1}{z}+\dfrac{1}{y}}{2}\end{matrix}\right.\)
\(\Rightarrow\dfrac{1}{\sqrt{xy}}+\dfrac{1}{\sqrt{yz}}+\dfrac{1}{\sqrt{xz}}\le\dfrac{1}{x}+\dfrac{1}{y}+\dfrac{1}{z}\) (1)
Áp dụng bất đẳng thức Cauchy
\(\Rightarrow\dfrac{x}{yz}+\dfrac{y}{xz}\ge2\sqrt{\dfrac{1}{z^2}}=\dfrac{2}{z}\)
Tượng tự ta có \(\left\{{}\begin{matrix}\dfrac{y}{xz}+\dfrac{z}{xy}\ge\dfrac{2}{x}\\\dfrac{x}{zy}+\dfrac{z}{xy}\ge\dfrac{2}{y}\end{matrix}\right.\)
\(\Rightarrow2\left(\dfrac{x}{yz}+\dfrac{y}{xz}+\dfrac{z}{xy}\right)\ge2\left(\dfrac{1}{x}+\dfrac{1}{y}+\dfrac{1}{z}\right)\)
\(\Leftrightarrow\dfrac{x}{yz}+\dfrac{y}{xz}+\dfrac{z}{xy}\ge\dfrac{1}{x}+\dfrac{1}{y}+\dfrac{1}{z}\) (2)
Từ (1) và (2)
\(\Rightarrow\dfrac{1}{\sqrt{xy}}+\dfrac{1}{\sqrt{yz}}+\dfrac{1}{\sqrt{xz}}\le3\) ( đpcm )
Vậy \(\dfrac{1}{4}\left(\dfrac{1}{\sqrt{xy}}+\dfrac{1}{\sqrt{yz}}+\dfrac{1}{\sqrt{xz}}\right)\le\dfrac{3}{4}\)
\(\Rightarrow2\left[\dfrac{x^2}{\left(x^2+\sqrt{yz}\right)^2}+\dfrac{y^2}{\left(y^2+\sqrt{xz}\right)^2}+\dfrac{z^2}{\left(z^2+\sqrt{xy}\right)}\right]\le\dfrac{3}{2}\)
Mà \(VT\le2\left[\dfrac{x^2}{\left(x^2+\sqrt{yz}\right)^2}+\dfrac{y^2}{\left(y^2+\sqrt{xz}\right)^2}+\dfrac{z^2}{\left(z^2+\sqrt{xy}\right)}\right]\)
\(\Rightarrow VT\le\dfrac{3}{2}\) ( đpcm )
Dấu " = " xảy ra khi \(x=y=z=1\)
BN
25 tháng 5 2017
3. Ta có :\(x^2\left(1-2x\right)=x.x.\left(1-2x\right)\le\dfrac{\left(x+x+1-2x\right)^3}{27}=\dfrac{1}{27}\)(bđt cô si)
Dấu "=" xảy ra khi :x=1-2x\(\Leftrightarrow x=\dfrac{1}{3}\)
Vậy max của Qlaf 1/27 khi x=1/3
24 tháng 9 2023
Tham khảo:
a) Vẽ đường thẳng \(\Delta : - 2x + y - 1 = 0\) đi qua hai điểm \(A(0;1)\) và \(B\left( { - 1; - 1} \right)\)
Xét gốc tọa độ \(O(0;0).\) Ta thấy \(O \notin \Delta \) và \( - 2.0 + 0 - 1 = - 1 < 0\)
Do đó, miền nghiệm của bất phương trình là nửa mặt phẳng kể cả bờ \(\Delta \), chứa gốc tọa độ O
(miền không gạch chéo trên hình)
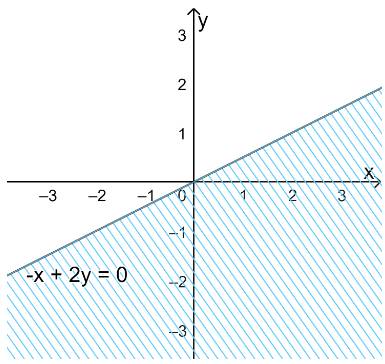
b) Vẽ đường thẳng \(\Delta : - x + 2y = 0\) đi qua hai điểm \(O(0;0)\) và \(B\left( {2;1} \right)\)
Xét điểm \(A(1;0).\) Ta thấy \(A \notin \Delta \) và \( - 1 + 2.0 = - 1 < 0\)
Do đó, miền nghiệm của bất phương trình là nửa mặt phẳng không kể bờ \(\Delta \), không chứa điểm A (1;0)
(miền không gạch chéo trên hình)
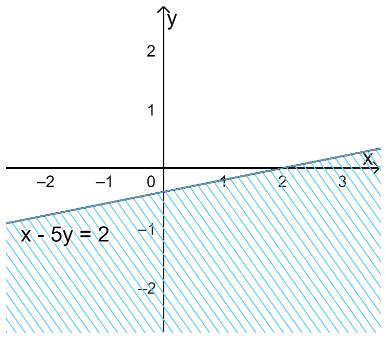
c) Vẽ đường thẳng \(\Delta :x - 5y = 2\) đi qua hai điểm \(A(2;0)\) và \(B\left( { - 3; - 1} \right)\)
Xét gốc tọa độ \(O(0;0).\) Ta thấy \(O \notin \Delta \) và \(0 - 5.0 = 0 < 2\)
Do đó, miền nghiệm của bất phương trình là nửa mặt phẳng không kể bờ \(\Delta \), chứa gốc tọa độ O
(miền không gạch chéo trên hình)
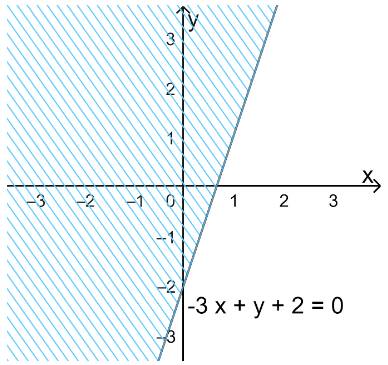
d) Vẽ đường thẳng \(\Delta : - 3x + y + 2 = 0\) đi qua hai điểm \(A(0; - 2)\) và \(B\left( {1;1} \right)\)
Xét điểm \(O(0;0).\) Ta thấy \(O \notin \Delta \) và \( - 3.0 + 0 + 2 = 2 > 0\)
Do đó, miền nghiệm của bất phương trình là nửa mặt phẳng kể cả bờ \(\Delta \), không chứa điểm O (0;0)
(miền không gạch chéo trên hình)
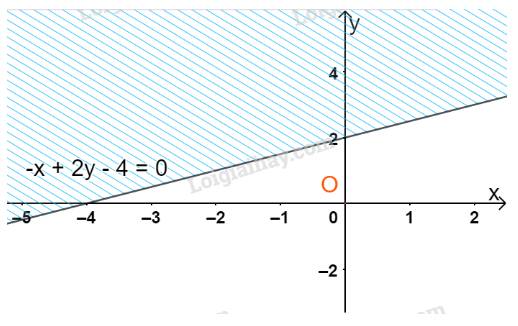
e) Ta có: \(3(x - 1) + 4(y - 2) < 5x - 3 \Leftrightarrow - 2x + 4y - 8 < 0 \Leftrightarrow - x + 2y - 4 < 0\)
Vẽ đường thẳng \(\Delta : - x + 2y -4 = 0\) đi qua hai điểm \(A(0;2)\) và \(B\left( {-4;0} \right)\)
Xét điểm \(O(0;0).\) Ta thấy \(O \notin \Delta \) và \( - 0 + 2.0 -4 = -4 < 0\)
Do đó, miền nghiệm của bất phương trình là nửa mặt phẳng không kể bờ \(\Delta \), chứa điểm O (0;0)
(miền không gạch chéo trên hình)
12 tháng 6 2017
a/ \(x^2+y^2=x^2+y^2+2xy-2xy =\left(x+y\right)^2-2xy\)
b/ mình không chắc nữa
bài 3
a/ \(9x^2-49=0 \Leftrightarrow x^2=\frac{49}{9} \Leftrightarrow\orbr{\begin{cases}x=\frac{7}{3}\\x=-\frac{7}{3}\end{cases}}\)
b/ \(\left(x+3\right)\left(x^2-3x+9\right)-x\left(x+1\right)\left(x-1\right)-27=0 \Leftrightarrow x^3+27-x\left(x^2-1\right)-27=0\)
\(\Leftrightarrow x^3-x^3+x=0\Leftrightarrow x=0\)
c/\(\left(x-1\right)\left(x+2\right)-x-2=0 \Leftrightarrow \left(x-1\right)\left(x+2\right)-\left(x+2\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(x-1\right)^2=0\Leftrightarrow\orbr{\begin{cases}x+2=0\\x-1=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=-2\\x=1\end{cases}}}\)
d/ \(x\left(3x+2\right)+\left(x+1\right)^2-\left(2x-5\right)\left(2x+5\right)=0\)
\(\Leftrightarrow3x^2+2x+x^2+2x+1-4x^2+25=0\)
\(\Leftrightarrow4x+25=0 \Leftrightarrow x=\frac{-25}{4}\)
e/ mình lười qá ko viết đề đâu
\(\Leftrightarrow4x^2-7x-2-4x^2+4x+3=7\)
\(\Leftrightarrow-3x+1=7 \Leftrightarrow x=-2\)
có gì sai bn sửa lại nha