phân tích đa thức sau thành nhân tử:
(x2+x+1)(x2+x+2)-12
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


-Đặt \(t=\left(x^2-x+1\right)\)
\(\left(x^2-x+1\right)^2-5x\left(x^2-x+1\right)+4x^2\)
\(=t^2-5xt+4x^2\)
\(=t^2-4xt-xt+4x^2\)
\(=t\left(t-4x\right)-x\left(t-4x\right)\)
\(=\left(t-4x\right)\left(t-x\right)\)
\(=\left(x^2-x+1-4x\right)\left(x^2-x+1-x\right)\)
\(=\left(x^2-5x+1\right)\left(x^2-2x +1\right)\)
\(=\left(x^2-5x+1\right)\left(x-1\right)^2\)

a) Áp dụng HĐT 1 thu được ( 2 x + y ) 2 .
b) Áp dụng HĐT 3 với A = 2x + l; B = x - l thu được
[(2x +1) + (x -1)] [(2x +1) - (x -1)] rút gọn thành 3x(x + 2).
c) Ta có: 9 - 6x + x 2 - y 2 = ( 3 - x ) 2 - y 2 = (3 - x - y)(3 -x + y).
d) Ta có: -(x + 2) + 3( x 2 - 4) = -{x + 2) + 3(x + 2)(x - 2)
= (x + 2) [-1 + 3(x - 2)] = (x + 2)(3x - 7).

Cách 1: Tách một hạng tử thành tổng hai hạng tử để xuất hiện nhân tử chung.
a) x2 – 3x + 2
= x2 – x – 2x + 2 (Tách –3x = – x – 2x)
= (x2 – x) – (2x – 2)
= x(x – 1) – 2(x – 1) (Có x – 1 là nhân tử chung)
= (x – 1)(x – 2)
Hoặc: x2 – 3x + 2
= x2 – 3x – 4 + 6 (Tách 2 = – 4 + 6)
= x2 – 4 – 3x + 6
= (x2 – 22) – 3(x – 2)
= (x – 2)(x + 2) – 3.(x – 2) (Xuất hiện nhân tử chung x – 2)
= (x – 2)(x + 2 – 3) = (x – 2)(x – 1)
b) x2 + x – 6
= x2 + 3x – 2x – 6 (Tách x = 3x – 2x)
= x(x + 3) – 2(x + 3) (có x + 3 là nhân tử chung)
= (x + 3)(x – 2)
c) x2 + 5x + 6 (Tách 5x = 2x + 3x)
= x2 + 2x + 3x + 6
= x(x + 2) + 3(x + 2) (Có x + 2 là nhân tử chung)
= (x + 2)(x + 3)
Cách 2: Đưa về hằng đẳng thức (1) hoặc (2)
a) x2 – 3x + 2
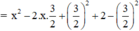
(Vì có x2 và 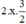 nên ta thêm bớt
nên ta thêm bớt 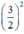 để xuất hiện HĐT)
để xuất hiện HĐT)
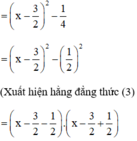
= (x – 2)(x – 1)
b) x2 + x - 6

= (x – 2)(x + 3).
c) x2 + 5x + 6

= (x + 2)(x + 3).

Cách 1: x2 – 4 + (x – 2)2
(Xuất hiện hằng đẳng thức (3))
= (x2– 22) + (x – 2)2
= (x – 2)(x + 2) + (x – 2)2
(Có nhân tử chung x – 2)
= (x – 2)[(x + 2) + (x – 2)]
= (x – 2)(x + 2 + x – 2)
= (x – 2)(2x)
= 2x(x – 2)
Cách 2: x2 – 4 + (x – 2)2
(Khai triển hằng đẳng thức (2))
= x2 – 4 + (x2 – 2.x.2 + 22)
= x2 – 4 + x2 – 4x + 4
= 2x2 – 4x
(Có nhân tử chung là 2x)
= 2x(x – 2)

\(x^2\left(x-3\right)+4\left(3-x\right)\)\(=x^2\left(x-3\right)-4\left(x-3\right)\)
\(=\left(x^2-4\right)\left(x-3\right)\)\(=\left(x-2\right)\left(x+2\right)\left(x-3\right)\)
\(x^2\left(x-3+12-4x\right)\)
\(=x^2\left(x-3\right)-4\left(x-3\right)\)
\(=\left(x-3\right)\left(x^2-4\right)\)
\(=\left(x-3\right)\left(x-2\right)\left(x+2\right)\)

a: \(x^2-6x+5=\left(x-5\right)\left(x-1\right)\)
b: \(x^2-x-12=\left(x-4\right)\left(x+3\right)\)
c: \(x^2+8x+15=\left(x+5\right)\left(x+3\right)\)
d: \(2x^2-5x-12=\left(x-4\right)\left(2x+3\right)\)
e: \(x^2-13x+36=\left(x-9\right)\left(x-4\right)\)

\(\left(x^2+x+1\right)\left(x^2+x+5\right)-21=x^4+x^3+5x^2+x^3+x^2+5x+x^2+x+5-21=x^4+2x^3+7x^2+6x-16=\left(x-1\right)\left(x+2\right)\left(x^2+x+8\right)\)
\(=\left(x^2+x+1\right)\left(x^2+x+1+4\right)-21\)
\(=\left(x^2+x+1\right)^2+4\left(x^2+x+1\right)-21\)
\(=\left(x^2+x+1\right)^2-3\left(x^2+x+1\right)+7\left(x^2+x+1\right)-21\)
\(=\left(x^2+x+1\right)\left(x^2+x-2\right)+7\left(x^2+x-2\right)\)
\(=\left(x^2+x-2\right)\left(x^2+x+8\right)\)
\(=\left(x-1\right)\left(x-2\right)\left(x^2+x+8\right)\)
(x2+x+1)(x2+x+2)-12
=\(\left(x^2+x+\frac{3}{2}-\frac{1}{2}\right)\left(x^2+x+\frac{3}{2}+\frac{1}{2}\right)-12\)
=\(\left(x^2+x+\frac{3}{2}\right)^2-\frac{1}{4}-12\)
=\(\left(x^2+x+\frac{3}{2}\right)^2-\frac{49}{4}\)
=\(\left(x^2+x+\frac{3}{2}-\frac{7}{2}\right)\left(x^2+x+\frac{3}{2}+\frac{7}{2}\right)\)
=\(\left(x^2+x-2\right)\left(x^2+x+5\right)\)
\(A=\left(x^2+x+1\right)\left(x^2+x+2\right)-12\)
Đặt: \(x^2+x+1=t\) Khi đó ta có:
\(A=t\left(t+1\right)-12\)
\(=t^2+t-12=\left(t-3\right)\left(t+4\right)\)
Thay trở lại đc:
\(A=\left(x^2+x-2\right)\left(x^2+x+5\right)\)
\(=\left(x-1\right)\left(x+2\right)\left(x^2+x+5\right)\)