Question

[Quoc Tran Anh Le]

a) Trong hình 4.14a, hãy chỉ ra vectơ \(\overrightarrow a  + \overrightarrow b \)và vectơ \(\overrightarrow b  + \overrightarrow a \).

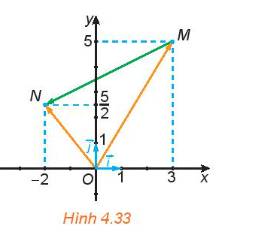
b) Trong hình 4.14b, hãy chỉ ra vectơ \(\left( {\overrightarrow a  + \overrightarrow b } \right) + \overrightarrow c \)và vectơ \(\overrightarrow a  + \left( {\overrightarrow b  + \overrightarrow c } \right)\).

Answer 1

[Hà Quang Minh]

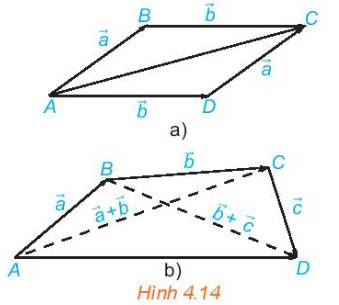
a) Ta có: \(\overrightarrow {AB}  = \overrightarrow a ,\;\overrightarrow {BC}  = \overrightarrow b \) nên \(\overrightarrow a  + \overrightarrow b  = \overrightarrow {AB}  + \overrightarrow {BC}  = \overrightarrow {AC} \)

Mặt khác: \(\overrightarrow {AD}  = \overrightarrow b ,\;\overrightarrow {DC}  = \overrightarrow a \) nên \(\overrightarrow b  + \overrightarrow a  = \overrightarrow {AD}  + \overrightarrow {DC}  = \overrightarrow {AC} \)

Do đó \(\overrightarrow a  + \overrightarrow b  = \overrightarrow b  + \overrightarrow a \).

b) Theo câu a) ta có \(\overrightarrow a  + \overrightarrow b  = \overrightarrow {AC} \) và \(\overrightarrow {CD}  = \overrightarrow c \) nên \(\left( {\overrightarrow a  + \overrightarrow b } \right) + \overrightarrow c  = \overrightarrow {AC}  + \overrightarrow {CD}  = \overrightarrow {AD} \).

Mặt khác: \(\overrightarrow {BC}  = \overrightarrow b ,\;\overrightarrow {CD}  = \overrightarrow c \) nên \(\overrightarrow b  + \overrightarrow c  = \overrightarrow {BC}  + \overrightarrow {CD}  = \overrightarrow {BD} \)

Và \(\overrightarrow a  = \overrightarrow {AB} \) nên \(\overrightarrow a  + \left( {\overrightarrow b  + \overrightarrow c } \right) = \overrightarrow {AB}  + \overrightarrow {BD}  = \overrightarrow {AD} \)

Vậy \(\left( {\overrightarrow a  + \overrightarrow b } \right) + \overrightarrow c  = \overrightarrow a  + \left( {\overrightarrow b  + \overrightarrow c } \right)\)