3x - 6 căn bậc x -6 . phân tích đa thức thành nhân tử
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
N
1
29 tháng 8 2023
a: x^3-7x-6
=x^3-x-6x-6
=x(x-1)(x+1)-6(x+1)
=(x+1)(x^2-x-6)
=(x-3)(x+2)(x+1)
b: =2x^3+x^2-2x^2-x+6x+3
=x^2(2x+1)-x(2x+1)+3(2x+1)
=(2x+1)(x^2-x+3)
c: =2x^3-3x^2-2x^2+3x+2x-3
=x^2(2x-3)-x(2x-3)+(2x-3)
=(2x-3)(x^2-x+1)
d: =2x^3+x^2+2x^2+x+2x+1
=(2x+1)(x^2+x+1)
e: =3x^3+x^2-3x^2-x+6x+2
=(3x+1)(x^2-x+2)
f: =27x^3-9x^2-18x^2+6x+12x-4
=(3x-1)(9x^2-6x+4)
H9
HT.Phong (9A5)
CTVHS
29 tháng 8 2023
a) \(x^3-7x-6\)
\(=x^3-x-6x-6\)
\(=\left(x^3-x\right)-\left(6x+6\right)\)
\(=x\left(x^2-1\right)-6\left(x+1\right)\)
\(=x\left(x+1\right)\left(x-1\right)-6\left(x+1\right)\)
\(=\left(x+1\right)\left(x^2-x-6\right)\)
b) \(2x^3-x^2+5x+3\)
\(=2x^3+x^2-2x^2-x+6x+3\)
\(=\left(2x^3+x^2\right)-\left(2x^2+x\right)+\left(6x+3\right)\)
\(=x^2\left(2x+1\right)-x\left(2x+1\right)+3\left(2x+1\right)\)
\(=\left(x^2-x+3\right)\left(2x+1\right)\)
c) \(2x^3-5x^2+5x+1\)
\(=2x^3-3x^2-2x^2+3x+2x-3\)
\(=\left(2x^3-3x^2\right)-\left(2x^2-3x\right)+\left(2x-3\right)\)
\(=x^2\left(2x-3\right)-x\left(2x-3\right)+\left(2x-3\right)\)
\(=\left(x^2-x+1\right)\left(2x-3\right)\)
d) \(2x^3+3x^2+3x+1\)
\(=2x^3+x^2+2x^2+x+2x+1\)
\(=\left(2x^3+x^2\right)+\left(2x^2+x\right)+\left(2x+1\right)\)
\(=x^2\left(2x+1\right)+x\left(2x+1\right)+\left(2x+1\right)\)
\(=\left(2x+1\right)\left(x^2+x+1\right)\)
e) \(3x^3-2x^2+5x+2\)
\(=3x^3+x^2-3x^2-x+6x+2\)
\(=\left(3x^3+x^2\right)-\left(3x^2+x\right)+\left(6x+2\right)\)
\(=x^2\left(3x+1\right)-x\left(3x+1\right)+2\left(3x+1\right)\)
\(=\left(3x-1\right)\left(x^2-x+2\right)\)
f) \(27x^3-27x^2+18x-4\)
\(=27x^3-9x^2-18x^2+6x+12x-4\)
\(=\left(27x^3-9x^2\right)-\left(18x^2-6x\right)+\left(12x-4\right)\)
\(=9x^2\left(3x-1\right)-6x\left(3x-1\right)+4\left(3x-1\right)\)
\(=\left(3x-1\right)\left(9x^2-6x+4\right)\)
NH
0
PL
2
T
25 tháng 8 2023
\(3x\cdot\left(x-y\right)^2-6\cdot\left(y-x\right)\)
\(=3x\left(x-y\right)^2+6\left(x-y\right)\)
\(=\left(x-y\right)\left[3x\left(x-y\right)+6\right]\)
\(=\left(x-y\right)\left(3x^2-3xy+6\right)\)
HN
2
NN
31 tháng 10 2020
Đặt \(x^2+3x+1=t\)
\(\Rightarrow\left(x^2+3x+1\right)\left(x^2+3x+2\right)-6=t.\left(t+1\right)-6\)
\(=t^2+t-6=\left(t^2-2t\right)+\left(3t-6\right)\)
\(=t\left(t-2\right)+3\left(t-2\right)=\left(t-2\right)\left(t+3\right)\)
\(=\left(x^2+3x+1-2\right)\left(x^2+3x+1+3\right)\)
\(=\left(x^2+3x-1\right)\left(x^2+3x+4\right)\)
G
31 tháng 10 2020
\(A=\left(x^2+3x+1\right)\left(x^2+3x+2\right)-6\)
Đặt \(x^2+3x+1=a\)ta có :
\(a\left(a+1\right)-6\)
\(=a^2+a-6\)
\(=a^2+6a-a-6\)
\(=\left(a^2+6a\right)-\left(a+6\right)\)
\(=a\left(a+6\right)-\left(a+6\right)\)
\(=\left(a+6\right)\left(a-1\right)\)
Thay \(a=x^2+3x+1\)vào A ta có :
\(A=\left(x^2+3x+1+6\right)\left(x^2+3x+1-1\right)\)
\(=\left(x^2+3x+7\right)\left(x^2+3x\right)\)
NK
1
YN
27 tháng 10 2021
\(x.\left(x^2-4\right)-3x+6\)
\(=x.\left(x+2\right).\left(x-2\right)-3.\left(x-2\right)\)
\(=\left(x^2+2x\right).\left(x-2\right)-3.\left(x-2\right)\)
\(=\left(x-2\right).\left(x^2+2x-3\right)\)
\(=\left(x-2\right).\left(x^2-x+3x-3\right)\)
\(=\left(x-2\right).[x.\left(x-1\right)+3.\left(x-1\right)]\)
\(=\left(x-2\right).\left(x-1\right).\left(x+3\right)\)
BA
1
9 tháng 7 2019
\(\left(x^2+3x+1\right)\left(x^2+3x+2\right)-6\)
Đặt \(\left(x^2+3x+1\right)=a\), ta được:
\(a\left(a+1\right)-6\)\(=a^2+a-6\)\(=\left(a^2+3a\right)-\left(2a+6\right)\)\(=a\left(a+3\right)-2\left(a+3\right)\)
\(=\left(a+3\right)\left(a-2\right)\)
Thay \(a=\left(x^2+3x+1\right)\), ta được:
\(=\left(x^2+3x+1+3\right)\left(x^2+3x+1-2\right)\)
\(=\left(x^2+3x+4\right)\left(x^2+3x-1\right)\)
PT
1
CM
24 tháng 9 2019
Cách 1: Tách một hạng tử thành tổng hai hạng tử để xuất hiện nhân tử chung.
a) x2 – 3x + 2
= x2 – x – 2x + 2 (Tách –3x = – x – 2x)
= (x2 – x) – (2x – 2)
= x(x – 1) – 2(x – 1) (Có x – 1 là nhân tử chung)
= (x – 1)(x – 2)
Hoặc: x2 – 3x + 2
= x2 – 3x – 4 + 6 (Tách 2 = – 4 + 6)
= x2 – 4 – 3x + 6
= (x2 – 22) – 3(x – 2)
= (x – 2)(x + 2) – 3.(x – 2) (Xuất hiện nhân tử chung x – 2)
= (x – 2)(x + 2 – 3) = (x – 2)(x – 1)
b) x2 + x – 6
= x2 + 3x – 2x – 6 (Tách x = 3x – 2x)
= x(x + 3) – 2(x + 3) (có x + 3 là nhân tử chung)
= (x + 3)(x – 2)
c) x2 + 5x + 6 (Tách 5x = 2x + 3x)
= x2 + 2x + 3x + 6
= x(x + 2) + 3(x + 2) (Có x + 2 là nhân tử chung)
= (x + 2)(x + 3)
Cách 2: Đưa về hằng đẳng thức (1) hoặc (2)
a) x2 – 3x + 2
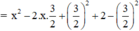
(Vì có x2 và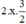 
nên ta thêm bớt 
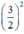để xuất hiện HĐT)
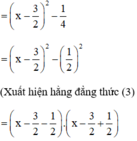
= (x – 2)(x – 1)
b) x2 + x - 6
= (x – 2)(x + 3).
c) x2 + 5x + 6
= (x + 2)(x + 3).