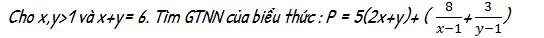
giải theo cách làm của lớp 8 giúp mình với ạ
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


5.
\(\Delta=m^2-4\left(m-1\right)=\left(m-2\right)^2\)
Pt có 2 nghiệm pb khi \(\left(m-2\right)^2>0\Rightarrow m\ne2\)
Khi đó theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=m\\x_1x_2=m-1\end{matrix}\right.\)
\(x_1^2+x_2^2=x_1+x_2\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=x_1+x_2\)
\(\Leftrightarrow m^2-2\left(m-1\right)=m\)
\(\Leftrightarrow m^2-3m+2=0\Rightarrow\left[{}\begin{matrix}m=1\\m=2\left(loại\right)\end{matrix}\right.\)
1.
\(\Delta=9+4m>0\Rightarrow m>-\dfrac{9}{4}\)
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=-3\\x_1x_2=-m\end{matrix}\right.\)
\(5x_1+5x_2=1-\left(x_1x_2\right)^2\)
\(\Leftrightarrow5\left(x_1+x_2\right)=1-\left(x_1x_2\right)^2\)
\(\Leftrightarrow5.\left(-3\right)=1-\left(-m\right)^2\)
\(\Leftrightarrow m^2=16\Rightarrow\left[{}\begin{matrix}m=4\\m=-4< -\dfrac{9}{4}\left(loại\right)\end{matrix}\right.\)
2.
\(\Delta=\left(2m+1\right)^2-4\left(m^2+1\right)=4m-3>0\Rightarrow m>\dfrac{3}{4}\)
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2m+1\\x_1x_2=m^2+1\end{matrix}\right.\)
\(\left(x_1+1\right)^2+\left(x_2+1\right)^2=13\)
\(\Leftrightarrow x_1^2+2x_1+1+x_2^2+2x_2+1=13\)
\(\Leftrightarrow x_1^2+x_2^2+2\left(x_1+x_2\right)=11\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2+2\left(x_1+x_2\right)=11\)
\(\Leftrightarrow\left(2m+1\right)^2-2\left(m^2+1\right)+2\left(2m+1\right)=11\)
\(\Leftrightarrow2m^2+8m-10=0\)
\(\Rightarrow\left[{}\begin{matrix}m=1\\m=-5< \dfrac{3}{4}\left(loại\right)\end{matrix}\right.\)

a: ΔAMN vuông tại A
mà AI là đường trung tuyến
nên AI=IM=IN=MN/2
=>I là tâm đường tròn ngoại tiếp ΔAMN
b: Xét (O) có
ΔBAC nội tiếp
BC là đường kính
Do đó: ΔBAC vuông tại A
Xét (O) có
ΔBEC nội tiếp
BC là đường kính
Do đó: ΔBEC vuông tại E

\(\dfrac{S_{AOD}}{S_{DOC}}=\dfrac{10}{20}=\dfrac{1}{2}\)
=>\(AO=\dfrac{1}{2}CO\)
Vì ABCD là hình thang có hai đáy là AB và CD
và AC cắt BD tại O
nên \(S_{AOD}=S_{BOC}\)
=>\(S_{BOC}=10\left(cm^2\right)\)
\(AO=\dfrac{1}{2}OC\)
=>\(S_{AOB}=\dfrac{1}{2}\cdot S_{BOC}=5\left(cm^2\right)\)
\(S_{ABCD}=S_{ABO}+S_{BOC}+S_{DOC}+S_{AOD}\)
\(=5+10+20+10=45\left(cm^2\right)\)

\(\dfrac{13}{2}\) : 4\(\dfrac{2}{3}\): 2
= \(\dfrac{13}{2}\): \(\dfrac{14}{3}\):2
= \(\dfrac{13}{2}\) \(\times\) \(\dfrac{3}{14}\):2
= \(\dfrac{39}{28}\) : 2
= \(\dfrac{39}{28}\) \(\times\) \(\dfrac{1}{2}\)
= \(\dfrac{39}{56}\)

Bài 3:
Nếu đáy lớn được tăng thêm 5 cm thì diện tích sẽ tăng một phần bằng 5 x chiều cao : 2
Vậy chiều cao của hình thang là:
$20\times 2:5=2$ (m)
Diện tích hình thang ban đầu là:
$50\times 2:2=50$ (m2)

`@` `\text {Ans}`
`\downarrow`
`a)`
\(\left(\dfrac{x}{2}-1\right)^3+2=-\dfrac{11}{8}\) phải k bạn nhỉ? `11/8` k có bậc lũy thừa nào `=5` á.
`=>`\(\left(\dfrac{x}{2}-1\right)^3=-\dfrac{11}{8}-2\)
`=>`\(\left(\dfrac{x}{2}-1\right)^3=-\dfrac{27}{8}\)
`=>`\(\left(\dfrac{x}{2}-1\right)^3=\left(-\dfrac{3}{2}\right)^3\)
`=>`\(\dfrac{x}{2}-1=-\dfrac{3}{2}\)
`=>`\(\dfrac{x}{2}=-\dfrac{3}{2}+1\)
`=>`\(\dfrac{x}{2}=-\dfrac{1}{2}\)
`=> x=1`
Vậy, `x=1`
`b)`
\(\left(\dfrac{x}{3}+\dfrac{1}{2}\right)\left(75\%-1\dfrac{1}{2}\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}\dfrac{x}{3}+\dfrac{1}{2}=0\\0,75-1\dfrac{1}{2}x=0\end{matrix}\right.\)
`=>`\(\left[{}\begin{matrix}\dfrac{x}{3}=-\dfrac{1}{2}\\-\dfrac{3}{2}x=\dfrac{75}{100}\end{matrix}\right.\)
`=>`\(\left[{}\begin{matrix}2x=-3\\-3x\cdot100=2\cdot75\end{matrix}\right.\)
`=>`\(\left[{}\begin{matrix}x=-\dfrac{3}{2}\\-3x\cdot100=150\end{matrix}\right.\)
`=>`\(\left[{}\begin{matrix}x=-\dfrac{3}{2}\\-3x=1,5\end{matrix}\right.\)
`=>`\(\left[{}\begin{matrix}x=-\dfrac{3}{2}\\x=-\dfrac{1}{2}\end{matrix}\right.\)
Vậy, `x={-3/2; -1/2}.`

a-b=7
(a-b)2=a2+b2-2ab=49
a2+b2+4ab-2ab=49+32=81
(a+b)2=81
a+b=9 hoặc a+b=(-9)

ta có \(x\in\left[-\frac{\pi}{4};0\right]\Rightarrow2x\in\left[-\frac{\pi}{2},0\right]\Rightarrow sin2x\in\left[-1,0\right]\)
Vậy \(\hept{\begin{cases}GTNN=-1\\GTLN=0\end{cases}}\)

\(\dfrac{x+2}{x-3}< 0\)vì \(x+2>x-3\)
\(\left\{{}\begin{matrix}x+2>0\\x-3< 0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x>-2\\x< 3\end{matrix}\right.\)<=> -2 < x < 3
mình đang cần gấp, cảm ơn trước ạ :(