A= (2m-1)2
Tìm giá trị nhỏ nhất của A
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
HP
6 tháng 5 2022
\(A=m^2-2m-5\)
\(=m^2-2m+1-6\)
\(=\left(m-1\right)^2-6\ge-6\)
Dấu '' = '' xảy ra khi \(\left(m-1\right)^2=0\Leftrightarrow m=1\)
Vậy \(Min_A=-6\) khi \(m=1\)
3 tháng 8 2021
\(\Delta=4m^2-4m+1-4\left(2m-2\right)=4m^2-12m+9=\left(2m-3\right)^2\ge0\)
Do đó pt luôn có nghiệm
Theo định lí Vi-ét:
\(\left\{{}\begin{matrix}x_1+x_2=2m-1\\x_1x_2=2m-2\end{matrix}\right.\)
Lại có: \(A=x_1^2+x_2^2=\left(x_1+x_2\right)^2-2x_1x_2\)
\(A=\left(2m-1\right)^2-2\left(2m-2\right)\)
\(A=4m^2-4m+1-4m+4\)
\(A=4m^2-8m+5\)
\(A=4\left(m-1\right)^2+1\ge1\)
Dấu "=" xảy ra \(\Leftrightarrow\) m=1
Tick hộ nha 😘
3 tháng 8 2021
pt có nghiệm \(< =>\Delta\ge0\)
\(< =>[-\left(2m-1\right)]^2-4\left(2m-2\right)\ge0\)
\(< =>4m^2-4m+1-8m+8\ge0\)
\(< =>4m^2-12m+9\ge0\)
\(< =>4\left(m^2-3m+\dfrac{9}{4}\right)\ge0\)
\(=>m^2-2.\dfrac{3}{2}m+\dfrac{9}{4}\ge0< =>\left(m-\dfrac{2}{3}\right)^2\ge0\)(luôn đúng)
=>pt luôn có 2 nghiệm
theo vi ét \(=>\left\{{}\begin{matrix}x1+x2=2m-1\\x1x2=2m-2\end{matrix}\right.\)
\(A=\left(x1+x2\right)^2-2x1x2=\left(2m-1\right)^2-2\left(2m-2\right)\)
\(A=4m^2-4m+1-4m+4=4m^2+5\ge5\)
dấu"=" xảy ra<=>m=0
DT
1
DD
Đoàn Đức Hà
Giáo viên
9 tháng 3 2021
\(A=\frac{2m+1}{m^2+2}\Leftrightarrow A\left(m^2+2\right)=2m+1\Leftrightarrow Am^2-2m+2A-1=0\)
Ta coi đây là phương trình ẩn \(m\)với \(A\)là tham số.
- Với \(A=0\): \(-2m-1=0\Leftrightarrow m=-\frac{1}{2}\).
- Với \(A\ne0\): phương trình có nghiệm khi:
\(\Delta'=1-A\left(2A-1\right)=-2A^2+A+1\ge0\)
\(\Leftrightarrow\frac{-1}{2}\le A\le1\).
Kết hợp cả hai trường hợp ta có \(minA=-\frac{1}{2},maxA=1\).
CM
12 tháng 2 2017
Đáp án đúng : C
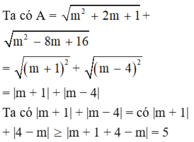
Dấu “=” xảy ra ⇔ m + 1 4 − m ≥ 0
⇔ − 1 ≤ m ≤ 4
Vậy GTNN của A là 5 khi − 1 ≤ m ≤ 4
6 tháng 11 2021
\(B=2\left(m^2-m+\dfrac{1}{4}\right)+\dfrac{9}{2}=2\left(m-\dfrac{1}{2}\right)^2+\dfrac{9}{2}\ge\dfrac{9}{2}\)
\(minB=\dfrac{9}{2}\Leftrightarrow m=\dfrac{1}{2}\)
NV
Nguyễn Việt Lâm
Giáo viên
25 tháng 3 2022
\(\Delta'=\left(m+1\right)^2-2m-10=m^2-9\ge0\Rightarrow\left[{}\begin{matrix}m\ge3\\m\le-3\end{matrix}\right.\)
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2\left(m+1\right)\\x_1x_2=2m+10\end{matrix}\right.\)
a.
\(P=x_1^2+x_2^2+6x_1x_2=\left(x_1+x_2\right)^2+4x_1x_2\)
\(P=4\left(m+1\right)^2+4\left(2m+10\right)\)
\(P=4m^2+16m+44=\left(4m^2+16m+12\right)+32\)
\(P=4\left(m+1\right)\left(m+3\right)+32\ge32\)
\(P_{min}=32\) khi \(m=-3\)
b.
Theo Viet: \(\left\{{}\begin{matrix}x_1+x_2=2m+2\\x_1x_2=2m+10\end{matrix}\right.\)
Trừ vế cho vế:
\(x_1+x_2-x_1x_2=-8\)
Đây là hệ thức liên hệ 2 nghiệm ko phụ thuộc m
dấu = xảy ra <=> 2m-1=0 <=> m=1/2