tìm a,b ϵ N sao cho 9/56<a/8<b/8<13/28
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
LD
1
K
0
AH
Akai Haruma
Giáo viên
13 tháng 1 2024
Lời giải:
$A=\frac{2023a+b}{2023a-b}=\frac{(2023a-b)+2b}{2023a-b}$
$=1+\frac{2b}{2023a-b}=1+\frac{2}{2023\frac{a}{b}-1}$
Để $A$ nhỏ nhất thì $\frac{2}{2023.\frac{a}{b}-1}$ nhỏ nhất, tức là $2023\frac{a}{b}-1$ lớn nhất, hay $\frac{a}{b}$ lớn nhất.
Với điều kiện $1\leq a\leq b\leq 9$ và $a,b$ là số tự nhiên thì $\frac{a}{b}$ lớn nhất khi mà $a=b$
Khi đó: $A_{\max}=\frac{2023a+a}{2023a-a}=\frac{2024}{2022}=\frac{1012}{1011}$
KV
29 tháng 7 2023
a) D = {2; 7; 12; ...; 82; 87}
Số phần tử của D:
(87 - 2) : 5 + 1 = 18 (phần tử)
b) x - 15 = 37
x = 37 + 15
x = 52
E = {52}
Số phần tử của E là 1
c) a . 6 = 4
a = 4 : 6
a = 2/3 (loại vì a ∈ ℕ)
F = ∅
Vậy F không có phần tử nào
29 tháng 7 2023
a) D = { 2 ; 7 ; 12 ; 17 ; 22 ; 27 ; 32 ; 37 ; 42 ; 47 ; 52 ; 57 ; 62 ; 67 ; 72 ; 77 ; 82 ; 87 }
b) E = { 52 }
c) F = { \(\varnothing\) }
- HokTot -
LT
0
7 tháng 8 2023
a) \(25⋮n+2\left(n\in Z\right)\)
\(\Rightarrow n+2\in\left\{-1;1;-5;5;-25;25\right\}\)
\(\Rightarrow n\in\left\{-3;-1;-7;3;-27;23\right\}\)
b) \(2n+4⋮n-1\)
\(\Rightarrow2n+4-2\left(n-1\right)⋮n-1\)
\(\Rightarrow2n+4-2n+2⋮n-1\)
\(\Rightarrow6⋮n-1\)
\(\Rightarrow n-1\in\left\{-1;1;-2;2;-3;3;-6;6\right\}\)
\(\Rightarrow n\in\left\{0;2;-1;3;-2;4;-5;7\right\}\)
c) \(1-4n⋮n+3\)
\(\Rightarrow1-4n+4\left(n+3\right)⋮n+3\)
\(\Rightarrow1-4n+4n+12⋮n+3\)
\(\Rightarrow13⋮n+3\)
\(\Rightarrow n+3\in\left\{-1;1;-13;13\right\}\)
\(\Rightarrow n\in\left\{-4;-2;-15;10\right\}\)
DT
7 tháng 8 2023
a) n ϵ{−3;−1;−7;3;−27;23}
b) n ∈{0;2;−1;3;−2;4;−5;7}
c) n ϵ {−4;−2;−15;10}
9 tháng 9 2023
a) \(\left\{{}\begin{matrix}UCLN\left(a;b\right)=64\\a+b=256\left(1\right)\end{matrix}\right.\) \(\left(a;b\inℕ^∗\right)\)
Nên ta đặt \(\left\{{}\begin{matrix}a=64x\\b=64y\end{matrix}\right.\) \(\left(x;y\inℕ^∗\right)\)
\(\left(1\right)\Rightarrow64x+64y=256\)
\(\Rightarrow64\left(x+y\right)=256\)
\(\Rightarrow x+y=4\)
\(\Rightarrow\left\{{}\begin{matrix}x=1\\y=3\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}a=1.64=64\\b=3.64=192\end{matrix}\right.\) \(\left(thỏa.vì.a+b=256\right)\)
Vậy \(\left(a;b\right)=\left(64;192\right)\)
b) \(\left\{{}\begin{matrix}UCLN\left(a;b\right)=48\\a+b=13824\left(1\right)\end{matrix}\right.\) \(\left(a;b\inℕ^∗\right)\)
Nên ta đặt \(\left\{{}\begin{matrix}a=48x\\b=48y\end{matrix}\right.\) \(\left(x;y\inℕ^∗\right)\)
\(\left(1\right)\Rightarrow48x+48y=13824\)
\(\Rightarrow48\left(x+y\right)=13824\)
\(\Rightarrow x+y=288\)
\(\Rightarrow\left\{{}\begin{matrix}x=200\\y=88\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}a=48.200=9600\\b=48.88=4224\end{matrix}\right.\) \(\left(thỏa.vì.a+b=13824\right)\)
Vậy \(\left(a;b\right)=\left(9600;4224\right)\)
TV
9 tháng 9 2023
b,Theo bài ra ta có:
a + b =13824
ƯCLN (a,b)=48
*Vì ƯCLN (a,b) =48 => a=48x (x < y, ƯCLN (x,y ) = 1)
b=48y
*Mà a + b = 13824
=> 48x + 48y = 13824
48(x + y) = 13824 : 48
x + y = 288
*Ta phải tìm hai số x,y thỏa mãn các điều kiện :
x < y
UCLN (x,y) = 1
x + y =4
=>Với x=1 thì y=3
Lập bảng:
x=1
y=3
a=288 . 1 = 288 thuộc N
b=288 . 3 = 864 thuộc N
Vậy a=288,b=864.
a,Theo bài ra ta có:
a + b =256
ƯCLN (a,b)=64
*Vì ƯCLN (a,b) =64 => a=64x (x < y, ƯCLN (x,y ) = 1)
b=64y
*Mà a + b = 256
=> 64x + 64y = 256
64(x + y) = 256 : 64
x + y = 4
*Ta phải tìm hai số x,y thỏa mãn các điều kiện :
x < y
UCLN (x,y) = 1
x + y =4
=>Với x=1 thì y=3
Lập bảng:
x=1
y=3
a=18 . 1 = 18 thuộc N
b=18 . 3 = 54 thuộc N
Vậy a=18,b=54.
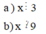
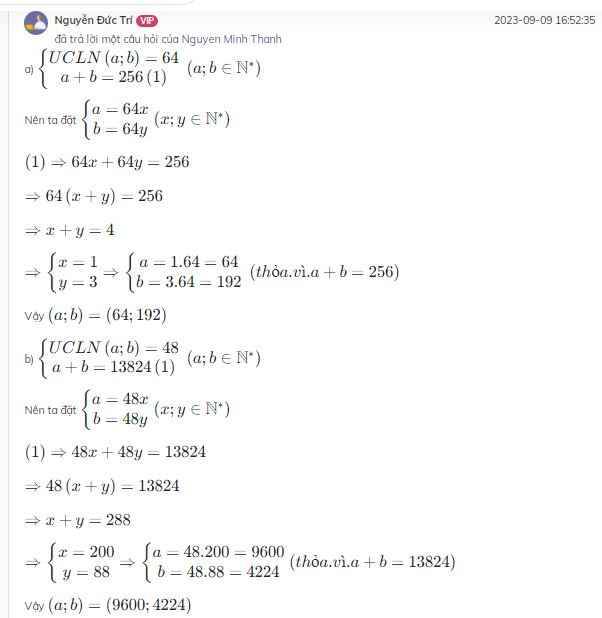
\(\dfrac{9}{56}\) < \(\dfrac{a}{8}\) < \(\dfrac{b}{8}\) < \(\dfrac{13}{28}\) (a; b \(\in\) N)
\(\dfrac{9}{56}\) < \(\dfrac{7a}{56}\) < \(\dfrac{7b}{56}\) < \(\dfrac{26}{56}\)
9 < 7a < 7b < 26
\(\dfrac{9}{7}\) < a < b < \(\dfrac{26}{7}\)
1,286 < a < b < 3,7
vì a < b , a, b \(\in\) N
a = 2; b = 3