2. Cho pt : 3x^2 - mx - m - 3 =0 M ? Pt có 2 No trái dấu và 2x1 - 3x2 = 5
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
AN
2
19 tháng 2 2021
- Để phương trình trên có hai nghiệm trái dấu
\(\Leftrightarrow ac< 0\)
\(\Leftrightarrow m\left(m-4\right)< 0\)
\(\Leftrightarrow m^2-4m< 0\)
\(\Leftrightarrow0< m< 4\)
Vậy ...
19 tháng 2 2021
Phương trình có 2 nghiệm trái dấu khi $ac<0$ hay \(m\left( {m - 4} \right) < 0 \Leftrightarrow 0 < m < 4\)
BT
2 tháng 6 2019
chủ yếu là hỏi câu c hả? tớ làm mỗi đoạn đưa về tổng - tích thôi, bạn giải thấy khó chỗ nào thì hỏi cụ thể nhe ^^
\(\left(x_1+2x_2\right)\left(x_2+2x_1\right)=x_1x_2+2x_2^2+2x_1^2+4x_1x_2=2\left(x_1+x_2\right)^2-4x_1x_2+5x_1x_2\)
đến đây Vi-ét đc òi
2 tháng 6 2019
Gotcha Tokoyami
Có \(\Delta=\left(m-2\right)^2-4\left(-m^2+3m-4\right)\)
\(=m^2-4m+4+4m^2-12m+16\)
\(=5m^2-16m+20\)
\(=5\left(m^2-\frac{16}{5}m+4\right)\)
\(=5\left[\left(m^2-2.\frac{8}{5}m+\frac{64}{25}\right)+\frac{36}{25}\right]\)
\(=5\left[\left(m-\frac{8}{5}\right)^2+\frac{36}{25}\right]>0\forall m\)
Nên pt có 2 nghiệm phân biệt với mọi m
a, Với m = 0 thì pt trở thành
\(x^2+2x-4=0\)
Có \(\Delta'=1+4=5>0\)
\(\Rightarrow\orbr{\begin{cases}x=-1+\sqrt{5}\\x=-1-\sqrt{5}\end{cases}}\)
b, Theo hệ thức Vi-et \(x_1x_2=-m^2+3m-4=-\left(m-\frac{3}{2}\right)^2-\frac{7}{4}< 0\)
nên pt có 2 nghiệm trái dấu
c, Thiếu đề , nhưng làm hộ 1 bước biến đổi như bạn dưới
HV
1
Y
9 tháng 3 2023
\(a,2x^2+12x-15m\)
Để pt có 2 nghiệm trái dấu thì \(\dfrac{c}{a}< 0\)
\(\Rightarrow\dfrac{-15m}{2}< 0\)
\(\Rightarrow-15m< 0\)
\(\Rightarrow m>0\)
Vậy để pt trên có 2 nghiệm trái dấu thì \(m>0\)
\(b,mx^2-2\left(m-2\right)x-3=0\)
Để pt có 2 nghiệm trái dấu thì \(\dfrac{c}{a}< 0\) và \(a\ne0\)
\(\left\{{}\begin{matrix}\dfrac{c}{a}< 0\\a\ne0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}-\dfrac{3}{m}< 0\\m\ne0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}-3< 0\left(LD\right)\\m\ne0\end{matrix}\right.\)
Vậy để pt trên có 2 nghiệm trái dấu thì \(m\ne0\)
NV
Nguyễn Việt Lâm
Giáo viên
15 tháng 2 2022
3.
Phương trình có 2 nghiệm khi:
\(\left\{{}\begin{matrix}m+1\ne0\\\Delta=m^2-12\left(m+1\right)\ge0\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}m\ne-1\\\left[{}\begin{matrix}m\ge6+4\sqrt{3}\\m\le6-4\sqrt{3}\end{matrix}\right.\end{matrix}\right.\) (1)
Khi đó theo Viet: \(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{m}{m+1}\\x_1x_2=\dfrac{3}{m+1}\end{matrix}\right.\)
Hai nghiệm cùng lớn hơn -1 \(\Rightarrow-1< x_1\le x_2\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left(x_1+1\right)\left(x_2+1\right)>0\\\dfrac{x_1+x_2}{2}>-1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_1x_2+x_1+x_1+1>0\\x_1+x_2>-2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{3}{m+1}-\dfrac{m}{m+1}+1>0\\-\dfrac{m}{m+1}>-2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{4}{m+1}>0\\\dfrac{m+2}{m+1}>0\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}m>-1\\\left[{}\begin{matrix}m>-1\\m< -2\end{matrix}\right.\end{matrix}\right.\) \(\Rightarrow m>-1\)
Kết hợp (1) \(\Rightarrow\left[{}\begin{matrix}-1< m< 6-4\sqrt{3}\\m\ge6+4\sqrt{3}\end{matrix}\right.\)
Những bài này đều là dạng toán lớp 10, thi lớp 9 chắc chắn sẽ không gặp phải
NV
Nguyễn Việt Lâm
Giáo viên
15 tháng 2 2022
1. Có 2 cách giải:
C1: đặt \(f\left(x\right)=x^2+2mx-3m^2\)
\(x_1< 1< x_2\Leftrightarrow1.f\left(1\right)< 0\Leftrightarrow1+2m-3m^2< 0\Rightarrow\left[{}\begin{matrix}m>1\\m< -\dfrac{1}{3}\end{matrix}\right.\)
C2: \(\Delta'=4m^2\ge0\) nên pt luôn có 2 nghiệm
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=-2m\\x_1x_2=-3m^2\end{matrix}\right.\)
\(x_1< 1< x_2\Leftrightarrow\left(x_1-1\right)\left(x_2-1\right)< 0\)
\(\Leftrightarrow x_1x_2-\left(x_1+x_2\right)+1< 0\)
\(\Leftrightarrow-3m^2+2m+1< 0\Rightarrow\left[{}\begin{matrix}m>1\\m< -\dfrac{1}{3}\end{matrix}\right.\)
HT
7 tháng 7 2015
a) Thay \(m=-5\) vào PT ta được:
\(x^2-\left(-5\right)x+2.\left(-5\right)-3=0\)
\(\Rightarrow x^2+5x-10-3=0\)
\(\Rightarrow x^2+5x-13=0\)
\(\Delta=5^2-4.1.\left(-13\right)=25+52=77>0\)
PT có 2 nghiệm phân biệt:
\(x_1=-\frac{5+\sqrt{77}}{2}\)
\(x_2=-\frac{5-\sqrt{77}}{2}\)
Vậy với m = -5 thì PT có nghiệm là \(S=\left\{-\frac{5+\sqrt{77}}{2};-\frac{5-\sqrt{77}}{2}\right\}\)
b) PT có nghiệm kép \(\Leftrightarrow\Delta=0\Leftrightarrow\left(-m\right)^2-4.1.\left(2m-3\right)=0\)
\(\Leftrightarrow m^2-8m+12=0\Leftrightarrow\left(m-2\right)\left(m-6\right)=0\)
\(\Leftrightarrow\int^{m-2=0}_{m-6=0}\Leftrightarrow\int^{m=2}_{m=6}\)
Vậy với m = 2 và m = 6 thì PT có nghiệm kép.
c) PT có 2 nghiệm trái dấu \(\Leftrightarrow\int^{\Delta>0}_{2m-36}_{m0\Leftrightarrow m>6\)
TT
1
NV
Nguyễn Việt Lâm
Giáo viên
26 tháng 3 2022
Pt có 2 nghiệm khi: \(\Delta=25-8\left(m+1\right)\ge0\Rightarrow m\le\dfrac{17}{8}\)
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=\dfrac{5}{2}\\x_1x_2=\dfrac{m+1}{2}\end{matrix}\right.\)
Kết hợp Viet và điều kiện đề bài: \(\left\{{}\begin{matrix}x_1+x_2=\dfrac{5}{2}\\2x_1+3x_2=4\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x_1=\dfrac{7}{2}\\x_1=-1\end{matrix}\right.\)
Thế vào \(x_1x_2=\dfrac{m+1}{2}\Rightarrow\dfrac{m+1}{2}=-\dfrac{7}{2}\)
\(\Rightarrow m=-8\)
LA
25 tháng 4 2022
xét delta phẩy có
1+1-m = 2-m vậy điều kiện để phương trình có 2 nghiệm x1;x2 là m ≤2
theo Vi-ét ta có:
\(\left\{{}\begin{matrix}x1+x2=2\\x1x2=m-1\end{matrix}\right.\)
theo bài ra ta có:
2x1 + x2 = 5
x1 + 2 = 5 => x1 = 3 => x2 = -1
ta có x1x2 = m - 1 => m - 1 = -3
=> m = -2 vậy m = -2 để phương trình có 2 nghiệm x1;x2 thỏa mãn 2x1 + x2 = 5.
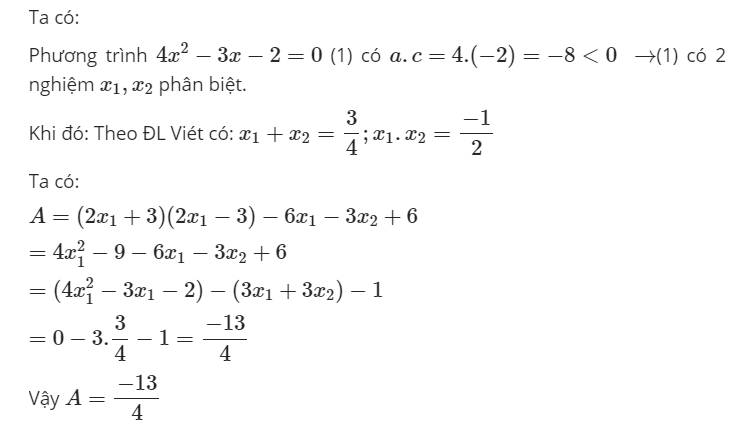
Pt có 2 nghiệm trái dấu khi \(3\left(-m-3\right)< 0\)
\(\Rightarrow m>-3\)
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=\dfrac{m}{3}\\x_1x_2=\dfrac{-m-3}{3}\end{matrix}\right.\)
Kết hợp điều kiện đề bài ta được:
\(\left\{{}\begin{matrix}x_1+x_2=\dfrac{m}{3}\\2x_1-3x_2=5\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}3x_1+3x_2=m\\2x_1-3x_2=5\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x_1=\dfrac{m+5}{5}\\x_2=\dfrac{m}{3}-x_1=\dfrac{2m-15}{15}\end{matrix}\right.\)
Thế vào \(x_1x_2=\dfrac{-m-3}{3}\)
\(\Rightarrow\left(\dfrac{m+5}{5}\right)\left(\dfrac{2m-15}{15}\right)=\dfrac{-m-3}{3}\)
\(\Leftrightarrow2m^2+20m=0\Rightarrow\left[{}\begin{matrix}m=0\\m=-10\left(loại\right)\end{matrix}\right.\)