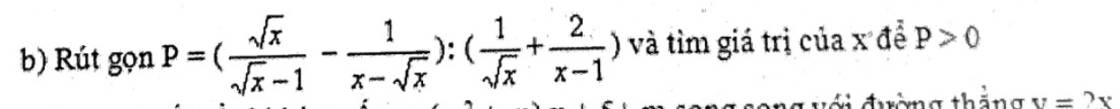Question

[Nam Trân]

Tìm giúp em giá trị của x ạ. E xin cảm ơn

Answer 1

[YangSu]

\(P=\left(\dfrac{\sqrt{x}}{\sqrt{x}-1}-\dfrac{1}{x-\sqrt{x}}\right):\left(\dfrac{1}{\sqrt{x}}+\dfrac{2}{x-1}\right)\)

\(=\left(\dfrac{\sqrt{x}}{\sqrt{x}-1}-\dfrac{1}{\sqrt{x}\left(\sqrt{x}-1\right)}\right):\left(\dfrac{1}{\sqrt{x}}+\dfrac{2}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\right)\)

\(=\dfrac{x-1}{\sqrt{x}\left(\sqrt{x}-1\right)}:\dfrac{x-1+2\sqrt{x}}{\sqrt{x}\left(x-1\right)}\)

\(=\dfrac{x-1}{\sqrt{x}\left(\sqrt{x}-1\right)}.\dfrac{\sqrt{x}\left(\sqrt{x}-1\right)}{x-1+2\sqrt{x}}\)

\(=\dfrac{x-1}{x-1+2\sqrt{x}}\)

Để \(P>0\)

\(\Rightarrow\dfrac{x-1}{x-1+2\sqrt{x}}>0\)

\(TH_1:x-1>0\Leftrightarrow x>1\)

\(TH_2:x-1+2\sqrt{x}>0\Leftrightarrow\left(\sqrt{x}+1\right)^2< 2\)

\(\Leftrightarrow-\sqrt{2}-1< \sqrt{x}< \sqrt{2}-1\)

\(\Leftrightarrow0< x< 3-2\sqrt{2}\)

Answer 2

[Nguyễn Lê Phước Thịnh]

\(=\dfrac{x-1}{\sqrt{x}\left(\sqrt{x}-1\right)}:\dfrac{x-1+2\sqrt{x}}{\sqrt{x}\left(x-1\right)}\)

\(=\dfrac{\sqrt{x}+1}{\sqrt{x}}\cdot\dfrac{\sqrt{x}\left(x-1\right)}{x+2\sqrt{x}-1}\)

\(=\dfrac{\left(x-1\right)\left(\sqrt{x}+1\right)}{x+2\sqrt{x}-1}\)

Để P>0 thì (x-1)/(x+2căn x-1)>0

TH1: x-1>0 và x+2căn x-1>0

=>x>1

TH2: x-1<0 và x+2căn x-1<0

=>0<x<1 và (căn x+1)^2<2

=>0<x<1và \(-\sqrt{2}< \sqrt{x}+1< \sqrt{2}\)

=>\(\left\{{}\begin{matrix}0< x< 1\\-\sqrt{2}-1< \sqrt{x}< \sqrt{2}-1\end{matrix}\right.\Leftrightarrow0< x< 3-2\sqrt{2}\)