Chứng minh rằng tam giác ABC vẽ trên giấy kẻ ô vuông như hình 61 là tam giác nhọn ( tức là tam giác có cả ba góc đều là góc nhọn )
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
PT
1
CM
4 tháng 11 2017
Nối A với D tạo thành đường chéo ô vuông
Gọi K giao điểm AC với đỉnh ô vuông, H là giao điểm DK với đường kẻ ngang ô vuông đi qua A. ( như hình vẽ)
Ta có: ΔAHK vuông cân tại H =>∠HAK =45o
ΔAHD vuông cân tại H=>∠HAD =45o
=>∠DAK =∠HAK +∠HAD =45o+45o=90o
hay ∠DAC =90o
=>∠BAC <90o
Hình vuông có 4 góc, mỗi góc bằng 900. Từ hình vẽ suy ra: ∠ACB <90o và ∠ABC <90o
Vậy tam giác ABC là tam giác nhọn
15 tháng 6 2022
https://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.htmlhttps://olm.vn/hoi-dap/detail/239868952049.html
9 tháng 9 2023
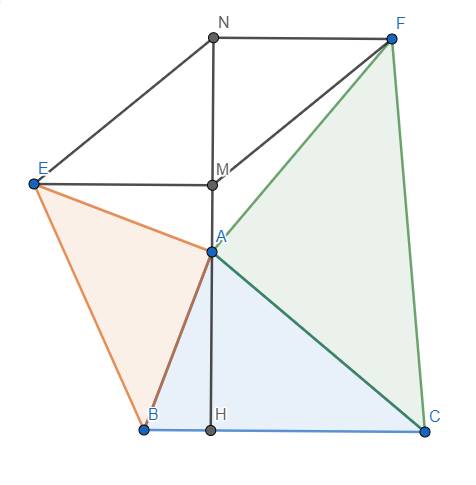
a) Do tam giác AEB vuông cân tại A nên \(\left\{{}\begin{matrix}\widehat{EAB}=90^o\\AE=AB\end{matrix}\right.\)
Ta thấy \(\widehat{MEA}=\widehat{BAH}\) vì chúng cùng phụ với \(\widehat{EAM}\)
Xét 2 tam giác HAB vuông tại H và MEA vuông tại M, ta có:
\(AE=AB\left(cmt\right),\widehat{MEA}=\widehat{BAH}\left(cmt\right)\)
\(\Rightarrow\Delta HAB=\Delta MEA\left(ch-gn\right)\) \(\Rightarrow AH=ME\) (1)
Tương tự, ta cũng có \(\Delta HAC=\Delta NFA\Rightarrow HC=AN\) (2)
Từ (1) và (2) suy ra \(EM+HC=AH+AN\) hay \(EM+HC=HN\) (đpcm)
b) Từ \(\Delta HAC=\Delta NFA\Rightarrow AH=NF\)
Từ đó suy ra \(ME=NF\left(=AH\right)\)
Xét tam giác MNE và NMF, ta có:
\(ME=NF\left(cmt\right),\widehat{EMN}=\widehat{FNM}\left(=90^o\right)\), MN là cạnh chung.
\(\Rightarrow\Delta MNE=\Delta NMF\left(c.g.c\right)\)
\(\Rightarrow\widehat{ENM}=\widehat{FMN}\) \(\Rightarrow\) EN//FM (2 góc so le trong bằng nhau)
Ta có đpcm.
SG
1
1 tháng 6 2017
Giả sử độ dài mỗi ô vuông nhỏ là 1
Đường chéo mỗi ô vuông là Căn 2.
Độ dài các cạnh AB, AC, BC lần lượt là: ( căn 13) , 3 căn 2, 5
Ta thấy 3 cạnh không bằng nhau nên không phải tam giác đều.
Thử định lý pytago đảo không đúng nên không phải tam giác vuông.
So sánh tỉ lện giữ cách cạnh đều nhỏ hơn 2. Nên trong tam giác không có góc tù. Vậy tam giác là tam giác nhọn
PT
1
CM
13 tháng 1 2019
Đặt độ dài cạnh ô vuông là 1 (đơn vị chiều dài)
Áp dụng định lí pitago ta có:
AB2=12+22=1+4=5
BC2=12+22=1+4=5
AC2=32+12=9+1=10
Suy ra: AC2=AB2+BC2
Áp dụng định lí pitago đảo ta có tam giác ABC vuông tại B
Lại có: AB2=BC2=5 suy ra: AB = BC. Do đó, tam giác ABC là tam giác cân tại B.
Vậy tam giác ABC vuông cân tại B
CH
Cô Hoàng Huyền
Admin
VIP
28 tháng 2 2018
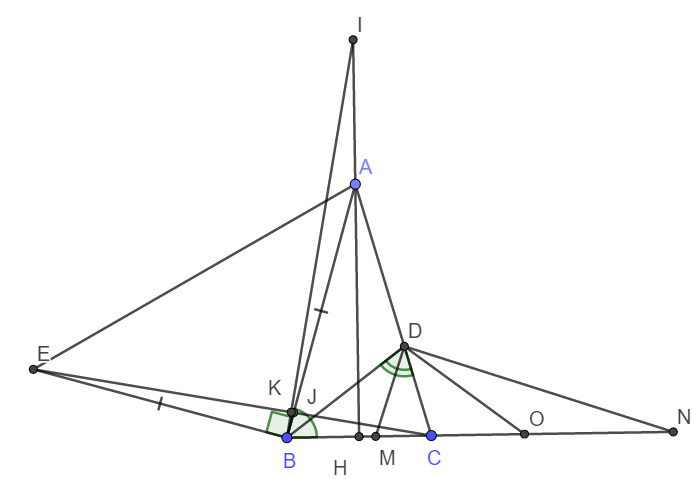
a)
+) Do tam giác ABC cân tại A nên trung tuyến AH đồng thời là đường caio.
Vậy nên \(\widehat{AHB}=90^o\)
Theo tính chất góc ngoài của tam giác, ta có:
\(\widehat{IAB}=\widehat{AHB}+\widehat{HBA}=90^o+\widehat{HBA}=\widehat{EBA}+\widehat{HBA}=\widehat{CBE}\)
Xét tam giác ABI và tam giác BEC có:
AI = BC (gt)
BA = EB (gt)
\(\widehat{IAB}=\widehat{CBE}\) (cmt)
\(\Rightarrow\Delta ABI=\Delta BEC\left(c-g-c\right)\)
+) Gọi giao điểm của EC với AB và BI lần lượt là J và K.
Do \(\Delta ABI=\Delta BEC\Rightarrow\widehat{KBJ}=\widehat{BEK}\)
Vậy thì \(\widehat{KBJ}+\widehat{KJB}=\widehat{BEK}+\widehat{KJB}=90^o\)
Suy ra \(\widehat{BKJ}=90^o\) hay \(BI\perp CE\)
b) Gọi O là trung điểm MN. Ta thấy DN và DM là phân giác của hai góc kề bù nên chúng vuông góc với nhau.
Vậy tam giác DMN vuông tại D. Khi đó ta có DO là trung tuyến ứng với cạnh huyền nên DO = MN/2
Vậy DO = OM = OM hay các tam giác DOM và DON cân tại O.
Ta có: \(\widehat{DOM}=180^o-2\widehat{DMO}=180^o-2\left(\widehat{MDB}+\widehat{MBD}\right)\)
\(=180^o-2.\widehat{MDB}-2.\widehat{MBD}=180^o-\widehat{BDC}-\widehat{ABC}\)
\(=180^o-\widehat{BDC}-\widehat{ACB}=\widehat{DBO}\)
Vậy tam giác DBO cân tại D hay DB = DO.
Vậy nên BD = MN/2.
25 tháng 8 2018
xét tam giác BAI va CBE
be=ab
bc=ia
iab=ebc
=>tam giác BAI=tam giác CBE
CH
Cô Hoàng Huyền
Admin
VIP
28 tháng 2 2018
Em tham khảo tại đây nhé.
Câu hỏi của Nguyễn Minh Huy - Toán lớp 7 - Học toán với OnlineMath
17 tháng 7 2017
a) Vẽ tia đối của BC là Bx. Gọi giao điểm của BI và CE là M. CE giao AB tại N.
\(\Delta\)ABC cân tại A. H là trung điểm của BC => AH là đường cao của \(\Delta\)ABC => AH\(⊥\)BC.
Ta có: ^ABH+^EBx=1800-^ABE=900 (1)
\(\Delta\)AHB vuông tại H => ^ABH+^BAH=900 (2)
Từ (1) và (2) => ^EBx=^BAH => 1800-^EBx=1800-^BAH => ^EBC=^BAI
Xét \(\Delta\)ABI và \(\Delta\)BEC:
AB=BE
^BAI=^EBC => \(\Delta\)ABI=\(\Delta\)BEC (c.g.c) (đpcm)
AI=BC
=> ^BEC=^ABI (2 góc tương ứng) hay ^BEN=^NBM.
\(\Delta\)EBN vuông tại B => ^BEN+^BNE=900. Thay ^BEN=^NBM, ta được:
^NBM+^BNE=900 hay ^NBM+^BNM=900. Xét \(\Delta\)BMN có:
^NBM+^BNM=900 => ^BMN=900 => BI\(⊥\)CE tại M (đpcm).
Hình 61 là hình nào ?
làm gì có hình 61 nào?