Giải pt giúp mình câu d ạ
giúp mình câu d ạ
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(b,B=\dfrac{\sqrt{x}+2}{\sqrt{x}-3}+\dfrac{\sqrt{x}-8}{x-5\sqrt{x}+6}\left(x\ge0;x\ne4;x\ne9\right)\\ B=\dfrac{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)+\sqrt{x}-8}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}\\ B=\dfrac{x-4+\sqrt{x}-8}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}=\dfrac{\left(\sqrt{x}-3\right)\left(\sqrt{x}-4\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}=\dfrac{\sqrt{x}-4}{\sqrt{x}-2}\)
\(c,B< A\Leftrightarrow\dfrac{\sqrt{x}-4}{\sqrt{x}-2}< \dfrac{\sqrt{x}+1}{\sqrt{x}-2}\Leftrightarrow\dfrac{\sqrt{x}-4}{\sqrt{x}-2}-\dfrac{\sqrt{x}+1}{\sqrt{x}-2}< 0\\ \Leftrightarrow\dfrac{-5}{\sqrt{x}-2}< 0\Leftrightarrow\sqrt{x}-2>0\left(-5< 0\right)\\ \Leftrightarrow x>4\\ d,P=\dfrac{B}{A}=\dfrac{\sqrt{x}-4}{\sqrt{x}-2}:\dfrac{\sqrt{x}+1}{\sqrt{x}-2}=\dfrac{\sqrt{x}-4}{\sqrt{x}+1}=1-\dfrac{5}{\sqrt{x}+1}\in Z\\ \Leftrightarrow5⋮\sqrt{x}+1\Leftrightarrow\sqrt{x}+1\inƯ\left(5\right)=\left\{-5;-1;1;5\right\}\\ \Leftrightarrow\sqrt{x}\in\left\{-6;-2;0;4\right\}\\ \Leftrightarrow x\in\left\{0;16\right\}\left(\sqrt{x}\ge0\right)\)
\(e,P=1-\dfrac{5}{\sqrt{x}+1}\)
Ta có \(\sqrt{x}+1\ge1,\forall x\Leftrightarrow\dfrac{5}{\sqrt{x}+1}\ge5\Leftrightarrow1-\dfrac{5}{\sqrt{x}+1}\le-4\)
\(P_{max}=-4\Leftrightarrow x=0\)

Bài 1:
3: ĐKXĐ: x>=1
\(x-\sqrt{x+3+4\sqrt{x-1}}=1\)
=>\(x-\sqrt{x-1+2\cdot\sqrt{x-1}\cdot2+4}=1\)
=>\(x-\sqrt{\left(\sqrt{x-1}+2\right)^2}=1\)
=>\(x-\left|\sqrt{x-1}+2\right|=1\)
=>\(x-\left(\sqrt{x-1}+2\right)=1\)
=>\(x-\sqrt{x-1}-2-1=0\)
=>\(x-1-\sqrt{x-1}-2=0\)
=>\(\left(\sqrt{x-1}\right)^2-2\sqrt{x-1}+\sqrt{x-1}-2=0\)
=>\(\left(\sqrt{x-1}-2\right)\left(\sqrt{x-1}+1\right)=0\)
=>\(\sqrt{x-1}-2=0\)
=>\(\sqrt{x-1}=2\)
=>x-1=4
=>x=5(nhận)

\(x^4+3x^2=0\)
Có \(x^4\ge0;\forall x\); \(3x^2\ge0;\forall x\)
=> VT\(\ge0;\forall x\)
Dấu = xảy ra <=> x=0
Ý C


a: Δ: 2x-y-1=0; A(-1;2)
B là ảnh của A qua phép đối xứng trục Δ
=>Δ là đường trung trực của AB
=>Δ vuông góc AB tại trung điểm H của AB
Đặt (d): ax+by+c=0 là phương trình đường thẳng AB
Δ: 2x-y-1=0
=>(d): x+y+c=0
Thay x=-1 và y=2 vào (d), ta được:
c-1+2=0
=>c+1=0
=>c=-1
=>(d): x+y-1=0
Tọa độ H là:
\(\left\{{}\begin{matrix}2x-y-1=0\\x+y-1=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2x-y=1\\x+y=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}3x=2\\x+y=1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=\dfrac{2}{3}\\y=1-\dfrac{2}{3}=\dfrac{1}{3}\end{matrix}\right.\)
H là trung điểm của AB
=>\(\left\{{}\begin{matrix}x_B+x_A=2\cdot x_H\\y_B+y_A=2\cdot y_H\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x_B-1=2\cdot\dfrac{2}{3}=\dfrac{4}{3}\\y_B+2=2\cdot\dfrac{1}{3}=\dfrac{2}{3}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x_B=\dfrac{4}{3}+1=\dfrac{7}{3}\\y_B=\dfrac{2}{3}-2=-\dfrac{4}{3}\end{matrix}\right.\)
Vậy: B(7/3;-4/3)
b: (C): \(\left(x-1\right)^2+\left(y-2\right)^2=9\); Δ: 2x-y-1=0
=>R=3 và tâm I(1;2)
Gọi D là điểm đối xứng của I qua phép đối xứng trục Δ, gọi E là giao điểm của DI với trục Δ, (d1): ax+by+c=0 là phương trình đường thẳng DI
D đối xứng I qua phép đối xứng trục Δ
=>Δ là đường trung trực của DI
=>Δ vuông góc (d1) tại trung điểm E của DI
Δ: 2x-y-1=0
=>(d1): x+y+c=0
Thay x=1 và y=2 vào (d1), ta được:
c+1+2=0
=>c+3=0
=>c=-3
=>(d1): x+y-3=0
Tọa độ E là:
\(\left\{{}\begin{matrix}2x-y-1=0\\x+y-3=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2x-y=1\\x+y=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}3x=4\\x+y=3\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=\dfrac{4}{3}\\y=3-\dfrac{4}{3}=\dfrac{5}{3}\end{matrix}\right.\)
E(4/3;5/3); I(1;2)
E là trung điểm của DI
=>\(\left\{{}\begin{matrix}x_D+x_I=2\cdot x_E\\y_D+y_I=2\cdot y_E\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x_D+1=2\cdot\dfrac{4}{3}=\dfrac{8}{3}\\y_D+2=2\cdot\dfrac{5}{3}=\dfrac{10}{3}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x_D=\dfrac{5}{3}\\y_D=\dfrac{4}{3}\end{matrix}\right.\)
Phương trình đường tròn (T) là:
\(\left(x-\dfrac{5}{3}\right)^2+\left(y-\dfrac{4}{3}\right)^2=9\)

a) \(A=\dfrac{\sqrt[]{x}+2}{\sqrt[]{x}-5}\) có nghĩa \(\Leftrightarrow\left\{{}\begin{matrix}x\ge0\\\sqrt[]{x}-5\ne0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge0\\\sqrt[]{x}\ne5\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x\ge0\\x\ne25\end{matrix}\right.\)
Khi \(x=16\Rightarrow A=\dfrac{\sqrt[]{16}+2}{\sqrt[]{16}-5}=\dfrac{4+2}{4-5}=-6\)
b) \(B=\dfrac{3}{\sqrt[]{x}+5}+\dfrac{20-2\sqrt[]{x}}{x-25}\)
B có nghĩa \(\Leftrightarrow\left\{{}\begin{matrix}x\ge0\\x-25\ne0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x\ge0\\x\ne25\end{matrix}\right.\)
\(\Leftrightarrow B=\dfrac{3\left(\sqrt[]{x}-5\right)+20-2\sqrt[]{x}}{\left(\sqrt[]{x}+5\right)\left(\sqrt[]{x}-5\right)}\)
\(\Leftrightarrow B=\dfrac{3\sqrt[]{x}-15+20-2\sqrt[]{x}}{\left(\sqrt[]{x}+5\right)\left(\sqrt[]{x}-5\right)}\)
\(\Leftrightarrow B=\dfrac{\sqrt[]{x}+5}{\left(\sqrt[]{x}+5\right)\left(\sqrt[]{x}-5\right)}\)
\(\Leftrightarrow B=\dfrac{1}{\sqrt[]{x}-5}\left(dpcm\right)\)
c) \(A=\dfrac{\sqrt[]{x}+2}{\sqrt[]{x}-5}\in Z\left(x\in Z\right)\)
\(\Leftrightarrow\sqrt[]{x}+2⋮\sqrt[]{x}-5\)
\(\Leftrightarrow\sqrt[]{x}+2-\left(\sqrt[]{x}-5\right)⋮\sqrt[]{x}-5\)
\(\Leftrightarrow\sqrt[]{x}+2-\sqrt[]{x}+5⋮\sqrt[]{x}-5\)
\(\Leftrightarrow7⋮\sqrt[]{x}-5\)
\(\Leftrightarrow\sqrt[]{x}-5\in U\left(7\right)=\left\{-1;1;-7;7\right\}\)
\(\Leftrightarrow x\in\left\{16;36;144\right\}\)
d) \(A>B\left(2\sqrt[]{x}+5\right)\)
\(\Leftrightarrow\dfrac{\sqrt[]{x}+2}{\sqrt[]{x}-5}>\dfrac{1}{\sqrt[]{x}-5}\left(2\sqrt[]{x}+5\right)\)
\(\Leftrightarrow\sqrt[]{x}+2>2\sqrt[]{x}+5\)
\(\Leftrightarrow\sqrt[]{x}< -3\)
mà \(\sqrt[]{x}\ge0\)
\(\Leftrightarrow x\in\varnothing\)


=>(50x+50x+250+65x+11050)*1,1=216500
=>165x+11300=196818,1818
=>165x=185518,1818
=>\(x\simeq124.353\)

c: ĐKXĐ: x<>8
\(\dfrac{3}{2x-16}+\dfrac{3x-20}{x-8}+\dfrac{1}{8}=\dfrac{13x-102}{3x-24}\)
=>\(\dfrac{9}{6\left(x-8\right)}+\dfrac{18x-120}{6\left(x-8\right)}-\dfrac{26x-204}{6\left(x-8\right)}=\dfrac{-1}{8}\)
=>\(\dfrac{18x-111-26x+204}{6\left(x-8\right)}=\dfrac{-1}{8}\)
=>\(\dfrac{-8x+93}{6x-48}=\dfrac{-1}{8}\)
=>\(\dfrac{8x-93}{6x-48}=\dfrac{1}{8}\)
=>8(8x-93)=6x-48
=>64x-744-6x+48=0
=>58x=696
=>x=12
d: ĐKXĐ: x<>1; x<>-1
\(\dfrac{6}{x^2-1}+5=\dfrac{8x-1}{4x+4}+\dfrac{12x-1}{4x-4}\)
=>\(\dfrac{24}{4\left(x-1\right)\left(x+1\right)}+\dfrac{20\left(x^2-1\right)}{4\left(x-1\right)\left(x+1\right)}=\dfrac{\left(8x-1\right)\left(x-1\right)+\left(12x-1\right)\left(x+1\right)}{4\left(x-1\right)\left(x+1\right)}\)
=>8x^2-9x+1+12x^2+12x-x-1=24+20x^2-20
=>20x^2+2x=20x^2+4
=>2x=4
=>x=2(loại)

a) Gọi x, y lần lượt là số mol Al, Fe
2Al + 3H2SO4 → Al2(SO4)3 + 3H2
Fe + H2SO4 → FeSO4+ H2
\(\left\{{}\begin{matrix}27x+56y=5,54\\\dfrac{3}{2}x+y=\dfrac{3,584}{22,4}\end{matrix}\right.\)
=> x=0,06 , y =0,07
=> \(m_{Al}=1,62\left(g\right);m_{Fe}=3,92\left(g\right)\)
b) \(n_{H_2SO_4\left(pứ\right)}=n_{H_2}=0,16\left(mol\right)\)
=> \(m_{H_2SO_4\left(pứ\right)}=0,16.98=15,68\left(g\right)\)
c) \(m_{ddH_2SO_4}=\dfrac{15,68}{20\%}=78,4\left(g\right)\)
c) 2NaOH + H2SO4 → Na2SO4 + 2H2O
\(n_{H_2SO_4\left(dư\right)}=\dfrac{1}{2}n_{NaOH}=\dfrac{1}{2}.0,25.0,6=0,075\left(mol\right)\)
=> \(m_{H_2SO_4\left(bđ\right)}=15,68+0,075.98=23,03\left(g\right)\)


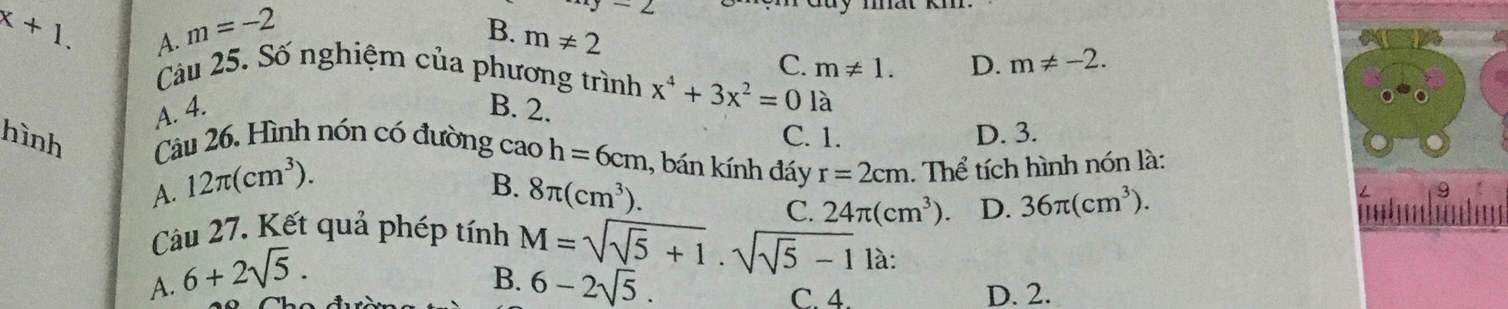

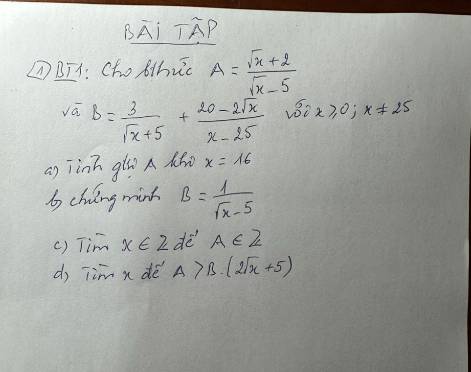

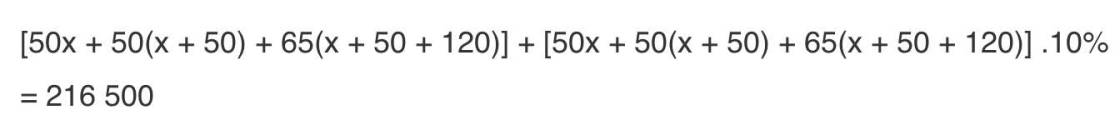
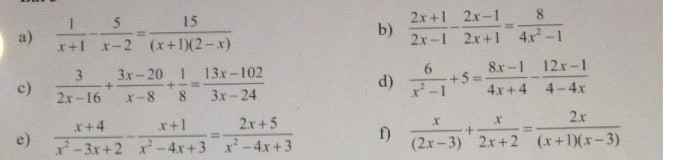

\(\dfrac{x-4}{x-1}+\dfrac{x+4}{x+1}=2\)
ĐKXĐ: \(x\ne\pm1\)
\(\Leftrightarrow\dfrac{\left(x-4\right)\left(x+1\right)}{\left(x-1\right)\left(x+1\right)}+\dfrac{\left(x+4\right)\left(x-1\right)}{\left(x-1\right)\left(x+1\right)}=\dfrac{2\left(x-1\right)\left(x+1\right)}{\left(x-1\right)\left(x+1\right)}\)
\(\Rightarrow x^2-3x-4+x^2+3x-4=2\left(x^2-1\right)\)
\(\Leftrightarrow2x^2-8-2x^2+2=0\)
\(\Leftrightarrow-6=0\) (Vô lí)
Vậy phương trình trên vô nghiệm.