Tìm x biết x3+6x2-x+30=0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
23 tháng 10 2021
\(\Leftrightarrow\left(x-2\right)^3=0\Leftrightarrow x-2=0\Leftrightarrow x=2\)
HV
27 tháng 8 2021
a,x(2x-1)-(x-1)^2-x^2=0
<=>x(2x-1-x)-(x-1)^2=0
<=>x(x-1)-(x-1)^2=0
<=>(x-x+1)(x-1)=0
<=>x-1=0
<=>x=1
b,(x+2)^3-x^3-6x^2=4
<=>x^3+6x^2+12x+8-x^3-6x^2=4
<=>12x+8=4
<=>x=-1/3
tick mik nha
27 tháng 8 2021
`a)x(2x-1)-(x-1)^2-x^2=0`
`<=>2x^2-x-x^2+2x-1-x^2=0`
`<=>x-1=0`
`<=>x=1`
Vậy `x=1.`
`b)(x+2)^3-x^3-6x^2=4`
`<=>x^3+6x^2+12x+8-x^3-6x^2=4`
`<=>12x+8=4`
`<=>12x=-4`
`<=>x=-1/3`
Vậy `x=-1/3.`
NL
1
8 tháng 10 2021
\(a,\Leftrightarrow2x^2-10x-2x^2-x=-11\\ \Leftrightarrow-11x=-11\Leftrightarrow x=1\\ b,\Leftrightarrow x\left(x^2-6x+9\right)=0\\ \Leftrightarrow x\left(x-3\right)^2=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x=3\end{matrix}\right.\\ c,\Leftrightarrow x\left(x-2018\right)-2017\left(x-2018\right)=0\\ \Leftrightarrow\left(x-2017\right)\left(x-2018\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=2017\\x=2018\end{matrix}\right.\)
C
1
C
2
8 tháng 8 2021
a: Ta có: \(\left(x-3\right)\left(x^2+3x+9\right)-x\left(x^2-3\right)=0\)
\(\Leftrightarrow x^3-27-x^3+3x=0\)
\(\Leftrightarrow x=9\)
b: Ta có: \(8x^4+x=0\)
\(\Leftrightarrow x\left(8x^3+1\right)=0\)
\(\Leftrightarrow x\left(2x+1\right)\left(4x^2-2x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-\dfrac{1}{2}\end{matrix}\right.\)
MN
1
26 tháng 2 2023
a: =>x^3+2x^2-8x^2-16x+15x+30=0
=>(x+2)(x^2-8x+15)=0
=>(x+2)(x-3)(x-5)=0
=>\(x\in\left\{-2;3;5\right\}\)
b: =x^2-12x+36-3
=(x-6)^2-3>=-3
Dấu = xảy ra khi x=6
3 tháng 9 2023
a: 49x^2-25=0
=>(7x-5)(7x+5)=0
=>7x-5=0 hoặc 7x+5=0
=>x=5/7 hoặc x=-5/7
b: Đề thiếu vế phải rồi bạn
c: (3x-2)^2-9(x+4)(x-4)=2
=>9x^2-12x+4-9(x^2-16)=2
=>9x^2-12x+4-9x^2+144=2
=>-12x+148=2
=>-12x=-146
=>x=146/12=73/6
d: x^3-6x^2+12x-8=0
=>(x-2)^3=0
=>x-2=0
=>x=2
e: x^3-9x^2+27x-27=0
=>(x-3)^3=0
=>x-3=0
=>x=3
H9
HT.Phong (9A5)
CTVHS
3 tháng 9 2023
a) \(-25+49x^2=0\)
\(\Leftrightarrow49x^2-25=0\)
\(\Leftrightarrow\left(7x\right)^2-5^2=0\)
\(\Leftrightarrow\left(7x-5\right)\left(7x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}7x-5=0\\7x+5=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}7x=5\\7x=-5\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{7}\\x=-\dfrac{5}{7}\end{matrix}\right.\)
b) \(16x^2-25\left(x-2\right)^2=0\)
\(\Leftrightarrow\left(4x\right)^2-\left[5\left(x-2\right)\right]^2=0\)
\(\Leftrightarrow\left(4x-5x+10\right)\left(4x+5x-10\right)=0\)
\(\Leftrightarrow\left(10-x\right)\left(9x-10\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}10-x=0\\9x=10\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=10\\x=\dfrac{10}{9}\end{matrix}\right.\)
c) \(\left(3x-2\right)^2-9\left(x+4\right)\left(x+4\right)=2\)
\(\Leftrightarrow9x^2-12x+4-9\left(x^2+8x+16\right)=2\)
\(\Leftrightarrow9x^2-12x+4-9x^2-72x-144=2\)
\(\Leftrightarrow-84x-140=2\)
\(\Leftrightarrow-84x=142\)
\(\Leftrightarrow x=-\dfrac{142}{84}\)
\(\Leftrightarrow x=-\dfrac{71}{42}\)
d) \(x^3-6x^2+12x-8=0\)
\(\Leftrightarrow x^3-3\cdot2\cdot x^2+3\cdot2^2\cdot x-2^3=0\)
\(\Leftrightarrow\left(x-2\right)^3=0\)
\(\Leftrightarrow x-2=0\)
\(\Leftrightarrow x=2\)
e) \(-27+27x-9x^2+x^3=0\)
\(\Leftrightarrow x^3-9x^2+27x-27=0\)
\(\Leftrightarrow\left(x-3\right)^3=0\)
\(\Leftrightarrow x-3=0\)
\(\Leftrightarrow x=3\)
26 tháng 9 2021
f: Ta có: \(x\left(2x-9\right)-4x+18=0\)
\(\Leftrightarrow\left(2x-9\right)\left(x-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{9}{2}\\x=2\end{matrix}\right.\)
g: Ta có: \(4x\left(x-1000\right)-x+1000=0\)
\(\Leftrightarrow\left(x-1000\right)\left(4x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1000\\x=\dfrac{1}{4}\end{matrix}\right.\)
HP
26 tháng 9 2021
f. x(2x - 9) - 4x + 18 = 0
<=> x(2x - 9) - 2(2x - 9) = 0
<=> (x - 2)(2x - 9) = 0
<=> \(\left[{}\begin{matrix}x-2=0\\2x-9=0\end{matrix}\right.\)
<=> \(\left[{}\begin{matrix}x=2\\x=\dfrac{9}{2}\end{matrix}\right.\)
g. 4x(x - 1000) - x + 1000 = 0
<=> 4x(x - 1000) - (x - 1000) = 0
<=> (4x - 1)(x - 1000) = 0
<=> \(\left[{}\begin{matrix}4x-1=0\\x-1000=0\end{matrix}\right.\)
<=> \(\left[{}\begin{matrix}x=\dfrac{1}{4}\\x=1000\end{matrix}\right.\)
h. 2x(x - 4) - 6x2(-x + 4) = 0
<=> 2x(x - 4) + 6x2(x - 4) = 0
<=> (2x + 6x2)(x - 4) = 0
<=> 2x(1 + 3x)(x - 4) = 0
<=> \(\left[{}\begin{matrix}2x=0\\1+3x=0\\x-4=0\end{matrix}\right.\)
<=> \(\left[{}\begin{matrix}x=0\\x=\dfrac{-1}{3}\\x=4\end{matrix}\right.\)
i. 2x(x - 3) + x2 - 9 = 0
<=> 2x(x - 3) + (x - 3)(x + 3) = 0
<=> (2x + x + 3)(x - 3) = 0
<=> (3x + 3)(x + 3) = 0
<=> \(\left[{}\begin{matrix}3x+3=0\\x+3=0\end{matrix}\right.\)
<=> \(\left[{}\begin{matrix}x=-1\\x=-3\end{matrix}\right.\)
j. 9x - 6x2 + x3 = 0
<=> x(9 - 6x + x2) = 0
<=> x(3 - x)2 = 0
<=> \(\left[{}\begin{matrix}x=0\\3-x=0\end{matrix}\right.\)
<=> \(\left[{}\begin{matrix}x=0\\x=3\end{matrix}\right.\)
KV
1 tháng 1
a) x² - 4 = 0
x² = 4
x = 2 hoặc x = -2
b) 2x(x + 5) - 3(5 + x) = 0
(x + 5)(2x - 3) = 0
X + 5 = 0 hoặc 2x - 3 = 0
*) x + 5 = 0
x = -5
*) 2x - 3 = 0
2x = 3
x = 3/2
c) x³ - 6x² + 11x - 6 = 0
x³ - x² - 5x² + 5x + 6x - 6 = 0
(x³ - x²) - (5x² - 5x) + (6x - 6) = 0
x²(x - 1) - 5x(x - 1) + 6(x - 1) = 0
(x - 1)(x² - 5x + 6) = 0
(x - 1)(x² - 2x - 3x + 6) = 0
(x - 1)[(x² - 2x) - (3x - 6)] = 0
(x - 1)[x(x - 2) - 3(x - 2)] = 0
(x - 1)(x - 2)(x - 3) = 0
x - 1 = 0 hoặc x - 2 = 0 hoặc x - 3 = 0
*) x - 1 = 0
x = 1
*) x - 2 = 0
x = 2
*) x - 3 = 0
x = 3
Vậy x = 1; x = 2; x = 3
6 tháng 7 2021
\(a,PT\Leftrightarrow x^3-6x^2+12x-8-x^3+x+6x^2-18x-10=0\)
\(\Leftrightarrow-5x-18=0\)
\(\Leftrightarrow x=-\dfrac{18}{5}\)
Vậy ...
\(b,PT\Leftrightarrow x^3+3x^2+3x+1-x^3+3x^2-3x+1-6x^2+12x-6+10=0\)
\(\Leftrightarrow12x+6=0\)
\(\Leftrightarrow x=-\dfrac{1}{2}\)
Vậy ...
\(c,PT\Leftrightarrow\left(x+1\right)^3+3^3=0\)
\(\Leftrightarrow\left(x+1+3\right)\left(x^2+2x+1-3x-3+9\right)=0\)
\(\Leftrightarrow\left(x+4\right)\left(x^2-x+7\right)=0\)
Thấy : \(x^2-\dfrac{2.x.1}{2}+\dfrac{1}{4}+\dfrac{27}{4}=\left(x-\dfrac{1}{2}\right)^2+\dfrac{27}{4}\ge\dfrac{27}{4}>0\)
\(\Rightarrow x+4=0\)
\(\Leftrightarrow x=-4\)
Vậy ...
\(d,PT\Leftrightarrow\left(x-2\right)^3+1^3=0\)
\(\Leftrightarrow\left(x-2+1\right)\left(x^2-4x+4-x+2+1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x^2-5x+7\right)=0\)
Thấy : \(x^2-5x+7=x^2-\dfrac{5.x.2}{2}+\dfrac{25}{4}+\dfrac{3}{4}=\left(x-\dfrac{5}{2}\right)^2+\dfrac{3}{4}\ge\dfrac{3}{4}>0\)
\(\Rightarrow x-1=0\)
\(\Leftrightarrow x=1\)
Vậy ...
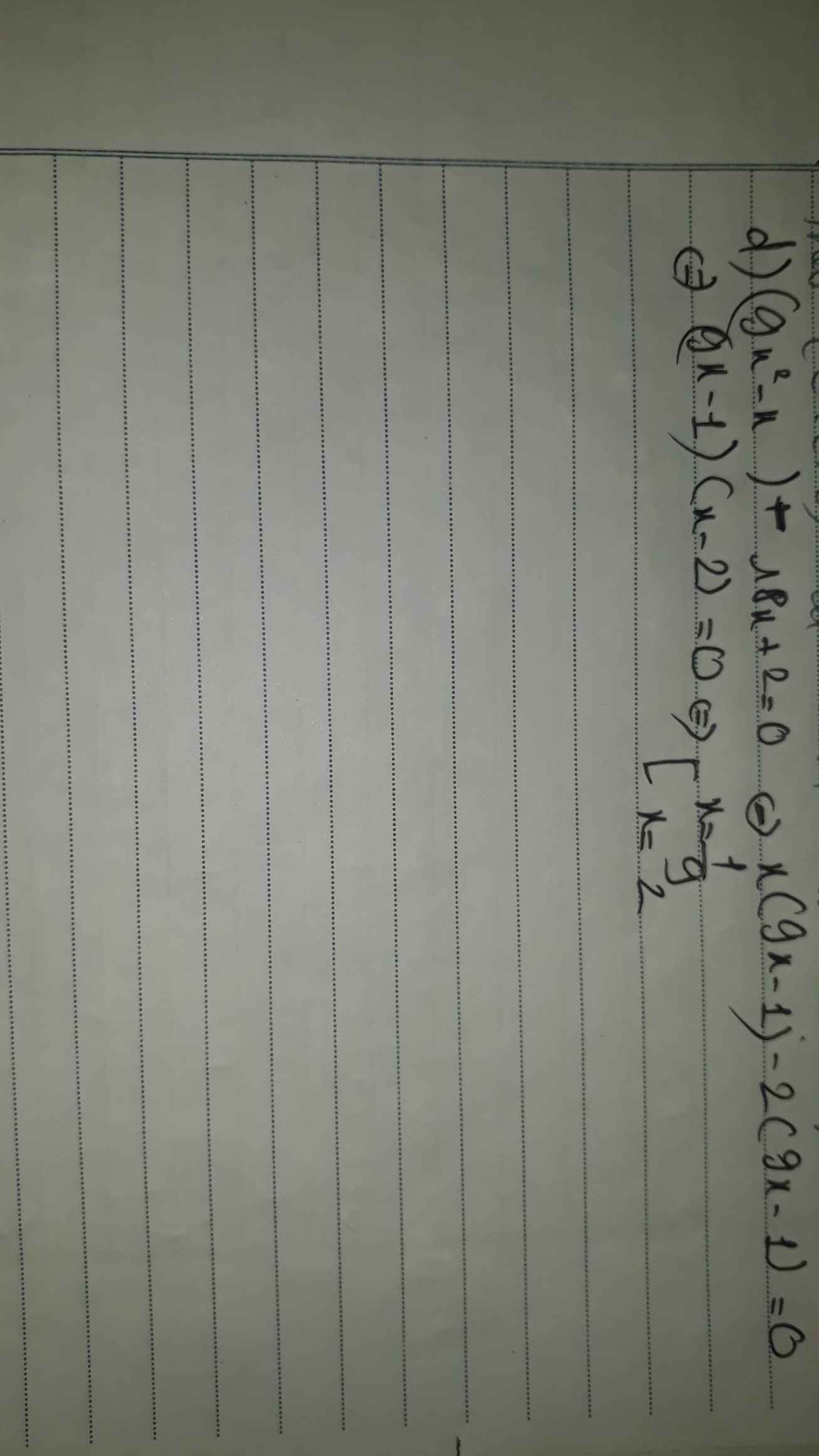
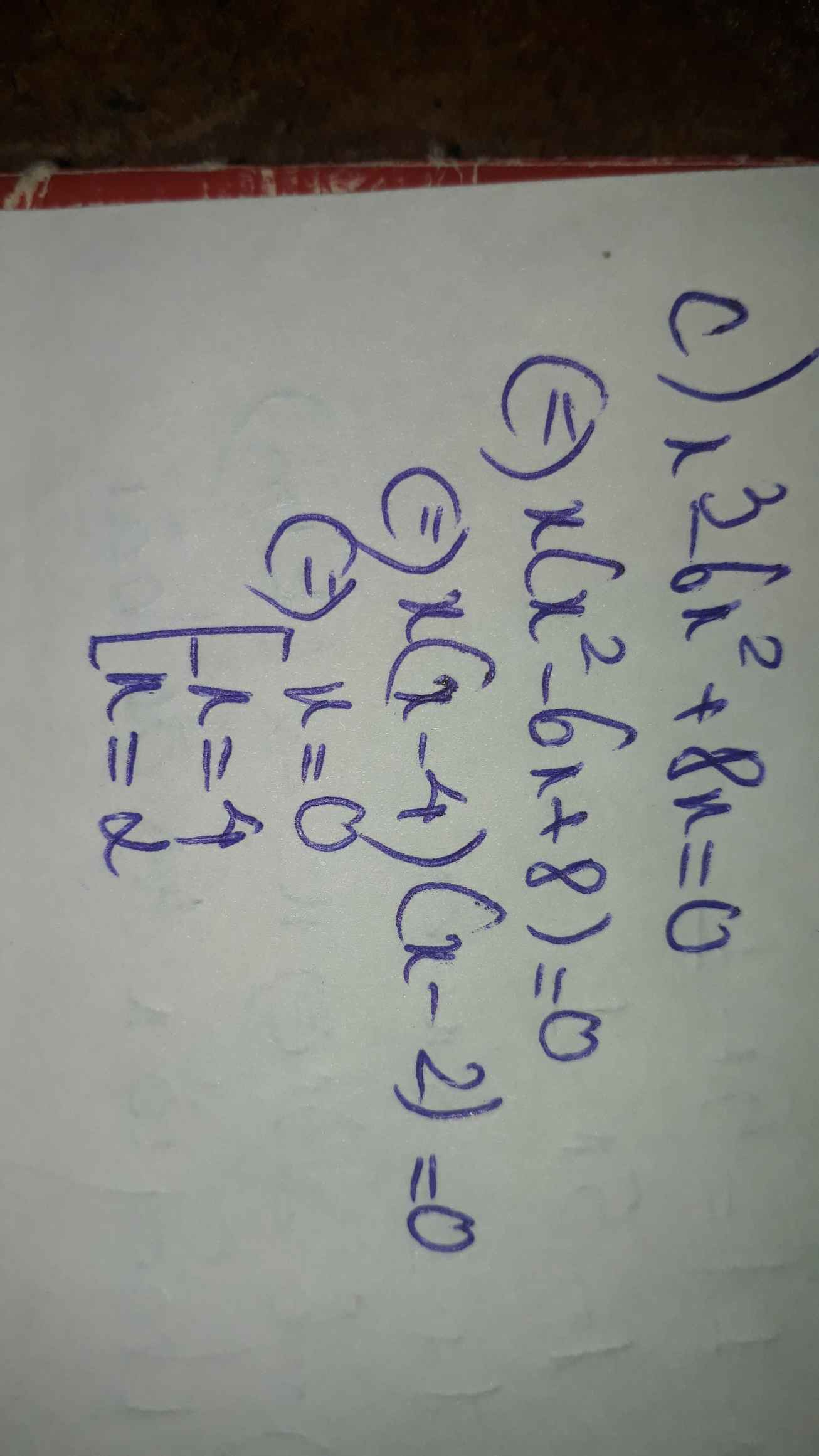
chiu
tk nhe
xin do
bye