Cho tan(a+b)=7 , tan(a-b)=4 . Khi đó , giá trị của tan2a là
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(tan2a=tan\left[\left(a+b\right)+\left(a-b\right)\right]=\dfrac{tan\left(a+b\right)+tan\left(a-b\right)}{1-tan\left(a+b\right)tan\left(a-b\right)}\)
\(\Rightarrow\dfrac{tan\left(a+b\right)+tan\left(a-b\right)}{1-tan\left(a+b\right)tan\left(a-b\right)}=\dfrac{5+4}{1-5.4}=-\dfrac{9}{19}\)
Vậy \(tan2a=-\dfrac{9}{19}\)

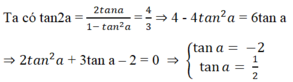
Vì π/2 < a < π nên tan a < 0, do đó tan a = -2.
Áp dụng công thức
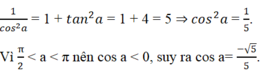
Đáp án là B.

Chọn B.
Theo công thức cộng ta có:
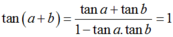
Mà a và b là các góc nhọn suy ra 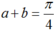

Theo giả thiết \(\tan A,\tan B,\tan C\) lập thành cấp số cộng thì ta có : \(\tan A+\tan C=2\tan B\)
\(\Leftrightarrow\tan A+\tan C=\frac{\sin\left(A+C\right)}{\cos A.\cos C}=\frac{\sin B}{\cos A.\cos C}\Rightarrow\frac{2\sin B}{\cos B}=\frac{\sin B}{\cos A.\cos C}\)
\(\Leftrightarrow\frac{2}{\cos B}=\frac{1}{\cos A.\cos C}\Leftrightarrow2\cos A.\cos C=\cos B\)
\(\Leftrightarrow\cos\left(A+C\right)+\cos\left(A-C\right)=\cos B\)
\(\Leftrightarrow-\cos B+\cos\left(A-C\right)=\cos B\Leftrightarrow\cos B=\frac{1}{2}\cos\left(A-C\right)\le\frac{1}{2}\left(2\right)\)
( Vì \(0 <\)\(\cos\left(A-C\right)\le1\) )
Do 0 < B \(\le\pi\Rightarrow\) giá trị nhỏ nhất của \(B=\frac{\pi}{3}\)

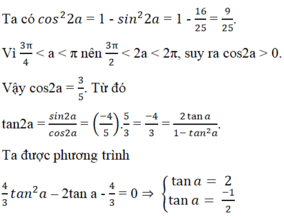
Vì 3π/4 < a < π nên tan a < 0. Vậy tan a = (-1)/2. Đáp án là D.

Bài 2:
\(n_{Na}=\dfrac{4,6}{23}=0,2\left(mol\right)\\a, 2Na+2H_2O\rightarrow2NaOH+H_2\\ b,n_{H_2}=\dfrac{0,2}{2}=0,1\left(mol\right)\\ V_{H_2\left(đktc\right)}=0,1.22,4=2,24\left(l\right)\\ c,NaOH:Tính.bazo\Rightarrow Quỳ.tím.hoá.xanh\)
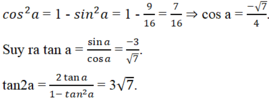

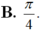
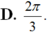

\(tan\left(a+b\right)=7;tan\left(a-b\right)=4\)
\(tan2a=tan\left[\left(a+b\right)+\left(a-b\right)\right]=\dfrac{7+4}{1-7.4}=\dfrac{11}{-27}=-\dfrac{11}{27}\)