Ét O Ét, giúp em bài 4 với ạ 🙏🙏🙏🙏🙏. Cần gấp
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
8 tháng 4 2022
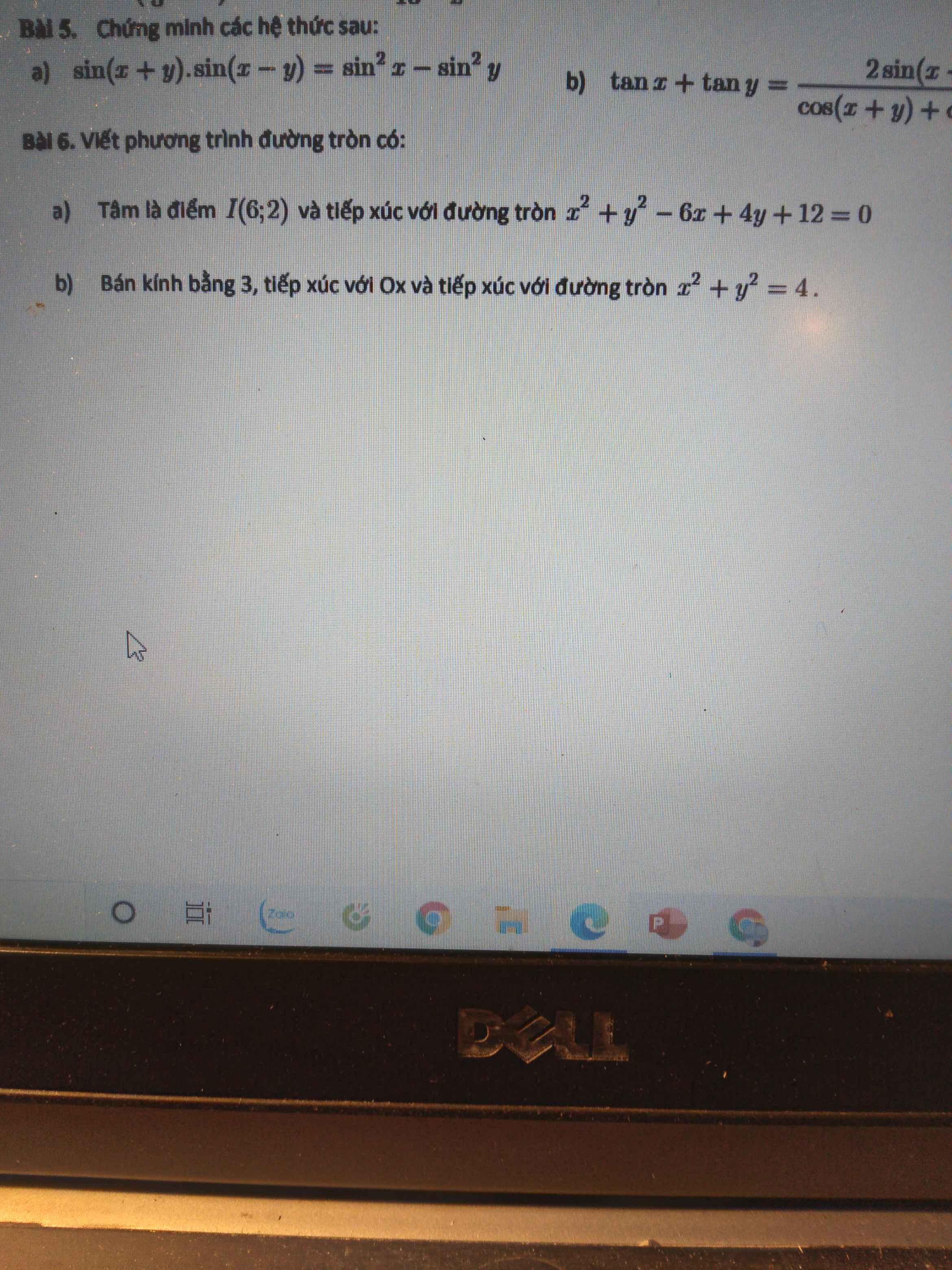
a.
Đường tròn (C): \(x^2+y^2-6x+4y+12=0\) có tâm \(J\left(3;-2\right)\) bán kính \(r=1\)
Tiếp điểm A của 2 đường tròn phải nằm trên đường nối tâm IJ
\(\overrightarrow{JI}=\left(3;4\right)\Rightarrow\) phương trình IJ có dạng:
\(4\left(x-3\right)-3\left(y+2\right)=0\Leftrightarrow4x-3y-18=0\)
Tọa độ tiếp điểm A là nghiệm của hệ :
\(\left\{{}\begin{matrix}4x-3y-18=0\\x^2+y^2-6x+4y+12=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}y=\dfrac{4x-18}{3}\\x^2+y^2-6x+4y+12=0\end{matrix}\right.\)
\(\Rightarrow x^2+\left(\dfrac{4x-18}{3}\right)^2-6x+4\left(\dfrac{4x-18}{3}\right)+12=0\)
\(\Rightarrow\dfrac{25}{9}x^2-\dfrac{50}{3}x+24=0\)
\(\Rightarrow\left[{}\begin{matrix}x=\dfrac{12}{5}\Rightarrow y=-\dfrac{14}{5}\\x=\dfrac{18}{5}\Rightarrow y=-\dfrac{6}{5}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}A\left(\dfrac{12}{5};-\dfrac{14}{5}\right)\\A\left(\dfrac{18}{5};-\dfrac{6}{5}\right)\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}\overrightarrow{AI}=\left(\dfrac{18}{5};\dfrac{24}{5}\right)\\\overrightarrow{AI}=\left(\dfrac{12}{5};\dfrac{16}{5}\right)\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}R^2=AI^2=36\\R^2=AI^2=\dfrac{36}{5}\end{matrix}\right.\)
Có 2 đường tròn thỏa mãn:
\(\left[{}\begin{matrix}\left(x-6\right)^2+\left(y-2\right)^2=36\\\left(x-6\right)^2+\left(y-2\right)^2=\dfrac{36}{5}\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
8 tháng 4 2022
b.
Đường tròn (C): \(x^2+y^2=4\) có tâm \(O\left(0;0\right)\) và bán kính \(r=2\)
Gọi \(I\left(a;b\right)\) là tâm của đường tròn (C') cần tìm
Do (C') tiếp xúc Ox \(\Rightarrow d\left(I;Ox\right)=3\Rightarrow\dfrac{\left|b\right|}{1}=3\Rightarrow b=\pm3\)
TH1: \(I\left(a;3\right)\Rightarrow\overrightarrow{OI}=\left(a;3\right)\Rightarrow OI=\sqrt{a^2+9}\)
Do 2 đường tròn tiếp xúc \(\Rightarrow\left[{}\begin{matrix}R+r=OI\\R-r=OI\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}OI=5\\OI=1\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}\sqrt{a^2+9}=5\\\sqrt{a^2+9}=1\left(vn\right)\end{matrix}\right.\)
\(\Rightarrow a=\pm4\)
TH2: hoàn toàn tương tự ta có tìm được \(a=\pm4\)
Vậy có 4 đường tròn thỏa mãn: \(\left[{}\begin{matrix}\left(x-4\right)^2+\left(y-3\right)^2=9\\\left(x+4\right)^2+\left(y-3\right)^2=9\\\left(x-4\right)^2+\left(y+3\right)^2=9\\\left(x+4\right)^2+\left(y+3\right)^2=9\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
10 tháng 4 2022
4b.
\(\dfrac{\pi}{2}< a< \pi\Rightarrow cosa< 0\Rightarrow cosa=-\sqrt{1-sin^2a}=-\dfrac{4}{5}\)
\(\Rightarrow tana=\dfrac{sina}{cosa}=-\dfrac{3}{4}\)
\(tan\left(a+\dfrac{\pi}{3}\right)=\dfrac{tana+tan\left(\dfrac{\pi}{3}\right)}{1-tana.tan\left(\dfrac{\pi}{3}\right)}=\dfrac{-\dfrac{3}{4}+\sqrt{3}}{1-\left(-\dfrac{3}{4}\right).\sqrt{3}}=...\)
c.
\(\dfrac{3\pi}{2}< a< 2\pi\Rightarrow cosa>0\Rightarrow cosa=\sqrt{1-sin^2a}=\dfrac{5}{13}\)
\(cos\left(\dfrac{\pi}{3}-a\right)=cos\left(\dfrac{\pi}{3}\right).cosa+sin\left(\dfrac{\pi}{3}\right).sina=\dfrac{1}{2}.\dfrac{5}{13}+\left(-\dfrac{12}{13}\right).\dfrac{\sqrt{3}}{2}=...\)
NN
1
S
10 tháng 2 2023
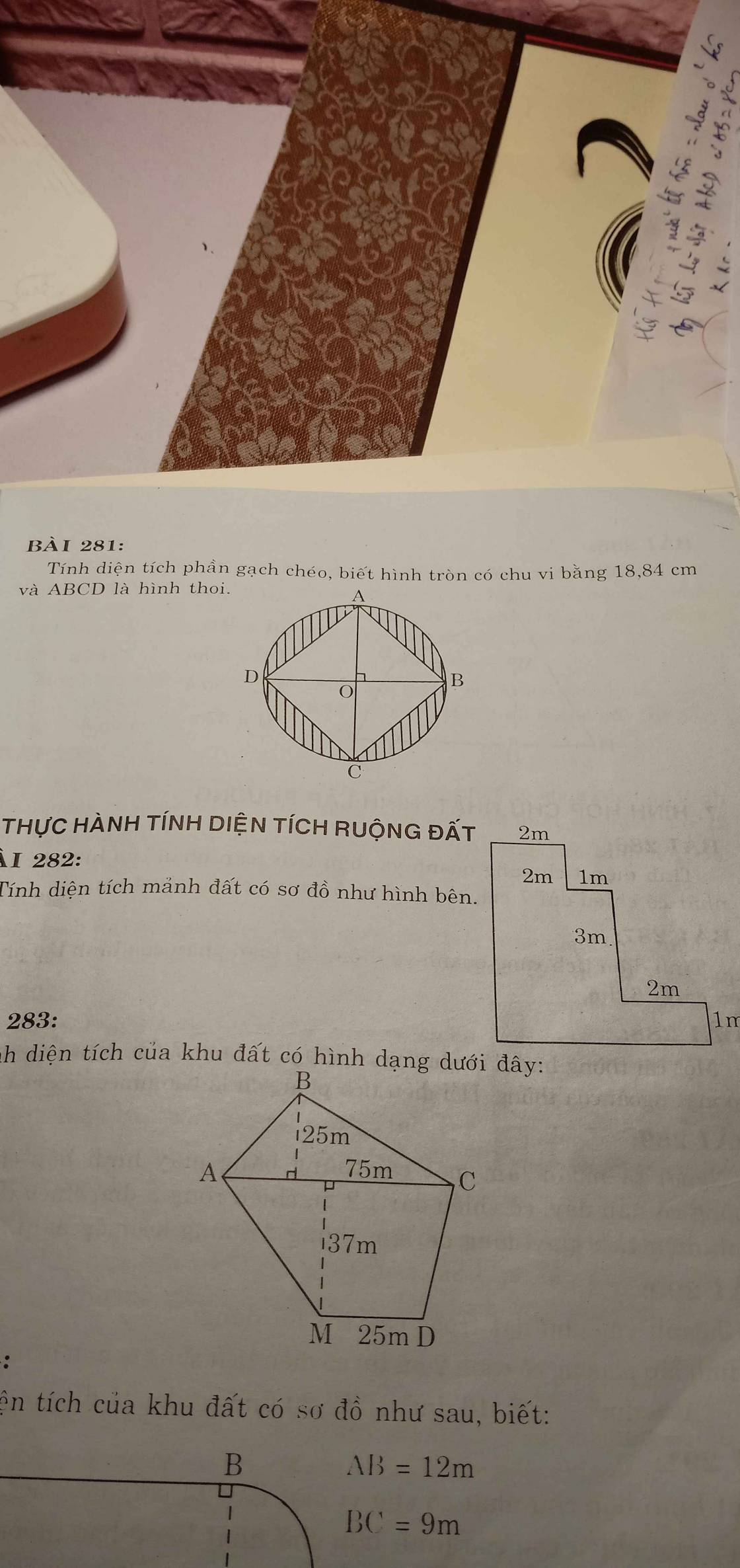
Bán kính hình tròn:
\(18,84:3,14:2=3\left(cm\right)\)
Diện tích hình tròn:
\(3\times3\times3,14=28,26\left(cm^2\right)\)
Đường kính hình tròn:
\(3\times2=6\left(cm\right)\)
Diện tích hình thoi:
\(\dfrac{6\times6}{2}=18\left(cm^2\right)\)
Diện tích phần gạch chéo:
\(28,26-18=10,26\left(cm^2\right)\)
16 tháng 6 2023
5:
a: sin x=2*cosx
\(A=\dfrac{6cosx+2cosx-4\cdot8\cdot cos^3x}{cos^3x-2cosx}\)
\(=\dfrac{8-32cos^2x}{cos^2x-2}\)
b: VT=sin^4(pi/2-x)+cos^4(x+pi/2)+6*1/2*sin^22x+1/2*cos4x
=cos^4x+sin^4x+3*sin^2(2x)+1/2*(1-2*sin^2(2x))
=1-2*sin^2x*cos^2x+3*sin^2(2x)+1/2-sin^2(2x)
==3/2=VP
NV
Nguyễn Việt Lâm
Giáo viên
21 tháng 12 2022
a.
Trong tam giác A'BC ta có: I là trung điểm BA', M là trung điểm BC
\(\Rightarrow IM\) là đường trung bình tam giác A'BC
\(\Rightarrow IM||A'C\)
\(\Rightarrow IM||\left(ACC'A'\right)\)
Do \(A\in\left(AB'M\right)\cap\left(ACC'A'\right)\) và \(\left\{{}\begin{matrix}IM\in\left(AB'M\right)\\A'C\in\left(ACC'A'\right)\\IM||A'C\end{matrix}\right.\)
\(\Rightarrow\) Giao tuyến của (AB'M) và (ACC'A') là đường thẳng qua A và song song A'C
Qua A kẻ đường thẳng d song song A'C
\(\Rightarrow d=\left(AB'M\right)\cap\left(ACC'A'\right)\)
b.
I là trung điểm AB', E là trung điểm AM
\(\Rightarrow IE\) là đường trung bình tam giác AB'M \(\Rightarrow IE||B'M\) (1)
Tương tự ta có IN là đường trung bình tam giác AA'B' \(\Rightarrow IN||A'B'\) (2)
(1);(2) \(\Rightarrow\left(EIN\right)||\left(A'B'M\right)\)
NV
Nguyễn Việt Lâm
Giáo viên
21 tháng 12 2022
c.
Trong mp (BCC'B'), qua K kẻ đường thẳng song song B'M lần lượt cắt BC và B'C' tại D và F
\(DF||B'M\Rightarrow DF||IE\Rightarrow DF\subset\left(EIK\right)\)
Trong mp (ABC), nối DE kéo dài cắt AB tại G
\(\Rightarrow G\in\left(EIK\right)\)
Trong mp (A'B'C'), qua F kẻ đường thẳng song song A'C' cắt A'B' tại H
Do IK là đường trung bình tam giác A'BC' \(\Rightarrow IK||A'B'\)
\(\Rightarrow FH||IK\Rightarrow H\in\left(EIK\right)\)
\(\Rightarrow\) Tứ giác DFHG là thiết diện (EIK) và lăng trụ
Gọi J là giao điểm BK và B'M \(\Rightarrow J\) là trọng tâm tam giác B'BC
\(\Rightarrow\dfrac{BJ}{BK}=\dfrac{2}{3}\)
Áp dụng talet: \(\dfrac{BM}{BD}=\dfrac{BJ}{BK}=\dfrac{2}{3}\Rightarrow BD=\dfrac{3}{2}BM=\dfrac{3}{2}.\dfrac{1}{2}BC=\dfrac{3}{4}BC\)
\(\Rightarrow MD=\dfrac{1}{4}BC=\dfrac{1}{2}CM\Rightarrow D\) là trung điểm CM
\(\Rightarrow DE\) là đường trung bình tam giác ACM
\(\Rightarrow DE||AC\Rightarrow DE||FH\)
\(\Rightarrow\) Thiết diện là hình thang
VT
1
4:
a: -90<a<0
=>cos a>0
cos^2a=1-(-4/5)^2=9/25
=>cosa=3/5
\(sin\left(45-a\right)=sin45\cdot cosa-cos45\cdot sina=\dfrac{\sqrt{2}}{2}\left(cosa-sina\right)\)
\(=\dfrac{\sqrt{2}}{2}\left(\dfrac{3}{5}-\dfrac{4}{5}\right)=\dfrac{-\sqrt{2}}{10}\)
b: pi/2<a<pi
=>cosa<0
cos^2a+sin^2a=0
=>cos^2a=16/25
=>cosa=-4/5
tan a=3/5:(-4/5)=-3/4
\(tan\left(a+\dfrac{pi}{3}\right)=\dfrac{tana+\dfrac{tanpi}{3}}{1-tana\cdot tan\left(\dfrac{pi}{3}\right)}\)
\(=\dfrac{-\dfrac{3}{4}+\sqrt{3}}{1-\dfrac{-3}{4}\cdot\sqrt{3}}=\dfrac{48-25\sqrt{3}}{11}\)
c: 3/2pi<a<pi
=>cosa>0
cos^2a+sin^2a=1
=>cos^2a=25/169
=>cosa=5/13
cos(pi/3-a)
\(=cos\left(\dfrac{pi}{3}\right)\cdot cosa+sin\left(\dfrac{pi}{3}\right)\cdot sina\)
\(=\dfrac{5}{13}\cdot\dfrac{1}{2}+\dfrac{-12}{13}\cdot\dfrac{\sqrt{3}}{2}=\dfrac{5-12\sqrt{3}}{26}\)