Tìm x,biết x4 + 6x3 - 60x + 100 = 11x2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
DN
1
4 tháng 9 2021
\(x^4+6x^3+11x^2+6x+1\)
\(=x^4+3x^3+x^2+3x^3+9x^2+3x+x^2+3x+1\)
\(=\left(x^2+3x+1\right)^2\)
DD
Đoàn Đức Hà
Giáo viên
17 tháng 5 2022
\(f\left(x\right)=x^4+6x^3+11x^2+6x=x\left(x+1\right)\left(x+2\right)\left(x+3\right)\)
\(x\) là số nguyên nên \(x\left(x+1\right)\left(x+2\right)\left(x+3\right)\) là tích của \(4\) số nguyên liên tiếp nên trong đó có nhất một số chia hết cho \(4\), một số chia hết cho \(3\), một số chia hết cho \(2\) nhưng không chia hết cho \(4\) nên \(f\left(x\right)\) chia hết cho \(2.3.4=24\).
Để \(f\left(x\right)\) chia hết cho \(5\) thì \(x,x+1,x+2,x+3\) có một số chia hết cho \(5\).
Có \(72=2.4.9\) nên để \(f\left(x\right)\) chia hết cho \(72\) thì trong \(4\) số \(x,x+1,x+2,x+3\) có một số chia hết cho \(9\) hoặc hai số chia hết cho \(3\), suy ra \(x\) chia hết cho \(3\).
C
1
L
14 tháng 8 2021
a) \(x^2-x+x=4\)
\(x^2=4\)
\(x=\pm2\)
b) \(3x\left(x-5\right)-2\left(x-5\right)=0\)
\(\left(x-5\right)\left(3x-2\right)=0\)
\(\left[{}\begin{matrix}x=5\\x=\dfrac{2}{3}\end{matrix}\right.\)
c) Ta có: \(a+b+c=5-3-2=0\)
\(\left[{}\begin{matrix}x=1\\x=\dfrac{c}{a}=\dfrac{-2}{5}\end{matrix}\right.\)
d) Đặt \(x^2=t\left(t\ge0\right)\) . Lúc đó phương trình trở thành :
\(t^2-11t+18=0\)
\(\left[{}\begin{matrix}t=9\left(tmđk\right)\\t=2\left(tmđk\right)\end{matrix}\right.\)
\(t=9\rightarrow x^2=9\rightarrow x=\pm3\)
\(t=2\rightarrow x^2=2\rightarrow x=\pm\sqrt{2}\)
15 tháng 10 2021
c: \(x^4+x^3-4x^2+x+1\)
\(=x^4-x^3+2x^3-2x^2-2x^2+2x-x+1\)
\(=\left(x-1\right)\left(x^3+2x^2-2x-1\right)\)
\(=\left(x-1\right)\left[\left(x-1\right)\left(x^2+x+1\right)+2x\left(x-1\right)\right]\)
\(=\left(x-1\right)^2\cdot\left(x^2+3x+1\right)\)
CM
31 tháng 3 2019
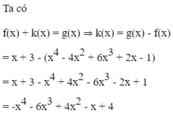
Nhận thấy số hạng có lũy thừa cao nhất của biến là - x 4 nên hệ số cao nhất là -1
Chọn đáp án A
C
2
14 tháng 8 2021
a:Ta có: \(x\left(x-1\right)+x=4\)
\(\Leftrightarrow x^2-x+x=4\)
\(\Leftrightarrow x^2=4\)
hay \(x\in\left\{2;-2\right\}\)
b: Ta có: \(3x\left(x-5\right)-2x+10=0\)
\(\Leftrightarrow\left(x-5\right)\left(3x-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=5\\x=\dfrac{2}{3}\end{matrix}\right.\)
c: Ta có: \(5x^2-3x-2=0\)
\(\Leftrightarrow5x^2-5x+2x-2=0\)
\(\Leftrightarrow\left(x-1\right)\left(5x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-\dfrac{2}{5}\end{matrix}\right.\)
d: Ta có: \(x^4-11x^2+18=0\)
\(\Leftrightarrow x^4-9x^2-2x^2+18=0\)
\(\Leftrightarrow x^2\left(x^2-9\right)-2\left(x^2-9\right)=0\)
\(\Leftrightarrow\left(x-3\right)\left(x+3\right)\left(x-\sqrt{2}\right)\left(x+\sqrt{2}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=3\\x=-3\\x=\sqrt{2}\\x=-\sqrt{2}\end{matrix}\right.\)
14 tháng 8 2021
a) x(x-1)+x=4
⇔x2=4⇔\(x=\pm2\)
b)3x(x-5)-2x+10=0
⇔3x(x-5)-2(x-5)=0
⇔(x-5)(3x-1)=0
\(\Leftrightarrow\left[{}\begin{matrix}x=5\\x=\dfrac{1}{3}\end{matrix}\right.\)
c)5x2-3x-2=0
⇔ 5x(x-1)+2(x-1)=0
⇔ (x-1)(5x+2)=0
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-\dfrac{2}{5}\end{matrix}\right.\)
d)x4-11x2+18=0
⇔ x2(x2-2)-9(x2-2)=0
⇔ (x2-2)(x2-9)=0
\(\Leftrightarrow\left[{}\begin{matrix}x^2=2\\x^2=9\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\pm\sqrt{2}\\x=\pm3\end{matrix}\right.\)
CM
26 tháng 6 2018
Đáp án A
Từ phương trình ta suy ra
x 4 − x 3 + 50 x 2 − 60 x + 20 = 13 x 3 − 11 x 2 + 22 x − 2 ⇔ x 4 − 14 x 3 + 61 x 2 − 82 x + 22 = 0 ⇔ x 4 − 8 x + 11 x 2 − 6 x + 2 = 0 ⇔ x = 3 − 7 x = 4 − 5 x = 3 + 7 x = 4 + 5
Ta đã biết phương trình đã cho có 4 nghiệm nên ta có
a = 3 − 7 ; c = 3 + 7 .
Do đó P = a 2 + c 2 = 32.
CM
17 tháng 5 2018
a) ( x 2 – 4x + 1)( x 2 – 2x + 3).
b) ( x 2 + 5x – 1)( x 2 + x – 1).
Ta có pt <=> (x-2)2 (x + 5)2
<=> x = 2 hoặc x = -5