5x-1+2.5x+5x+1=36
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
CM
13 tháng 4 2017
Chọn C.
Điều kiện: 5x – 1 > 0 hay x > 0
Phương trình đã cho tương đương:
log2( 5x - 1) [log2( 5x - 1) + 1] = 2
Đặt t = log2(5x - 1), khi đó phuơng trình trở thành: t(t + 1) = 2
Suy ra t = 1 hoặc t = -2
Với t =1 ta có log2(5x - 1) = 1 nên 5x – 1 = 2; x = log53
Với t = -2ta có log2(5x - 1) = - 2 nên 5x – 1 = 2-2; x = log5(5/4)
Mặt khác x1 > x2 suy ra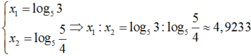 
GN
GV Nguyễn Trần Thành Đạt
Giáo viên
3 tháng 12 2023
\(X^2=49\\ Mà:7^2=49;\left(-7\right)^2=49\\ \Rightarrow X=7.hoặc.x=-7\\ ----\\ b,\left(5x+1\right)^2=121=11^2=\left(-11\right)^2\\ Nên:5x+1=11.hoặc.5x+1=-11\\ Nên:5x=10.hoặc.5x=-12\\ Vậy:x=2.hoặc.x=-\dfrac{12}{5}\\ ---\\ 3x+36=-7x-64\\ \Rightarrow3x+7x=-64-36\\ \Rightarrow10x=-100\\ \Rightarrow x=-\dfrac{100}{10}=-10\\ ---\\ -5x-1178=14x+145\\ \Rightarrow14x+5x=-1178-145\\ \Rightarrow19x=-1323\\ \Rightarrow x=\dfrac{-1323}{19}\)
21 tháng 8 2023
3) \(...\Rightarrow2^x\left(2^3+1\right)=36\)
\(\Rightarrow2^x.9=36\)
\(\Rightarrow2^x=4\)
\(\Rightarrow2^x=2^2\Rightarrow x=2\)
4) \(...\Rightarrow4^{x+1}-4^x=12\)
\(\Rightarrow4^x\left(4-1\right)=12\)
\(\Rightarrow4^x.3=12\)
\(\Rightarrow4^x=4=4^1\Rightarrow x=1\)
5) \(...\Rightarrow5^{x+1}\left(5^2-1\right)=3000\)
\(\Rightarrow5^{x+1}.24=3000\)
\(\Rightarrow5^{x+1}=125\)
\(\Rightarrow5^{x+1}=5^3\)
\(\Rightarrow x+1=3\)
\(\Rightarrow x=2\)
6) Bạn xem lại đề
21 tháng 8 2023
a. \(2^x.2^3+2^x=36\)
\(2^x\left(2^3+1\right)=36\)
\(2^x.9=36\)
\(2^x=4\Rightarrow x=2\)
b. \(4^x.4^1-\left(2^2\right)^x=12\)
\(4^x.4-4^x=12\)
\(4^x\left(4-1\right)=12\)
\(4^x.3=12\)
\(4^x=4\)
x = 1
c. \(5^x.5^3-5^x.5^1=3000\)
\(5^x\left(5^3-5^1\right)=3000\)
\(5^x.120=3000\)
\(5^x=25\)
x = 2
d. \(4^{x+1}=2^{2x}\)
\(4^x.4=\left(2^2\right)^x\)
\(4^x.4=4^x\)
Có vẻ như câu 4 này để bài thiếu
N
giải các Phương trình sau
a) (5x+3)(x2+1)(x-1)=0
b) (4x-1)(x-3)-(x-3)(5x+2)=0
c) (x+6)(3x-1)+x2-36 =0
1
23 tháng 2 2023
a: =>(5x+3)(x-1)=0
=>x=1 hoặc x=-3/5
b: =>(x-3)(4x-1-5x-2)=0
=>(x-3)(-x-3)=0
=>x=-3 hoặc x=3
c: =>(x+6)(3x-1+x-6)=0
=>(x+6)(4x-7)=0
=>x=7/4 hoặc x=-6
20 tháng 10 2021
\(\left(5x+1\right)^2=\dfrac{36}{25}\)
\(\Leftrightarrow\left[{}\begin{matrix}5x+1=\dfrac{6}{5}\\5x+1=-\dfrac{6}{5}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}5x=\dfrac{1}{5}\\5x=-\dfrac{11}{5}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{25}\\x=-\dfrac{11}{25}\end{matrix}\right.\)
QT
1
HQ
Hà Quang Minh
Giáo viên
19 tháng 9 2023
\(\begin{array}{l}a){\rm{ }}3{x^2}-{\rm{ }}3x\left( {x{\rm{ }}-{\rm{ }}2} \right){\rm{ }} = {\rm{ }}36\\ \Leftrightarrow 3{x^2}-{\rm{ [}}3x.x + 3x.( - 2)] = 36\\ \Leftrightarrow 3{x^2} - (3{x^2} - 6x) = 36\\ \Leftrightarrow 3{x^2} - 3{x^2} + 6x = 36\\ \Leftrightarrow 6x = 36\\ \Leftrightarrow x = 36:6\\ \Leftrightarrow x = 6\end{array}\)
Vậy x = 6
\(\begin{array}{l}b){\rm{ }}5x\left( {4{x^2}-{\rm{ }}2x{\rm{ }} + {\rm{ }}1} \right){\rm{ }}-{\rm{ }}2x\left( {10{x^2}-{\rm{ }}5x{\rm{ }} + {\rm{ }}2} \right){\rm{ }} = {\rm{ }} - 36\\ \Leftrightarrow 5x.4{x^2} + 5x.( - 2x) + 5x.1 - [2x.10{x^2} + 2x.( - 5x) + 2x.2] = - 36\\ \Leftrightarrow 20{x^3} - 10{x^2} + 5x - (20{x^3} - 10{x^2} + 4x) = - 36\\ \Leftrightarrow 20{x^3} - 10{x^2} + 5x - 20{x^3} + 10{x^2} - 4x = - 36\\ \Leftrightarrow (20{x^3} - 20{x^3}) + ( - 10{x^2} + 10{x^2}) + (5x - 4x) = - 36\\ \Leftrightarrow x = - 36\end{array}\)
Vậy x = -36