Mn ơi giúp e với ạ bài khó lắm luôn :((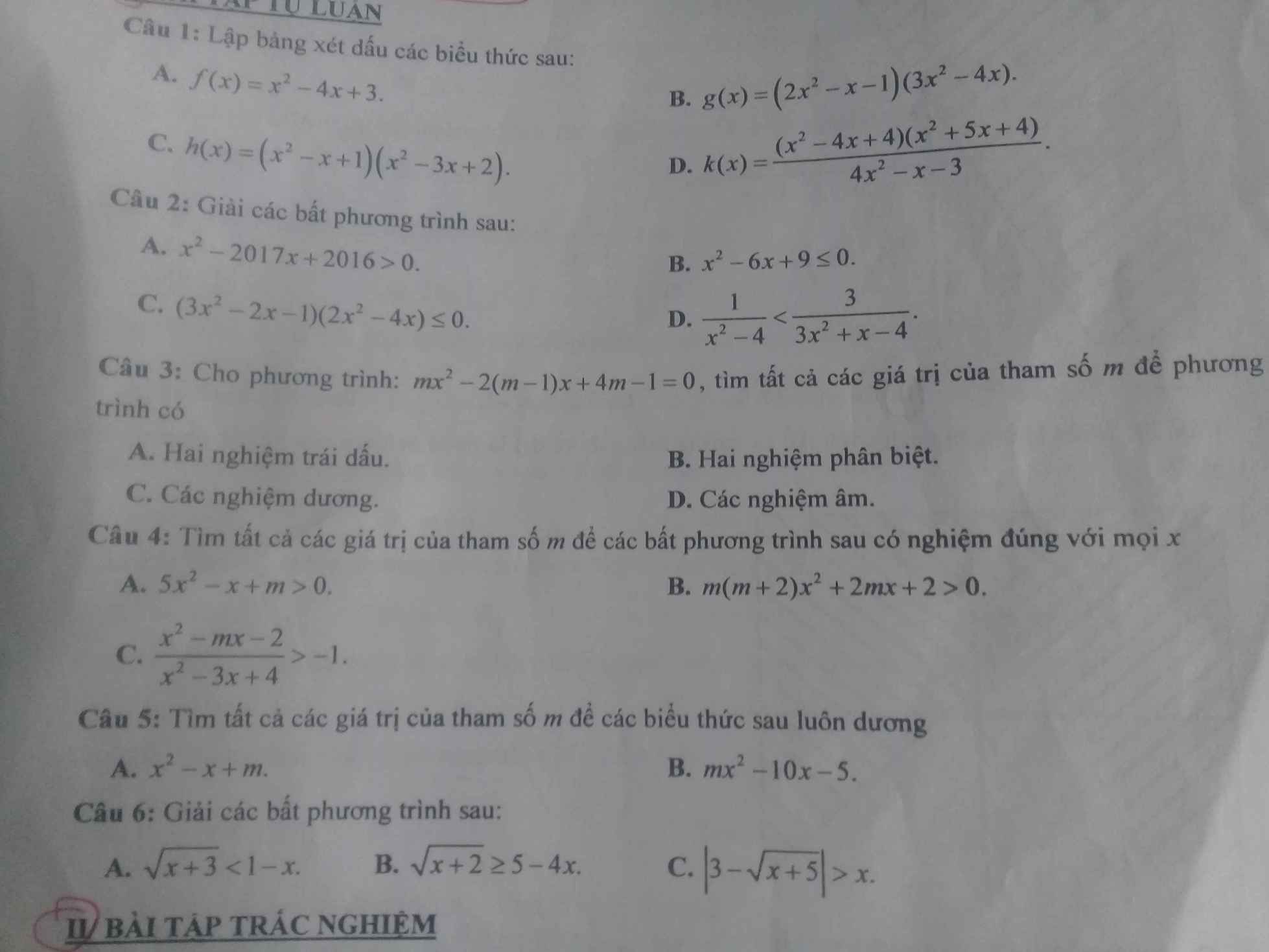
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(a,=x^2+x+4x+4=\left(x+1\right)\left(x+4\right)\\ b,=x^2+2x-3x-6=\left(x-3\right)\left(x+2\right)\\ c,=x^2-2x-3x+6=\left(x-2\right)\left(x-3\right)\\ d,=3\left(x^2-2x+5x-10\right)=3\left(x-2\right)\left(x+5\right)\\ e,=-3x^2+6x-x+2=\left(x-2\right)\left(1-3x\right)\\ f,=x^2-x-6x+6=\left(x-1\right)\left(x-6\right)\\ h,=4\left(x^2-3x-6x+18\right)=4\left(x-3\right)\left(x-6\right)\\ i,=3\left(3x^2-3x-8x+5\right)=3\left(x-1\right)\left(3x-8\right)\\ k,=-\left(2x^2+x+4x+2\right)=-\left(2x+1\right)\left(x+2\right)\\ l,=x^2-2xy-5xy+10y^2=\left(x-2y\right)\left(x-5y\right)\\ m,=x^2-xy-2xy+2y^2=\left(x-y\right)\left(x-2y\right)\\ n,=x^2+xy-3xy-3y^2=\left(x+y\right)\left(x-3y\right)\)

Bài 2: Chọn C
Bài 4:
a: \(\widehat{C}=180^0-80^0-50^0=50^0\)
Xét ΔABC có \(\widehat{A}=\widehat{C}< \widehat{B}\)
nên BC=AB<AC
b: Xét ΔABC có AB<BC<AC
nên \(\widehat{C}< \widehat{A}< \widehat{B}\)

ĐKXĐ: \(x\notin\left\{0;-9\right\}\)
Ta có: \(\dfrac{1}{x+9}-\dfrac{1}{x}=\dfrac{1}{5}+\dfrac{1}{4}\)
\(\Leftrightarrow\dfrac{20x}{20x\left(x+9\right)}-\dfrac{20\left(x+9\right)}{20x\left(x+9\right)}=\dfrac{4x\left(x+9\right)+5x\left(x+9\right)}{20x\left(x+9\right)}\)
Suy ra: \(4x^2+36x+5x^2+45x=20x-20x-180\)
\(\Leftrightarrow9x^2+81x+180=0\)
\(\Leftrightarrow x^2+9x+20=0\)
\(\Leftrightarrow x^2+4x+5x+20=0\)
\(\Leftrightarrow x\left(x+4\right)+5\left(x+4\right)=0\)
\(\Leftrightarrow\left(x+4\right)\left(x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+4=0\\x+5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-4\left(nhận\right)\\x=-5\left(nhận\right)\end{matrix}\right.\)
Vậy: S={-4;-5}

Hướng làm:
Thấy cả tử mẫu cộng lại đều bằng 2021 → Cộng thêm 1 rồi quy đồng với mỗi phân thức
\(\dfrac{x+2}{2019}+1+\dfrac{x+3}{2018}+1=\dfrac{x+4}{2017}+1+\dfrac{x}{2021}+1\\ \Leftrightarrow\dfrac{x+2021}{2019}+\dfrac{x+2021}{2018}-\dfrac{x+2021}{2017}-\dfrac{x+2021}{2021}=0\\ \Leftrightarrow\left(x+2021\right)\left(\dfrac{1}{2019}+\dfrac{1}{2018}-\dfrac{1}{2017}-\dfrac{1}{2021}\right)=0\\ \Leftrightarrow x+2021=0\Leftrightarrow x=-2021\)
\(< =>\dfrac{x+2}{2019}+1+\dfrac{x+3}{2018}+1=\dfrac{x+4}{2017}+1+\dfrac{x}{2021}+1\)
\(< =>\dfrac{x+2+2019}{2019}+\dfrac{x+3+2018}{2018}=\dfrac{x+4+2017}{2017}+\dfrac{x+2021}{2021}\)
\(< =>\dfrac{x+2021}{2019}+\dfrac{x+2021}{2018}-\dfrac{x+2021}{2017}-\dfrac{x+2021}{2021}=0\)
\(< =>\left(x+2021\right)\left(\dfrac{1}{2019}+\dfrac{1}{2018}-\dfrac{1}{2017}-\dfrac{1}{2021}=\right)=0\)
\(< =>x+2021=0< =>x=-2021\)
Vậy....

\(\frac{x+2}{2019}+\frac{x+3}{2018}=\frac{x+4}{2017}+\frac{x}{2021}\)
\(\Leftrightarrow\frac{x+2}{2019}+1+\frac{x+3}{2018}+1=\frac{x+4}{2017}+1+\frac{x}{2021}+1\)
\(\Leftrightarrow\frac{x+2021}{2019}+\frac{x+2021}{2018}=\frac{x+2021}{2017}+\frac{x+2021}{2021}\)
\(\Leftrightarrow x+2021=0\)
\(\Leftrightarrow x=-2021\)


a) \(n_{Zn}=\dfrac{13}{65}=0,2\left(mol\right);n_{H_2SO_4}=0,1.2,5=0,25\left(mol\right)\)
PTHH: Zn + H2SO4 → ZnSO4 + H2
Mol: 0,2 0,2 0,2 0,2
Ta có: \(\dfrac{0,2}{1}< \dfrac{0,25}{1}\) ⇒ Zn hết, H2SO4 dư
b) \(V_{H_2}=0,2.22,4=4,48\left(l\right)\)
c) \(m_{ZnSO_4}=0,2.161=32,2\left(g\right)\)
\(m_{H_2SO_4\left(dư\right)}=\left(0,25-0,2\right).98=4,9\left(g\right)\)
Bài 2 :
\(n_{Zn}=\dfrac{13}{65}=0,2\left(mol\right)\)
100ml = 0,1l
\(n_{H2SO4}=2,5.0,1=0,25\left(mol\right)\)
a) Pt : \(Zn+2H_2SO_4\rightarrow ZnSO_4+H_2|\)
1 1 1 1
0,2 0,25 0,2 0,2
b) Lập tỉ số so sánh : \(\dfrac{0,2}{1}< \dfrac{0,25}{2}\)
⇒ Zn phản ứng hết , H2SO4 dư
⇒ Tính toán dựa vào số mol của Zn
\(n_{H2}=\dfrac{0,2.1}{1}=0,2\left(mol\right)\)
\(V_{H2\left(dktc\right)}=0,2.22,4=4,48\left(l\right)\)
c) \(n_{ZnCl2}=\dfrac{0,2.1}{1}=0,2\left(mol\right)\)
⇒ \(m_{ZnCl2}=0,2.136=27,2\left(g\right)\)
\(n_{H2SO4\left(dư\right)}=0,25-0,2=0,05\left(mol\right)\)
⇒ \(m_{H2SO4\left(dư\right)}=0,05.98=4,9\left(g\right)\)
Chúc bạn học tốt

a, Vì D,M là trung điểm AB,AC nên DM là đtb tg ABC
Do đó \(DM=\dfrac{1}{2}BC=\dfrac{7}{2}\left(cm\right)\) và DM//BC
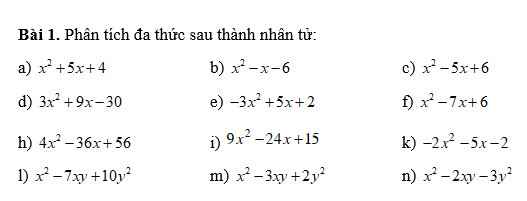



3.
TH1: \(m=0,pt\Leftrightarrow2x-1=0\Leftrightarrow x=\dfrac{1}{2}\)
TH2: \(m\ne0\)
a, Phương trình có hai nghiệm trái dấu khi \(m\left(4m-1\right)< 0\Leftrightarrow0< m< \dfrac{1}{4}\)
b, Phương trình có hai nghiệm phân biệt khi \(\Delta'=-3m^2-m+1>0\)
\(\Leftrightarrow\left[{}\begin{matrix}m< \dfrac{-1-\sqrt{13}}{6}\\m>\dfrac{-1+\sqrt{13}}{6}\end{matrix}\right.\)
c, Phương trình có hai nghiệm dương khi:
\(\left\{{}\begin{matrix}\Delta'>0\\x_1x_2>0\\x_1+x_2>0\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}m< -\dfrac{1+\sqrt{13}}{6}\\m>1\end{matrix}\right.\)