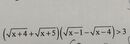
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


ĐK: \(x\ge0\)
Dễ thấy \(1-\sqrt{2\left(x^2-x+1\right)}\le1-\sqrt{2}< 0\)
Khi đó bất phương trình tương đương:
\(x-\sqrt{x}\le1-\sqrt{2\left(x^2-x+1\right)}\)
\(\Leftrightarrow\sqrt{x}-\dfrac{1}{\sqrt{x}}-1+\sqrt{2\left(x+\dfrac{1}{x}-1\right)}\le0\)
\(\Leftrightarrow\sqrt{x}-\dfrac{1}{\sqrt{x}}-1+\sqrt{2\left(\sqrt{x}-\dfrac{1}{\sqrt{x}}\right)^2+2}\le0\)
\(\Leftrightarrow t-1+\sqrt{2t^2+2}\le0\)

\(\dfrac{x^2+2}{x^2+4}=0\\ \Leftrightarrow x^2+2=0\)
Ta có: \(x^2\ge0;2>0\Rightarrow x^2+2>0\)
Vậy pt vô nghiệm

\(\dfrac{1}{a}-\dfrac{a-4}{4a}=6\)
\(ĐK:x\ne0\)
\(\Leftrightarrow\dfrac{4-\left(a-4\right)}{4a}=\dfrac{24a}{4a}\)
\(\Leftrightarrow4-\left(a-4\right)=24a\)
\(\Leftrightarrow4-a+4=24a\)
\(\Leftrightarrow8=25a\)
\(\Leftrightarrow a=\dfrac{8}{25}\left(tm\right)\)
Vậy \(S=\left\{\dfrac{8}{25}\right\}\)

Ta có:
(2 - 3x)(x + 8) = (3x - 2)(3 - 5x)
⇔ (2 - 3x)(x + 8) - (3x - 2)(3 - 5x) = 0
⇔ (2 - 3x)(x + 8) + (2 - 3x)(3 - 5x) = 0
⇔ (2 - 3x)(x + 8 + 3 - 5x) = 0
⇔ (2 - 3x)(11 - 4x) = 0
⇔ 2 - 3x = 0 hay 11 - 4x = 0
⇔ 2 = 3x hay 11 = 4x
⇔ x = \(\dfrac{2}{3}\) hay x = \(\dfrac{11}{4}\)
Vậy tập nghiệm của pt S = \(\left\{\dfrac{2}{3};\dfrac{11}{4}\right\}\)
<=> (2-3x ) (x+8) + (2-3x ) (3-5x)=0
<=> (2-3x ) ( x+8 + 3-5x ) =0
<=> (2-3x ) ( 11 - 4x ) = 0
=> 2-3x =0 hoặc 11-4x =0
3x = 2 4x =11
x = 2/3 x = 11/4

\(\left|4x-1\right|=5-x\)
\(\Leftrightarrow\left[{}\begin{matrix}4x-1=5-x\left(x\ge\dfrac{1}{4}\right)\\4x-1=x-5\left(x< \dfrac{1}{4}\right)\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{6}{5}\left(nhận\right)\\x=-\dfrac{4}{3}\left(nhận\right)\end{matrix}\right.\)

\(\Leftrightarrow2cos4x\left(cos2x-sin2x\right)=0\)
\(\Leftrightarrow cos4x=0\) (do \(cos4x=cos^22x-sin^22x\) đã bao hàm \(cos2x-sin2x\))
\(\Rightarrow4x=\dfrac{\pi}{2}+k\pi\)
\(\Rightarrow x=\dfrac{\pi}{8}+\dfrac{k\pi}{4}\)

\(\sqrt{x+3}-\sqrt{7-x}>\sqrt{2x-8}\)
⇔ \(\sqrt{x+3}>\sqrt{7-x}+\sqrt{2x-8}\)
⇔ \(\left\{{}\begin{matrix}4\le x\le8\\x+3>7-x+2x-8+2\sqrt{\left(7-x\right)\left(2x-8\right)}\end{matrix}\right.\)
⇔ \(\left\{{}\begin{matrix}4\le x\le8\\x+3>x-1+2\sqrt{\left(7-x\right)\left(2x+8\right)}\end{matrix}\right.\)
⇔ \(\left\{{}\begin{matrix}4\le x\le8\\4>2\sqrt{\left(7-x\right)\left(2x+8\right)}\end{matrix}\right.\)
⇔ \(\left\{{}\begin{matrix}4\le x\le8\\\sqrt{\left(7-x\right)\left(2x-8\right)}< 2\end{matrix}\right.\)
⇔ \(\left\{{}\begin{matrix}4\le x\le8\\-2x^2+22x-56< 2\end{matrix}\right.\)
⇔ \(\left\{{}\begin{matrix}4\le x\le8\\\left[{}\begin{matrix}x>\dfrac{11+\sqrt{5}}{2}\\x< \dfrac{11-\sqrt{5}}{2}\end{matrix}\right.\end{matrix}\right.\)
⇔ \(\left[{}\begin{matrix}4\le x< \dfrac{11-\sqrt{5}}{2}\\\dfrac{11+\sqrt{5}}{2}< x\le8\end{matrix}\right.\)
Các giá trị nguyên của x thỏa mãn là S = {4 ; 7 ; 8}
Ấy chết sai điều kiện XĐ rồi, bạn sửa lại điều kiện thôi nhé


\(D=[4;+\infty)\)
Bất phương trình tương đương:
\(\frac{\sqrt{x-4}-\sqrt{x-1}}{\sqrt{x+4}-\sqrt{x+5}}>3\)
\(\Leftrightarrow\sqrt{x-4}+3\sqrt{x+5}>3\sqrt{x+4}+\sqrt{x-1}\)
\(\Leftrightarrow10x+41+6\sqrt{x^2+x-20}>10x+35+6\sqrt{x^2+3x-4}\)(Vì VP và VT dương)
\(\Leftrightarrow1+\sqrt{x^2+x-20}>\sqrt{x^2+3x-4}\)
\(\Leftrightarrow1+2\sqrt{x^2+x-20}+x^2+x-20>x^2+3x-4\)(Vì \(VT>VP\ge0\))
\(\Leftrightarrow2\sqrt{x^2+x-20}>2x+15\)
\(\Leftrightarrow2x+15< 0\left(h\right)\hept{\begin{cases}2x+15\ge0\\4\left(x^2+x-20\right)>4x^2+60x+225\end{cases}}\)
\(\Leftrightarrow x< -\frac{15}{2}\left(h\right)\hept{\begin{cases}x\ge-\frac{15}{2}\\x< -\frac{305}{56}\end{cases}}\Leftrightarrow x< -\frac{305}{56}\)
Kết hợp TXĐ thì BPT vô nghiệm.