cho đa thức f(x)=x3 +(2m-n)x2-(m-3n)x+m.Tìm m và n để đa thức nhận m và n là nghiêm
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

- Để đa thức f(x) trên là một đa thức không thì :
\(\left\{{}\begin{matrix}2m-n+1=0\\m-3n+2=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2m-n=-1\\m-3n=-2\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}m=-\dfrac{1}{5}\\n=\dfrac{3}{5}\end{matrix}\right.\)
Vậy ...

a: \(M\left(x\right)=2x^2+3\)
\(N\left(x\right)=3x^3-2x^2+x\)
b: \(M\left(x\right)+N\left(x\right)=3x^3+x+3\)
\(M\left(x\right)-N\left(x\right)=2x^2+3-3x^3+2x^2-x=-3x^3+2x^2-x+3\)

Sửa đê: Q=mx^3+(m-2)x^2-(3n-5)x-4n
\(\dfrac{Q\left(x\right)}{x+1}\)
\(=\dfrac{mx^3+mx^2-2x^2-2x+\left(2-3n+5\right)x-4n}{x+1}\)
\(=mx^2-2x+\dfrac{\left(7-3n\right)x+7-3n-7-n}{x+1}\)
\(=mx^2-2x+7-3n+\dfrac{-n-7}{x+1}\)
Q(x) chia hết cho x+1
=>-n-7=0
=>n=-7
=>Q(x)=mx^3+(m-2)x^2+26x-28
\(\dfrac{Q\left(x\right)}{x-3}=\dfrac{mx^3-3mx^2+\left(4m-2\right)x^2-3\left(4m-2\right)x+\left(12m-6+26\right)x-28}{x-3}\)
\(=mx^2+\left(4m-2\right)x+\dfrac{\left(12m+20\right)x-28}{x-3}\)
\(=mx^2+\left(4m-2\right)x+\dfrac{\left(12m+20\right)x-3\left(12m+20\right)+3\left(12m+20\right)-28}{x-3}\)
\(=mx^2+\left(4m-2\right)x+12m+20+\dfrac{36m+32}{x-3}\)
Q(x) chia hết cho x-3
=>36m+32=0
=>m=-8/9

21:
a: \(f\left(x\right)=4x^4-x^3-4x^2+x-1\)
\(g\left(x\right)=x^4+4x^3+x-5\)
b: f(x)-g(x)
=4x^4-x^3-4x^2+x-1-x^4-4x^3-x+5
=3x^4-5x^3-4x^2+4
f(x)+g(x)
=4x^4-x^3-4x^2+x-1+x^4+4x^3+x-5
=5x^4+3x^3-4x^2+2x-6
c: g(-1)=1-4-1-5=-9

+ P(x) chia hết cho x + 1
⇔ P(-1) = 0
⇔ m . ( - 1 ) 3 + ( m – 2 ) ( - 1 ) 2 – ( 3 n – 5 ) . ( - 1 ) – 4 n = 0
⇔ -m + m – 2 + 3n – 5 – 4n = 0
⇔ -n – 7 = 0
⇔ n = -7 (1)
+ P(x) chia hết cho x – 3
⇔ P(3) = 0
⇔ m.33 + (m – 2).32 – (3n – 5).3 – 4n = 0
⇔ 27m + 9m – 18 – 9n + 15 – 4n = 0
⇔ 36m – 13n = 3 (2)
Từ (1) và (2) ta có hệ phương trình :
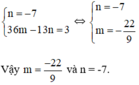

+ P(x) chia hết cho x + 1
⇔ P(-1) = 0
⇔ m.(-1)3 + (m – 2)(-1)2 – (3n – 5).(-1) – 4n = 0
⇔ -m + m – 2 + 3n – 5 – 4n = 0
⇔ -n – 7 = 0
⇔ n = -7 (1)
+ P(x) chia hết cho x – 3
⇔ P(3) = 0
⇔ m.33 + (m – 2).32 – (3n – 5).3 – 4n = 0
⇔ 27m + 9m – 18 – 9n + 15 – 4n = 0
⇔ 36m – 13n = 3 (2)
Từ (1) và (2) ta có hệ phương trình :
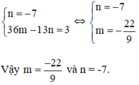

\(a,P\left(x\right)=2x^3-x+x^2-x^3+3x+5\\ =\left(2x^3-x^3\right)+x^2+\left(-x+3x\right)+5\\ =x^3+x^2+2x+5\\ Q\left(x\right)=3x^3+4x^2+3x-4x^3-5x^2+10\\ =\left(3x^3-4x^3\right)+\left(4x^2-5x^2\right)+3x+10\\ =-x^3-x^2+3x+10\\ b,M\left(x\right)=P\left(x\right)+Q\left(x\right)=x^3+x^2+2x+5-x^3-x^2+3x+10\\ =\left(x^3-x^3\right)+\left(x^2-x^2\right)+\left(2x+3x\right)+\left(5+10\right)=5x+15\\ N\left(x\right)=P\left(x\right)-Q\left(x\right)=x^3+x^2+2x+5-\left(-x^3-x^2+3x+10\right)\\ =x^3+x^2+2x+5+x^3+x^2-3x-10\\ =\left(x^3+x^3\right)+\left(x^2+x^2\right)+\left(2x-3x\right)+\left(5-10\right)\\ =2x^3+2x^2-x-5\)
`a,P(x)= 2x^3 -x+x^2 -x^3 +3x+5`
`= (2x^3 -x^3)+x^2+(-x+3x) +5`
`= x^3 +x^2 + 2x+5`
`Q(x)=3x^3 +4x^2+3x-4x^3-5x^2+10`
`= (3x^3-4x^3)+(4x^2-5x^2)+3x+10`
`= -x^3 -x^2+3x+10`
`b,M(x)=P(x)+Q(x)`
`->M(x)=(x^3 +x^2 + 2x+5)+(-x^3 -x^2+3x+10)`
`=x^3 +x^2 + 2x+5+(-x^3) -x^2+3x+10`
`=(x^3 -x^3)+(x^2 -x^2)+(2x+3x)+(5+10)`
`= 5x+15`
`N(x)=P(x)-Q(x)`
`->N(x)=(x^3 +x^2 + 2x+5)-(-x^3 -x^2+3x+10)`
`=x^3 +x^2 + 2x+5-x^3 +x^2-3x-10`
`=(x^3-x^3)+(x^2+x^2)+(2x-3x)+(5-10)`
`=2x^2 -x-5`

\(f\left(x\right)⋮\left(x+1\right)\)tức là chia hết cho \(\left[x-\left(-1\right)\right]\)
Do đó: \(f\left(-1\right)=0\Rightarrow n=-7\)
Tương tự, \(f\left(x\right)⋮\left(x-3\right)\)nên \(f\left(3\right)=0\)
\(\Rightarrow36m-13n-3=0\)
Giải hệ\(\hept{\begin{cases}n=-7\\36m-13n-3=0\end{cases}}\)
\(\Rightarrow\hept{\begin{cases}n=-7\\m=\frac{-22}{9}\end{cases}}\)

\(a,f\left(x\right):g\left(x\right)=\left(3x^4+9x^3+7x+2\right):\left(x+3\right)\\ =\left[3x^3\left(x+3\right)+7\left(x+3\right)-19\right]:\left(x+3\right)\\ =\left[\left(3x^3+7\right)\left(x+3\right)-19\right]:\left(x+3\right)\\ =3x^3+7.dư.19\)
\(c,\) Để \(k\left(x\right)⋮g\left(x\right)\Leftrightarrow-x^3-5x+2m=\left(x+3\right)\cdot a\left(x\right)\)
Thay \(x=-3\)
\(\Leftrightarrow-\left(-3\right)^3-5\left(-3\right)+2m=0\\ \Leftrightarrow27+15+2m=0\\ \Leftrightarrow2m=-42\\ \Leftrightarrow m=-21\)