Chứng minh phương trình : x2 - x + 3 vô nghiệm
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Điều kiện xác định x ≥ –8
Ta có: 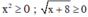 nên
nên 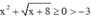 với mọi x ≥ –8.
với mọi x ≥ –8.
Do đó BPT 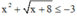 vô nghiệm.
vô nghiệm.

\(a,x^2-\left(2m-3\right)x+m^2=0-vô-ngo\)
\(\Leftrightarrow\Delta< 0\Leftrightarrow[-\left(2m-3\right)]^2-4m^2< 0\Leftrightarrow m>\dfrac{3}{4}\)
\(b,\left(m-1\right)x^2-2mx+m-2=0\)
\(m-1=0\Leftrightarrow m=1\Rightarrow-2x-1=0\Leftrightarrow x=-0,5\left(ktm\right)\)
\(m-1\ne0\Leftrightarrow m\ne1\Rightarrow\Delta'< 0\Leftrightarrow\left(-m\right)^2-\left(m-2\right)\left(m-1\right)< 0\Leftrightarrow m< \dfrac{2}{3}\)
\(c,\left(2-m\right)x^2-2\left(m+1\right)x+4-m=0\)
\(2-m=0\Leftrightarrow m=2\Rightarrow-6x+2=0\Leftrightarrow x=\dfrac{1}{3}\left(ktm\right)\)
\(2-m\ne0\Leftrightarrow m\ne2\Rightarrow\Delta'< 0\Leftrightarrow[-\left(m+1\right)]^2-\left(4-m\right)\left(2-m\right)< 0\Leftrightarrow m< \dfrac{7}{8}\)

Bài 2:
a: TH1: m=0
=>-x+1=0
=>x=-1(nhận)
TH2: m<>0
\(\text{Δ}=\left(m-1\right)^2-4m\left(1-m\right)\)
=m^2-2m+1-4m+4m^2
=5m^2-6m+1
=(2m-1)(3m-1)
Để phương trình có nghiệm thì (2m-1)(3m-1)>=0
=>m>=1/2 hoặc m<=1/3
b: Để phương trình có hai nghiệm phân biệt thì (2m-1)(3m-1)>0
=>m>1/2 hoặc m<1/3
c: Để phương trình có hai nghiệmtrái dấu thì (1-m)*m<0
=>m(m-1)>0
=>m>1 hoặc m<0
d: Để phương trình có hai nghiệm dương phân biệt thì
\(\left\{{}\begin{matrix}m\in\left(-\infty;\dfrac{1}{3}\right)\cup\left(\dfrac{1}{2};+\infty\right)\\\dfrac{-m+1}{m}>0\\\dfrac{1-m}{m}>0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m\in\left(-\infty;\dfrac{1}{3}\right)\cup\left(\dfrac{1}{2};+\infty\right)\\0< m< 1\end{matrix}\right.\)
=>1/2<m<1

x² + 2x + 3
= x² + 2x + 1 + 2
= (x + 1)² + 2 > 0 với mọi x
Vậy phương trình đã cho vô nghiệm

Chọn B và D
Phương trình B vô nghiệm vì \(5x^2+10\ge10>0\forall x\)
Phương trình C vô nghiệm vì \(x^2+6\ge6>-9\forall x\)
B và C
vì \(5x^2+10=0\Leftrightarrow5x^2=-10\Leftrightarrow x^2=-2\)(VL)
\(x^2+6=-9\Leftrightarrow x^2=-15\left(VL\right)\)

pt<=>x^2-2x.1/2+1/4-1/4+12/4=0
<=> (x-1/2)^2+11/4>=11/4>0
=>phương trình vô nghiệm
Ta có : x^2 - x +3 = 0
<=>x(x-1)=-3
Vì x(x-1) là 2 số tự nhiên liên tiếp nên chia hết cho 2
Mà 3 không chia hết cho 2
=> vậy phương trình trên vô nghiệm

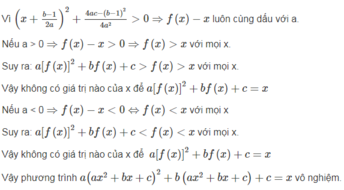
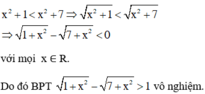
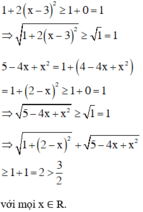
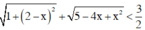 vô nghiệm.
vô nghiệm.
ta có: x2-x+3
= x2 - 1/2x - 1/2x + 1/4 + 11/4
= x(x - 1/2) - 1/2(x - 1/2) + 11/4
= (x - 1/2)*(x - 1/2) +11/4
= (x - 1/2)2 +11/4
vì (x - 1/2)2 >= 0 (với mọi x)
=> (x - 1/2)2 + 11/4 >= 11/4 (với mọi x)
hay đa thức x2 - x + 3 khác 0
vậy đa thức x2 - x + 3 vô nghiệm