4x3-4x2-x+1=0
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a, \(x^4-4x^3-6x^2-4x+1=0\)(*)
<=> \(x^4+4x^2+1-4x^3-4x+2x^2-12x^2=0\)
<=> \(\left(x^2-2x+1\right)^2=12x^2\)
<=>\(\left(x-1\right)^4=12x^2\) <=> \(\left[{}\begin{matrix}\left(x-1\right)^2=\sqrt{12}x\\\left(x-1\right)^2=-\sqrt{12}x\end{matrix}\right.\)<=> \(\left[{}\begin{matrix}x^2-2x+1-\sqrt{12}x=0\left(1\right)\\x^2-2x+1+\sqrt{12}x=0\left(2\right)\end{matrix}\right.\)
Giải (1) có: \(x^2-2x+1-\sqrt{12}x=0\)
<=> \(x^2-2x\left(1+\sqrt{3}\right)+\left(1+\sqrt{3}\right)^2-\left(1+\sqrt{3}\right)^2+1=0\)
<=> \(\left(x-1-\sqrt{3}\right)^2-3-2\sqrt{3}=0\)
<=> \(\left(x-1-\sqrt{3}\right)^2=3+2\sqrt{3}\) <=> \(\left[{}\begin{matrix}x-1-\sqrt{3}=\sqrt{3+2\sqrt{3}}\\x-1-\sqrt{3}=-\sqrt{3+2\sqrt{3}}\end{matrix}\right.\) <=> \(\left[{}\begin{matrix}x=\sqrt{3+2\sqrt{3}}+\sqrt{3}+1\left(ktm\right)\\x=-\sqrt{3+2\sqrt{3}}+\sqrt{3}+1\left(tm\right)\end{matrix}\right.\)
=> \(x=-\sqrt{3+2\sqrt{3}}+\sqrt{3}+1\)
Giải (2) có: \(x^2-2x+1+\sqrt{12}x=0\)
<=> \(x^2-2x\left(1-\sqrt{3}\right)+\left(1-\sqrt{3}\right)^2-\left(1-\sqrt{3}\right)^2+1=0\)
<=> \(\left(x+\sqrt{3}-1\right)^2=3-2\sqrt{3}\) .Có VP<0 => PT (2) vô nghiệm
Vậy pt (*) có nghiệm x=\(-\sqrt{3+2\sqrt{3}}+\sqrt{3}+1\)

Điều kiện: x 3 - 2 x 2 = x 2 x - 2 ≠ 0 ⇒ x ≠ 0 và x ≠ 2
Ta có: 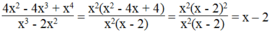
Nếu phân thức có giá trị bằng 0 thì biểu thức x - 2 cũng có giá trị bằng 0. Suy ra: x - 2 = 0 ⇒ x = 2 mà x = 2 không thỏa mãn điều kiện.
Vậy không có giá trị nào của x để phân thức có giá trị bằng 0.

c: \(x^4+x^3-4x^2+x+1\)
\(=x^4-x^3+2x^3-2x^2-2x^2+2x-x+1\)
\(=\left(x-1\right)\left(x^3+2x^2-2x-1\right)\)
\(=\left(x-1\right)\left[\left(x-1\right)\left(x^2+x+1\right)+2x\left(x-1\right)\right]\)
\(=\left(x-1\right)^2\cdot\left(x^2+3x+1\right)\)

$4x^3 + x = 4x^2$
$\Leftrightarrow x(4x^2 - 4x + 1) = 0$
$\Leftrightarrow x(2x - 1)^2 = 0$
$\Leftrightarrow \left[\begin{aligned}&x = 0\\ &2x - 1 = 0\\ \end{aligned}\right. \Leftrightarrow \left[\begin{aligned}&x = 0\\ &x = \dfrac12\\ \end{aligned}\right.$

1: \(A=5x^5-5x^3+7x^2-2x+4\)
\(B\left(x\right)=-5x^6+2x^4+4x^3+4x^2-4x-1\)
2: \(A\left(x\right)+B\left(x\right)=5x^5-5x^3+7x^2-2x+4-5x^6+2x^4+4x^3+4x^2-4x-1\)
\(=-5x^6+5x^5+2x^4-x^3+11x^2-6x+3\)
\(A\left(x\right)-B\left(x\right)\)
\(=5x^5-5x^3+7x^2-2x+4+5x^6-2x^4-4x^3-4x^2+4x+1\)
\(=5x^6+5x^5-2x^4-9x^3+3x^2+2x+5\)

a: Ta có: \(A=\left(2x+3\right)\left(4x^2-6x+9\right)-2\left(4x^3-1\right)\)
\(=8x^3+27-8x^3+2\)
=29
b: Ta có: \(B=\left(64x^3-1\right)-\left(4x-3\right)\left(16x^2+3\right)\)
\(=64x^3-1-64x^3-12x-48x^2+9\)
\(=-12x+8\)
c: Ta có: \(2\left(x^3+y^3\right)-3\left(x^2+y^2\right)\)
\(=2\left(x^2+xy+y^2\right)-3\left(-2xy\right)\)
\(=2x^2+2xy+2y^2+6xy\)
\(=2x^2+8xy+2y^2\)
\(\Leftrightarrow4x^2\left(x-1\right)-\left(x-1\right)=0\Leftrightarrow\left(2x-1\right)\left(2x+1\right)\left(x-1\right)=0\Leftrightarrow x=\dfrac{1}{2};x=-\dfrac{1}{2};x=1\)
\(4x^3-4x^2-x+1=0\)
<=>\(\left(2x+1\right)\left(x-1\right)\left(2x-1\right)=0\)
<=>\(\left[{}\begin{matrix}x=\dfrac{-1}{2}\\x=1\\x=\dfrac{1}{2}\end{matrix}\right.\)