A=3^2022-3^2021+3^2020-3^2019+...+3^2-3+1 với 1/4
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


B/A
\(=\dfrac{1+\dfrac{2020}{2}+1+\dfrac{2019}{3}+...+1+\dfrac{1}{2021}+1}{\dfrac{1}{2}+\dfrac{1}{3}+...+\dfrac{1}{2021}+\dfrac{1}{2022}}\)
\(=\dfrac{2022\left(\dfrac{1}{2}+\dfrac{1}{3}+...+\dfrac{1}{2021}+\dfrac{1}{2022}\right)}{\dfrac{1}{2}+\dfrac{1}{3}+...+\dfrac{1}{2021}+\dfrac{1}{2022}}=2022\)

a) Ta có:
2A=2.(12+122+123+...+122020+122021)2�=2.12+122+123+...+122 020+122 021
2A=1+12+122+123+...+122019+1220202�=1+12+122+123+...+122 019+122 020
Suy ra: 2A−A=(1+12+122+123+...+122019+122020)2�−�=1+12+122+123+...+122 019+122 020
−(12+122+123+...+122020+122021)−12+122+123+...+122 020+122 021
Do đó A=1−122021<1�=1−122021<1.
Lại có B=13+14+15+1360=20+15+12+1360=6060=1�=13+14+15+1360=20+15+12+1360=6060=1.
Vậy A < B.

\(B=\left(\dfrac{2020}{2}+1\right)+\left(\dfrac{2019}{3}+1\right)+...+\left(\dfrac{1}{2021}+1\right)+1\)
\(=\dfrac{2022}{2}+\dfrac{2022}{3}+...+\dfrac{2022}{2021}+\dfrac{2022}{2022}\)
=2022(1/2+1/3+...+1/2021+1/2022)
=>B/A=2022

\(\dfrac{x-4}{2022}+\dfrac{x-3}{2021}+\dfrac{x-2}{2020}+\dfrac{x-1}{2019}\text{=}-4\)
\(\dfrac{x-4}{2022}+\dfrac{x-3}{2021}+\dfrac{x-2}{2020}+\dfrac{x-1}{2019}+4\text{=}0\)
\(\left(\dfrac{x-4}{2022}+1\right)+\left(\dfrac{x-3}{2021}+1\right)+\left(\dfrac{x-2}{2020}+1\right)+\left(\dfrac{x-1}{2019}+1\right)\text{=}0\)
\(\dfrac{x-2018}{2022}+\dfrac{x-2018}{2021}+\dfrac{x-2018}{2020}+\dfrac{x-2018}{2019}\text{=}0\)
\(\left(x-2018\right)\left(\dfrac{1}{2022}+\dfrac{1}{2021}+\dfrac{1}{2020}+\dfrac{1}{2019}\right)\text{=}0\)
\(Do:\) \(\dfrac{1}{2022}+\dfrac{1}{2021}+\dfrac{1}{2020}+\dfrac{1}{2019}\ne0\)
\(x-2018\text{=}0\)
\(x\text{=}2018\)
\(Vậy...\)

=1+(2-3-4+5)+(6-7-8+9)+.....+(2018-2019-2020+2021)+2022
=1+0+0+.....+0+2022
=2023
số năm nay luôn

Ta có: 1+2-3-4+5+6-7-8+.....-2019-2020+2021+2022
=1+(2-3-4+5)+(6-7-8+9)+.....+(2018-2019-2020+2021)+2022
=1+0+0+.....+0+2022
=2023
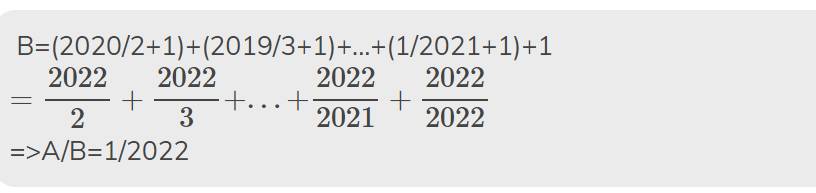
\(A=\left(3^{2022}-3^{2021}\right)+\left(3^{2020}-3^{2019}\right)+...+\left(3^2-3\right)+1\)
\(3^{2022}>3^{2021}\Rightarrow3^{2022}-3^{2021}>0\)
\(3^{2020}>3^{2019}\Rightarrow3^{2020}-3^{2019}>0\)
.....
\(3^2>3\Rightarrow3^2-3>0\)
\(\Rightarrow A>0+0+0+....+0+1=1>\frac{1}{4}\)
\(\Rightarrow A>\frac{1}{4}\)